Punjab State Board PSEB 12th Class English Book Solutions Supplementary Chapter 1 The School for Sympathy Textbook Exercise Questions and Answers.
Class 12th English Supplementary Chapter 1 The School for Sympathy Question Answers
The School for Sympathy Class 12 Questions and Answers
Short Answer Type Questions
Question 1.
Give a brief account of Mr. Lucas’s visit to Miss Beam’s school.
Mr: Lucas के Miss Beam के स्कूल में दौरे का संक्षिप्त वर्णन करो।
Answer:
Once the author visited Miss Beam’s school. It taught normal school subjects and also made the students sympathetic, thoughtful and kind. The author saw many handicapped children. Actually they were all healthy. They were playing at being crippled. Each child was made to have one blind day, one lame day, one dumb day and one maimed day in a term.
This made the students understand the misfortunes of the handicapped. The blind day was very troublesome. At the end of the visit, the author thought that Miss Beam’s school did a very useful service in making the students sympathetic and kind.
![]()
एक बार लेखक मिस बीम के स्कूल गया। इसमें आम विषय पढ़ाए जाते थे और यह विद्यार्थियों को सहानुभूतिपूर्ण, विचारशील और दयालु बनाता था। लेखक ने बहुत से अपंग बच्चे देखे। वास्तव में वे सभी स्वस्थ थे। वे अपंग होने का अभिनय कर रहे थे।
एक अवधि में हर बच्चे के लिए एक अन्धा होने का दिन, एक लंगड़ा होने का दिन, एक बहरा और गूंगा होने का दिन और एक अपाहिज होने का दिन आवश्यक था। इससे विद्यार्थियों को अपंग मानवों के दुर्भाग्य की जानकारी होती थी। अन्धा होने का दिन बहुत कष्टदायक था। दौरे के अन्त में लेखक ने सोचा कि मिस बीम का स्कूल विद्यार्थियों को सहानुभूतिपूर्ण तथा दयालु बनने में बहुत लाभदायक काम करता था।
Question 2.
“In the course of the term every child has one blind day, one lame day, one deaf day, one maimed day, one dumb day.” What were the children expected to do on these days ? ”
(पढ़ाई की) अवधि के दौरान प्रत्येक बच्चे को एक दिन अन्धा, एक दिन लंगड़ा, एक दिन बहरा, एक दिन अपंग, एक दिन गूंगा होना पड़ता है।” इन दिनों बच्चों से क्या आशा की जाती थी ?
Answer:
On the blind day, the eyes of children were bandaged. Such children needed help in everything. On the lame day, a leg of the child was tied up and he was to hop about on a crutch. On the deaf day, the ears of children were clogged. On the maimed day, an arm was tied up and the children had to get their food cut for them.
On the dumb day, they were to remain silent. As their mouths were not bandaged, they had to depend upon their will power. They were made to take part in these misfortunes in order to make them appreciate and understand the misfortune of others. The basic idea was to make the children sympathetic towards such helpless children.
![]()
अन्धा होने के दिन, बच्चों की आंखों पर पट्टी बांध दी जाती थी। ऐसे बच्चों को प्रत्येक काम में सहायता की आवश्यकता थी। लंगड़ा होने के दिन बच्चे की एक टांग बांध दी जाती थी और उसे बैसाखी पर फुदकना पड़ता था। बहरा होने के दिन, बच्चों के कान अवरुद्ध कर दिये जाते थे।
अपंग होने के दिन बच्चे की एक भुजा बांध दी जाती थी और बच्चों को उनका भोजन काटना होता था। गूंगा होने के दिन उन्हें चुप रहना होता था। क्योंकि उनके मुंह पर पट्टी नहीं बांधी जाती थी उन्हें अपनी इच्छा-शक्ति पर निर्भर रहना पड़ता था। उन्हें इन दुर्भाग्यों में भाग लेने के लिये शिक्षित किया जाता था ताकि वे दूसरों के दुर्भाग्य को समझ सकें। मुख्य विचार बच्चों को ऐसे असहाय बच्चों के प्रति सहानुभूतिपूर्ण बनाना था।
Long Answer Type Questions
Question 1.
What did the author see in Miss Beam’s school at first sight? How did he feel about it?
पहली नज़र में लेखक ने Miss Beam के स्कूल में क्या देखा ? इस के बारे में उसे कैसा लगा ?
Answer:
The author visited Miss Beam’s school. He looked out of the window. He told Miss Beam that he had seen some very beautiful grounds and a lot of jolly children. But it was an unpleasant and painful experience. He pointed out that all the children were not as healthy and active as they should be.
On entering the school, he saw a girl being led about by another child. It could be understood that the girl had some trouble with her eyes. After that, the writer could see two more girls in the same condition. He also saw a girl with a crutch watching the other children at play. He came to the conclusion that the girl must be a helpless cripple.
लेखक मिस बीम के स्कूल गया। उसने खिड़की से बाहर देखा। उसने मिस बीम को बताया कि उसने बहुत सुन्दर स्थल और बहुत से प्रसन्न बच्चे देखे हैं। लेकिन यह असुहावना और दुखद अनुभव था। उसने कहा कि सब बच्चे इतने स्वस्थ और चुस्त नहीं थे जितने होने चाहिये। स्कूल में प्रवेश करने पर उसने एक लड़की को दूसरे बच्चे द्वारा ले जाते हुए देखा।
यह समझा जा सकता था कि लड़की की आंखों मे कोई तकलीफ थी। इसके पश्चात् लेखक दो और लड़कियों को उसी हालत में देख सकता था। उसने एक लड़की को बैसाखी के साथ दूसरे बच्चों को खेलते हुए देखा। वह इस निष्कर्ष पर पहुंचा कि लड़की असहाय विकलांग थी।
Question 2.
Give a character-sketch of Miss Beam.
Answer:
Miss Beam was kind-hearted, middle-aged, authoritative and full of understanding. She started a new school known as the School for Sympathy. Important school subjects were taught in this school. But this school was different in one aspect. Here the students were given training in good qualities. The real aim of the school was to give training in thoughtfulness, humanity and good citizenship.
Every child in her school had one blind day, one lame day, one deaf day and one dumb day etc. The children thus had a taste of misfortune. As a result, they learnt to be sympathetic towards handicapped people. Miss Beam was an asset to society. She wanted to promote noble ideas in society.
![]()
मिस बीम एक दयालु-हृदय वाली, अधेड़ अवस्था की, रौबदार और समझदार स्त्री थी। उसने School for Sympathy के नाम से एक नया स्कूल चालू किया। स्कूल के महत्त्वपूर्ण विषय इस स्कूल में पढ़ाये जाते थे। लेकिन एक बात में यह स्कूल भिन्न था। यहां विद्यार्थियों को अच्छे गुणों की शिक्षा दी जाती थी। स्कूल का मुख्य उद्देश्य विचारशीलता, मानवता और नागरिकता में प्रशिक्षण देना था।
इसके स्कूल के प्रत्येक बच्चे का एक अन्धा होने का दिन, एक लंगड़ा होने का दिन, एक बहरा होने का दिन और एक गूंगा होने का दिन होता था। इस तरह बच्चे दुर्भाग्य का अनुमान लगा सकते थे। परिणामस्वरूप, उन्होंने अपंग लोगों के प्रति सहानुभूतिशील होना सीख लिया। मिस बीम समाज के लिए एक पूंजी थी। वह समाज में अच्छे विचारों का विकास करना चाहती थी।
Question 3.
Give in your own words the theme of the lesson ‘The School For Sympathy’.
Answer:
Traditional or conventional education given in schools is not ideal. It gives information of facts. It enables a person to earn his living. In addition to the normal subjects, the students of Miss Beam’s ideal school were also given lessons on humanity and citizenship.
Here students got a real understanding of misfortune. During training every child had one blind day, one deaf day and one dumb day. During the blind day their eyes were bandaged. The bandage was also put during the night. By being blind for a day the child realised what a misfortune it was to be blind. In the same way children learnt the difficulties of the deaf and the dumb people.
स्कलों में दी जाने वाली परम्परागत शिक्षा आदर्श नहीं है। यह तथ्यों की सूचना देती है। यह मनुष्य को अपनी आजीविका कमाने योग्य बनाती है। आम विषयों के अतिरिक्त मिस बीम के आदर्श स्कूल में विद्यार्थियों को मानवता और नागरिकता के पाठ पढ़ाए जाते थे। यहां विद्यार्थियों को दुर्भाग्य की वास्तविक जानकारी दी जाती थी।
प्रशिक्षण के दौरान प्रत्येक बच्चे का एक अन्धा होने का दिन, एक बहरा होने का दिन और एक गूंगा होने का दिन होता था। अन्धे होने के दिन के दौरान उनकी आंखों पर पट्टी बांध दी जाती थी। पट्टी रात को बांध दी जाती थी। एक दिन अन्धे बने रहने पर बच्चे को महसूस होता था कि अन्धे होना कितना दुर्भाग्यपूर्ण था। इसी तरह बच्चों को बहरे और गूंगे लोगों की कठिनाइयों का पता चलता था।
Objective Type Questions
This question will consist of 3 objective type questions carrying one mark each. These objective questions will include questions to be answered in one word to one sentence or fill in the blank or true/false or multiple choice type questions.
Question 1.
What does the author tell us about Miss Beam ?
Answer:
He tells us that Miss Beam was a middle-aged, kindly, understanding and impressive lady.
Question 2.
What was the real aim of Miss Beam’s school ?
Answer:
Its real aim was to make the students thoughtful, helpful and sympathetic citizens.
Question 3.
Why did the author feel sorry for some of the children ?
Answer:
He felt sorry for some children because they seemed to be handicapped.
Question 4.
Were the children playing in the ground really physically handicapped ?
Answer:
They were not really handicapped.
![]()
Question 5.
Why were the children acting to be blind, deaf or lame ?
Answer:
The children were acting to be blind, lame and deaf to have experience of misfortune.
Question 6.
What is the educative value of a blind, deaf or lame day?
Answer:
Students get an idea of the discomfort of handicapped persons and then they have sympathy for the handicapped.
Question 7.
Which day is the most difficult for children ?
Answer:
The blind day is the most difficult for children.
Question 8.
Who did Miss Beam lead the author to ?
Answer:
Miss Beam led the author to the girl whose eyes were bandaged.
Question 9.
How did the girl with bandaged eyes feel on her blind day?
Answer:
All the time she feared that she was going to be hit by something.
Question 10.
What does the girl with the bandaged eyes tell the author about her guides ?
Answer:
She tells the author that the guides were very good.
Question 11.
What, according to the girl with the bandaged eyes, is almost a fun ?
Answer:
According to her, hopping about with a crutch is almost a fun.
Question 12.
Why does the girl with the bandaged eyes say that her head aches all the time on her blind day?
Answer:
She says that her head aches all the time just from dodging things that are not there.
Question 13.
What does the girl, with the bandaged eyes, tell the author about the head girl ?
Answer:
She says that she is very decent.
![]()
Question 14.
What does the girl with the bandaged eyes say about the gardener ?
Answer:
She says that he is hundreds of years old.
Question 15.
What made Miss Beam think that there was something in her system?
Answer:
Miss Beam was right to think so because her school had taught the author to share the sorrows of others.
Question 16.
Choose the correct option:
(i) Miss Beam was a cruel lady.
(ii) Miss Beam was a young lady, teaching in a school.
(iii) Miss Beam was a middle aged, kindly and impressive lady.
Answer:
(iii) Miss Beam was a middle aged, kindly and impressive lady.
Question 17.
Choose the correct option :
(i) The aim of Miss Beam’s school was to make the students thoughtful, helpful and sympathetic citizens.
(ii) The object of Miss Beam’s school was to make the students bookworms.
(iii) Miss Beam’s school made the students into good sportspersons.
Answer:
(i) The aim of Miss Beam’s school was to make the students thoughtful, helpful and sympathetic citizens.
Question 18.
Choose the correct option :
The author was sorry for some children of Miss Beam’s school because they were :
(i) poor.
(ii) handicapped.
(iii) sick.
Answer:
(ii) handicapped.
Question 19.
Write True or False as appropriate :
(i) The children in Miss Beam’s school were handicapped.
(ii) They were acting to be handicapped.
(iii) They were being treated for being handicapped.
Answer:
(i) False
(ii) True
(iii) False.
Question 20.
Write True or False as appropriate :
The most difficult day for the students in Miss Beam’s school was the lame day.
Answer:
False.
Question 21.
Write True or False as appropriate :
The most difficult day for the students in Miss Beam’s school was the deaf day.
Answer:
False.
![]()
Question 22.
Write True or False as appropriate :
The most difficult day for the students in Miss Beam’s school was the blind day.
Answer:
True.
Question 23.
The writer had heard of the ……….. of the system of Miss Beam’s school. (Fill up the blank)
Answer:
originality
Question 24.
The bandaged girl tells the writer that the gardener was …………. of years old. (Fill in the blank)
Answer:
hundreds
Question 25.
What was the name of the bandaged girl ?
Answer:
Millie.
The School for Sympathy Summary in English
The School for Sympathy Introduction:
In this essay the writer tells us about a new type of school. As the name indicates, its purpose is to create sympathy among its students for the lame, the blind and the handicapped. It teaches all the subjects taught by other schools. But it differs from other schools in one important aspect. It makes its students good citizens.
The School for Sympathy Summary in English:
The writer had heard a lot about Miss Beam’s School for Sympathy. One day he got the chance to visit it. He saw a twelve-year old girl. Her eyes were covered with a bandage. An eight-year old boy was leading her carefully between the flower-beds.
After that the author met Miss Beam. She was a middle-aged, kindly and understanding lady. He asked her questions about her way of teaching. She told him that the teaching methods in her school were very simple. The students were taught spelling, arithmetic and writing.
The author told Miss Beam that he had heard a lot about the originality of her teaching method. Miss Beam told him that the real aim of her school was to make the students thoughtful. She wanted to make them helpful and sympathetic citizens. She added that parents sent their children to her school gladly. She then asked the writer to look out of the window.
![]()
The author looked out of the window. He saw a large garden and playground. Many children were playing there. He told Miss Beam that he felt sorry for the physically handicapped. Miss Beam laughed at it. She explained to him that they were not really handicapped. It was the blind day for a few while for some it was the deaf day. There were still others for whom it was the lame day. Then she explained the system.
To make the students understand misfortune, they were made to have experience of misfortunes. In the course of the term every child had one blind day, one lame day, one deaf day, one maimed day and one dumb day. On the blind day, their eyes were bandaged. They did everything with the help of other children. It was educative to both the blind and the helpers.
Miss Beam told the author that the blind day was very difficult for the children. But some of the children feared the dumb day. On the dumb day, the child had to exercise willpower because the mouth was not bandaged. Miss Beam introduced the author to a girl whose eyes were bandaged. The author asked her if she ever peeped. She told him that it would be cheating. She also told the author that she had no idea of the difficulties of the blind.
All the time she feared that she was going to be hit by something. The author asked her if her guides were good to her. She replied that they were very good. She also informed the author that those who had been blind already were the best guides. The author walked with the girl leading her to the playground. She told him that the blind day was the worst day.
She didn’t feel so bad on the maimed day, lame day and deaf day. The girl asked the author where they were at the moment. He told her that they were going towards the house. He also told her that Miss Beam was walking up and down the terrace with a tall girl. The blind girl asked what that tall girl was wearing.
![]()
When the author told her about the tall girls dress, she at once made out that she was Millie. The author described the surroundings to her. He felt that as a guide to the blind, one had to be thoughtful. He was full of praise for Miss Beam’s system of education which made the student sympathetic and kind. The writer himself had become ten times more thoughtful.
The School for Sympathy Summary in Hindi
The School for Sympathy Introduction:
इस लेख में लेखक एक नये प्रकार के स्कूल के बारे बतलाता है। जैसा कि इसके नाम से स्पष्ट होता है, इसका उद्देश्य उसके छात्रों में लंगड़ों, अन्धों और अपंगों के लिए सहानुभूति पैदा करना है। इस स्कूल में वे तमाम विषय पढ़ाये जाते हैं जो कि अन्य स्कूलों में पढ़ाये जाते हैं। लेकिन यह स्कूल दूसरे स्कूलों से एक महत्त्वपूर्ण पक्ष में भिन्न है। यह अपने छात्रों को अच्छे नागरिक बनाता है।
The School for Sympathy Summary in Hindi:
लेखक ने Miss Beam के सहानुभूति के लिए स्कूल के बारे में बहुत कुछ सुन रखा था। एक दिन उसे यह देखने का अवसर मिला। उसने एक 12 वर्ष की लड़की देखी। उसकी आंखें पट्टी से ढकी हुई थीं। एक आठ वर्ष का लड़का बड़ी सावधानी के साथ फूलों की क्यारियों में से उसका मार्ग-दर्शन कर रहा था।
उसके बाद लेखक मिस बीम को मिला। वह अधेड़ उम्र की दयालु समझदार स्त्री थी। उसने उससे पढ़ाने के ढंग के बारे में पूछा। उसने उसे बताया कि उसके स्कूल में पढ़ाने का ढंग बहुत सादा था। विद्यार्थियों को हिज्जे करना, गणित और लिखना सिखाया जाता था।
लेखक ने मिस बीम को बताया कि वह उसके पढ़ाने के ढंग की मौलिकता के विषय में बहुत कुछ सुन चुका था। मिस बीम ने उसे बताया कि उसके स्कूल का वास्तविक ध्येय विद्यार्थियों को विचारशील बनाना था। वह अपने विद्यार्थियों को सहायक और सहानुभूतिशील नागरिक बनाना चाहती थी। उसने फिर कहा कि माता-पिता बच्चों को उसके स्कूल में खुशी से भेजते थे। उसने तब लेखक को खिड़की से बाहर देखने को कहा। .
लेखक ने खिड़की से बाहर देखा। उसने एक बड़ा बाग़ और खेल का मैदान देखा। बहुत से बच्चे वहीं खेल रहे थे। लेखक ने मिस बीम को बताया कि उसे इन अपंग बच्चों से हमदर्दी है। मिस बीम हंस पड़ी। उसने बताया कि वे अपंग बच्चे नहीं थे। कुछ बच्चों के लिए यह ‘अन्धा रहने का दिन था’ और कुछ के लिए बहरा रहने का दिन था। कुछ बच्चों के लिए यह लंगड़ा रहने का दिन था। फिर मिस बीम ने शिक्षा प्रणाली समझाई।
विपत्ति से पीड़ित मनुष्य की भावनाओं का अनुभव कराने के लिए बच्चों को विपत्ति में भागीदार बनाया जाता था। शिक्षा के दौरान हर बच्चे को एक दिन अन्धा, एक दिन बहरा, एक दिन लंगड़ा और एक दिन गूंगा रहना पड़ता था। अन्धे रहने वाले दिन उसकी आंखों पर पट्टी बांध दी जाती थी। वे हर काम दूसरे बच्चों की सहायता से करते थे।
यह अन्धे लड़के और उसके सहायक दोनों के लिए शिक्षाप्रद होता था। मिस बीम ने लेखक को कहा कि अन्धा रहने वाला दिन बच्चों के लिए कठिन होता था। किन्तु कुछ बच्चे गूंगे रहने वाले दिन से डरते थे। गूंगे रहने वाले बच्चे को इच्छा शक्ति प्रयोग करनी पड़ती थी क्योंकि मुंह पर
![]()
पट्टी नहीं बांधी जाती थी। मिस बीम ने लेखक को एक अन्धी लड़की से मिलवाया। उसकी आंखों पर पट्टी बन्धी थी। लड़की और लेखक अकेले रह गए। लेखक ने पूछा क्या वह कभी पट्टी में से झांकती है। लड़की ने बताया यह धोखा होगा। उसने यह भी बताया कि अन्धे मनुष्य की कठिनाइयों का उसे कोई भी अनुमान नहीं था।
उसे हर समय यही डर लगा रहता था वह किसी चीज़ से टकराने वाली थी। लेखक ने पूछा क्या उसके सहायक उसके प्रति अच्छे थे। उसने उत्तर दिया कि वे काफ़ी अच्छे थे। उसने लेखक को यह भी बताया कि जो सहायक पहले अन्धे रह चुके थे वे सबसे बढ़िया थे।
लेखक लड़की को खेल के मैदान तक ले आया। उस अन्धी लड़की ने बताया कि ‘अन्धा दिन’ सबसे बुरा था। उसने ‘लंगड़े दिन’, ‘बहरे दिन’ ऐसा बुरा महसूस नहीं किया था। अन्धी लड़की ने पूछा कि वे इस समय कहां थे। लेखक ने बताया कि वे मकान की ओर जा रहे थे। उसने यह भी बताया कि मिस बीम एक लम्बी लड़की के साथ बरामदे में टहल रही थी। अन्धी लड़की ने पूछा कि उस लम्बी लड़की ने क्या पहना है।
जब लेखक ने लड़की को उसकी वेश-भूषा के विषय में बताया तो अन्धी लड़की एकदम भांप गई कि यह मिल्ली है। लेखक ने लड़की के आस-पड़ोस का वर्णन किया। उसने अनुभव किया कि अन्धे मनुष्य का पथ-प्रदर्शक बनने के लिए विचारवान् बनना पड़ता है। लेखक ने मिस बीम की शिक्षा प्रणाली की बहुत सराहना की। इस शिक्षा प्रणाली से विद्यार्थी हमदर्द और दूसरों के प्रति दयालु बनता था। लेखक स्वयं दस गुना अधिक विचारशील बन गया था।
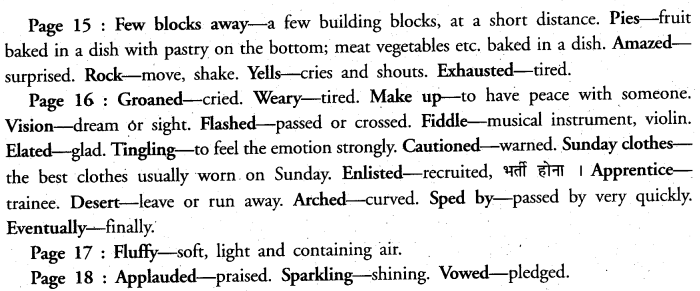
Word Meanings:

Class 12 PSEB Solutions Supplementary Reading