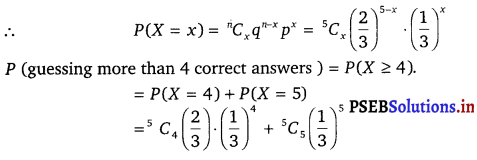Punjab State Board PSEB 12th Class Environmental Education Book Solutions Chapter 1 ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ / ਜੀਵ ਅਨੇਕਰੂਪਤਾ (ਭਾਗ-1) Textbook Exercise Questions and Answers.
PSEB Solutions for Class 12 Environmental Education Chapter 1 ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ / ਜੀਵ ਅਨੇਕਰੂਪਤਾ (ਭਾਗ-1)
Environmental Education Guide for Class 12 PSEB ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ / ਜੀਵ ਅਨੇਕਰੂਪਤਾ (ਭਾਗ-1) Textbook Questions and Answers
ਛੋਟੇ ਉੱਤਰਾਂ ਵਾਲੇ ਪ੍ਰਸ਼ਨ (Short Answer Type Questions)
ਪ੍ਰਸ਼ਨ 1.
ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਤੋਂ ਤੁਹਾਡਾ ਕੀ ਭਾਵ ਹੈ ? ਉਦਾਹਰਣਾਂ ਦਿਓ ।
ਉੱਤਰ-
ਕਿਸੇ ਖੇਤਰ ਜਾਂ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਵਿਚ ਪਾਏ ਜਾਂਦੇ ਸਾਰੇ ਦੇ ਸਾਰੇ ਪੌਦਿਆਂ, ਜੰਤੂਆਂ ਅਤੇ ਸੂਖ਼ਮਜੀਵਾਂ ਦੇ ਉਲੇਖ ਨੂੰ ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਜਾਂ ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਆਖਿਆਂ ਜਾਂਦਾ ਹੈ । ਇਹ ਕਿਸੇ ਖੇਤਰ ਵਿਚ ਪਾਏ ਜਾਣ ਵਾਲੇ ਸਾਰੇ ਪੌਦਿਆਂ ਅਤੇ ਜੰਤੂਆਂ ਦੀਆਂ ਕਿਸਮਾਂ ਅਤੇ ਪਰਿਵਰਤਤਾ (Variability) ਨੂੰ ਦਰਸਾਉਂਦਾ ਹੈ ।
ਉਦਾਹਰਨ – ਕੁੱਤਾ, ਬਿੱਲੀ, ਕਿਰਲੀ, ਟਾਹਲੀ, ਕਿੱਕਰ ਅਤੇ ਬੈਕਟੀਰੀਆ ਆਦਿ ।
ਪ੍ਰਸ਼ਨ 2.
ਆਪਣੇ ਚੌਗਿਰਦੇ ਵਿਚਲੇ ਕੁੱਝ ਦਵਾਈਆਂ ਦੇਣ ਵਾਲੇ ਪੌਦਿਆਂ ਦੇ ਨਾਮ ਅਤੇ ਲਾਭ ਦੱਸੋ ।
ਜਾਂ
ਆਪਣੇ ਇਲਾਕੇ ਦੇ ਕੁੱਝ ਦਵਾਈਆਂ ਦੇਣ ਵਾਲੇ ਪੌਦਿਆਂ ਦੇ ਨਾਂ ਅਤੇ ਫਾਇਦੇ ਦੱਸੋ ।
ਉੱਤਰ-
ਦਵਾਈਆਂ (ਔਸ਼ਧੀਆਂ ਦੇਣ ਵਾਲੇ ਕੁੱਝ ਪੌਦੇ ਜਿਹੜੇ ਸਾਡੇ ਚੌਗਿਰਦੇ ਆਲੇਦੁਆਲੇ ਵਿਚ ਮਿਲਦੇ ਹਨ, ਉਹ ਹੇਠ ਦਿੱਤੇ ਜਾਂਦੇ ਹਨ-
- ਨਿੰਮ (Neem) – ਨਿੰਮ ਦੇ ਸੁਕੇ ਹੋਏ ਪੱਤਿਆਂ ਦੀ ਵਰਤੋਂ ਕੀੜਿਆਂ ਅਤੇ ਭੰਬਟਾਂ (Moths) ਦੇ ਅਪਕਰਸ਼ਨ (Repelling) ਲਈ ਕੀਤੀ ਜਾਂਦੀ ਹੈ । ਪੱਤਿਆਂ ਤੋਂ ਤਿਆਰ ਕੀਤੇ ਗਏ ਸਫੂਫ ਪਾਊਡਰ) ਤੋਂ ਤਿਆਰ ਕੀਤੀ ਗਈ ਲੇਵੀ ਪੁਲਟਸ ਦੀ ਵਰਤੋਂ ਕੀਟਾਣੂ ਰੋਧਕ (Antibacterial) ਵਜੋਂ ਕੀਤੀ ਜਾਂਦੀ ਹੈ । ਨਿੰਮ ਦੀਆਂ ਨਰਮ-ਨਰਮ ਅਤੇ ਕੁਲੀਆਂ ਸ਼ਾਖਾਂ ਨੂੰ ਦਾਤਨ ਵਜੋਂ ਵਰਤਦੇ ਹਨ ।
- ਤੁਲਸੀ (Tulsi) – ਇਸ ਦੀ ਵਰਤੋਂ ਖਾਂਸੀ ਅਤੇ ਜ਼ੁਕਾਮ ਆਦਿ ਦਾ ਇਲਾਜ ਕਰਨ ਲਈ ਕੀਤੀ ਜਾਂਦੀ ਹੈ ।
- ਕੁਆਰ-ਗੰਦਲ ਜਾਂ ਅਲੋਵੀਰਾ (Aloe-vera) – ਇਸ ਪੌਦੇ ਦੇ ਪੱਤਿਆਂ ਦੀ ਵਰਤੋਂ ਚਮੜੀ ਦੇ ਵਾਸਤੇ ਗੁਣਕਾਰੀ ਕਰੀਮਾਂ ਆਦਿ ਤਿਆਰ ਕਰਨ ਤੋਂ ਇਲਾਵਾ ਇਸ ਦੇ ਪੱਤਿਆਂ ਤੋਂ ਅਚਾਰ ਵੀ ਤਿਆਰ ਕੀਤਾ ਜਾਂਦਾ ਹੈ ।
![]()
ਪ੍ਰਸ਼ਨ 3.
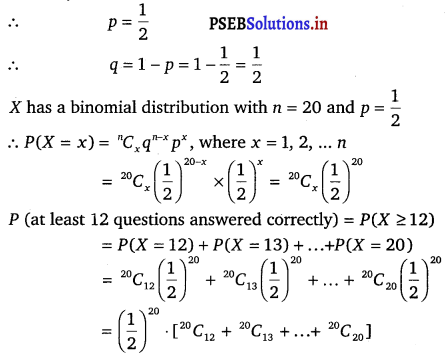
ਉਹ ਕਿਹੜੀਆਂ-ਕਿਹੜੀਆਂ ਬਾਹਰੀ ਤਾਕਤਾਂ ਹਨ, ਜੋ ਕੁਦਰਤ ਵਿਚ ਅਸੰਤੁਲਨ ਪੈਦਾ ਕਰਦੀਆਂ ਹਨ ?
ਜਾਂ
ਕੁਦਰਤ ਵਿਚ ਅਸੰਤੁਲਨ ਪੈਦਾ ਕਰਨ ਵਾਲੀਆਂ ਬਾਹਰਲੀਆਂ ਸ਼ਕਤੀਆਂ ਬਾਰੇ ਲਿਖੋ ।
ਉੱਤਰ-
ਕੁਦਰਤ ਵਿਚ ਅਸੰਤੁਲਨ ਪੈਦਾ ਕਰਨ ਵਾਲੀਆਂ ਬਾਹਰੀ ਤਾਕਤਾਂ ਵਿਚ ਹੜ੍ਹ, ਹਨੇਰੀਆਂ, ਅੱਗ (ਲੱਗਣਾ) ਅਤੇ ਵਿਨਾਸ਼ਕਾਰੀ ਜੰਤੂ (Pests) ਆਦਿ ਸ਼ਾਮਿਲ ਹਨ । ਇਨ੍ਹਾਂ ਬਾਹਰੀ ਤਾਕਤਾਂ ਨਾਲ ਅਸੰਤੁਲਿਤ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀਆਂ ਦਾ ਵਤੀਰਾ, ਸੰਤੁਲਿਤ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀਆਂ ਦੇ ਮੁਕਾਬਲੇ ਕਾਫ਼ੀ ਅਲੱਗ ਕਿਸਮ ਦਾ ਹੁੰਦਾ ਹੈ । ਜਿਵੇਂ ਕਿ ਜ਼ਮੀਨ ਦੇ ਖਿਸਕਣ ਕਾਰਨ ਜਾਂ ਰੁੱਖ ਦੇ ਡਿੱਗਣ ਨਾਲ ਜੇਕਰ ਕੋਈ ਪਾੜ (Gap) ਪੈਦਾ ਹੋ ਜਾਂਦਾ ਹੈ, ਤਾਂ ਅਜਿਹੀ ਜਗ੍ਹਾ (ਪਾੜ) ਵਿਚ ਨਵੇਂ ਪੌਦੇ ਉੱਗ ਆਉਂਦੇ ਹਨ, ਜਿਸ ਦੇ ਸਿੱਟੇ ਵਜੋਂ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਵਿਚ ਮੁੜ ਸੰਤੁਲਨ ਪੈਦਾ ਹੋ ਜਾਂਦਾ ਹੈ । ਇਸ ਦੇ ਵਿਪਰੀਤ ਜੇਕਰ
ਅਸੰਤੁਲਿਤ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਵਿਚ ਬਾਹਰੀ ਤਾਕਤਾਂ ਕਾਰਨ ਕੋਈ ਪਾੜ ਆਦਿ ਉਤਪੰਨ ਹੋ ਜਾਵੇ, ਤਾਂ ਅਜਿਹੇ ਸਥਾਨਾਂ ਤੇ ਰੁੱਖ ਆਦਿ ਨਹੀਂ ਉੱਗਦੇ, ਜਿਸ ਦੇ ਫਲਸਰੂਪ ਇਹ ਅਸੰਤੁਲਿਤ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਵਿਚ ਸੰਤੁਲਨ ਉਤਪੰਨ ਨਹੀਂ ਹੁੰਦਾ । ਪਰ ਇਸ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਦੀ ਥਾਂ ਕੋਈ ਨਵੀਂ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਪੈਦਾ ਹੋ ਸਕਦੀ ਹੈ, ਜਿਵੇਂ ਕਿ-ਐਮਾਜ਼ੋਨ ਦੇ ਜੰਗਲਾਂ ਦੀ ਜ਼ਮੀਨ, ਜਿਸ ਦੀ ਵਰਤੋਂ ਖੇਤੀ ਕਾਰਜਾਂ ਲਈ ਕੀਤੀ ਜਾਂਦੀ ਸੀ, ਦੇ ਤਿਆਗ ਕਰਨ (Abandon) ਦੇ ਫਲਸਰੂਪ ਇਹ ਜ਼ਮੀਨ ਘਾਹ ਦੇ ਮੈਦਾਨ (ਸਵਾਨਾ ਵਿਚ ਤਾਂ ਤਬਦੀਲ ਤਾਂ ਹੋ ਗਈ, ਪਰ ਜੰਗਲ ਵਿਚ ਪਰਿਵਰਤਿਤ ਨਾ ਹੋ ਸਕੀ ।
ਪ੍ਰਸ਼ਨ 4.
ਸ੍ਰੋਤਾਂ ਦੀ ਸੀਮਾਬੱਧਤਾ (Resource Limitations) ਤੋਂ ਕੀ ਭਾਵ ਹੈ ?
ਉੱਤਰ-
ਦੀ ਸੀਮਾਬੱਧਤਾ (Resource Limitations) – ਕੁਦਰਤੀ ਸਰੋਤਾਂ ਤੋਂ ਜਿਹੜੀ ਵੀ ਵਸਤੂ ਅਸੀਂ ਆਪਣੀ ਉਤਰਜੀਵਤਾ ਲਈ ਪ੍ਰਾਪਤ ਕਰਦੇ ਹਾਂ, ਉਸਨੂੰ ਸਾਧਨ ਆਖਦੇ ਹਨ । ਸਾਧਨਾਂ ਦੀ ਸੀਮਾਬੱਧਤਾ ਤੋਂ ਭਾਵ ਹੈ ਕਿ ਇਨ੍ਹਾਂ ਕੁਦਰਤੀ ਸਾਧਨਾਂ ਦੀ ਵੀ ਕੋਈ ਹੱਦ ਹੈ ਅਤੇ ਸਾਨੂੰ ਇਨ੍ਹਾਂ ਸਾਧਨਾਂ ਦੀ ਵਰਤੋਂ ਬੜੀ ਸਮਝਦਾਰੀ ਨਾਲ ਕਰਨੀ ਚਾਹੀਦੀ ਹੈ । ਜ਼ਮੀਨ, ਹਵਾ, ਪਾਣੀ ਅਤੇ ਖਣਿਜ ਪਦਾਰਥ ਕੁਦਰਤੀ ਸਾਧਨਾਂ ਵਿਚ ਸ਼ਾਮਿਲ ਹਨ । ਇਹਨਾਂ ਦੀ ਵਰਤੋਂ ਬੜੀ ਸਮਝਦਾਰੀ ਨਾਲ ਕਰਨੀ ਚਾਹੀਦੀ ਹੈ ।
ਭੂਮੀ ਸ੍ਰੋਤਾਂ ਦੀ ਸੀਮਾਬੱਧਤਾ (Limitations of Land Resources) – ਖੇਤੀ ਯੋਗ ਖੇਤਰਫਲ ਬਹੁਤ ਹੀ ਘੱਟ ਹੈ । ਸਾਡੇ ਲਈ ਖੇਤੀ ਯੋਗ ਖੇਤਰਫਲ ਹੀ ਲਾਹੇਵੰਦ ਹੈ, ਪਰ ਇਸ ਜ਼ਮੀਨ ‘ਤੇ ਬੜਾ ਦਬਾਅ ਪੈ ਰਿਹਾ ਹੈ ।
ਪਾਣੀ ਦੇ ਸੋਤਾਂ ਦੀ ਸੀਮਾਬੱਧਤਾ (Limitations of Water Resources) – ਸਾਡੇ, ਪੌਦਿਆਂ ਅਤੇ ਪ੍ਰਾਣੀਆਂ ਦੇ ਜੀਵਨ ਲਈ ਪਾਣੀ ਦੀ ਬੜੀ ਮਹੱਤਤਾ ਹੈ । ਪਰ ਪਿੰਡਾਂ ਅਤੇ ਕਸਬਿਆਂ ਵਿਚ ਪਾਣੀ ਦੀ ਉਪਲੱਬਧੀ ਵਿਚ ਮੁਸ਼ਕਲਾਂ ਪੇਸ਼ ਆ ਰਹੀਆਂ ਹਨ । ਮਨੁੱਖਾਂ ਦੁਆਰਾ ਪਾਣੀ ਦੀ ਜ਼ਿਆਦਾਤਰ ਵਰਤੋਂ ਠੀਕ ਅਤੇ ਸੁਚੱਜੇ ਢੰਗ ਨਾਲ ਨਹੀਂ ਕੀਤੀ ਜਾਂਦੀ । ਪੀਣ ਵਾਲੇ ਪਾਣੀ ਦੀ ਮੰਗ ਹਰ ਦਿਨ ਵੱਧਦੀ ਜਾਂਦੀ ਹੈ ।

ਖਣਿਜੀ ਸੋਤਾਂ ਦੀ ਸੀਮਾਬੱਧਤਾ (Limitations of Mineral Resources) – ਕੋਲਾ, ਲੋਹਾ, ਅਤੇ ਤਾਂਬਾ ਸਾਡੇ ਦੇਸ਼ ਦੇ ਮੁੱਖ ਖਣਿਜ ਹਨ ਅਤੇ ਇਨ੍ਹਾਂ ਕੁਦਰਤੀ ਸਾਧਨਾਂ ਦੀ ਮਾਤਰਾ ਸੀਮਿਤ ਹੈ । ਜਿਸ ਦਰ ਨਾਲ ਇਨ੍ਹਾਂ ਸਾਧਨਾਂ ਦੀ ਵਰਤੋਂ ਕੀਤੀ ਜਾ ਰਹੀ ਹੈ, ਉਸ ਹਿਸਾਬ ਨਾਲ ਇਹ ਬਹੁਤ ਜਲਦੀ ਖ਼ਤਮ ਹੋ ਜਾਣਗੇ ।
ਸਾਧਨਾਂ ਦੀ ਬਚੀ ਹੋਈ ਰਹਿੰਦ-ਖੂੰਹਦ ਦਾ ਪੁਨਰ-ਚੱਕਰਣ ਕਰਕੇ, ਇਨ੍ਹਾਂ ਨੂੰ ਮੁੜ ਵਰਤੋਂ ਵਿਚ ਲਿਆਂਦਾ ਜਾਣਾ ਚਾਹੀਦਾ ਹੈ ।
ਜੈਵਿਕ ਸੋਤਾਂ ਦੀ ਸੀਮਾਬੱਧਤਾ (Limitations of Biological Resources) – ਵਣ ਅਤੇ ਜੰਗਲੀ ਜੀਵਨ ਹੀ ਸਾਡੇ ਜੈਵਿਕ ਸਾਧਨ ਹਨ । ਵਣਾਂ ਤੋਂ ਸਾਨੂੰ ਕਈ ਪ੍ਰਕਾਰ ਦੀਆਂ ਲਾਹੇਵੰਦ ਵਸਤਾਂ ਪ੍ਰਾਪਤ ਹੁੰਦੀਆਂ ਹਨ ਜਿਵੇਂ ਕਿ ਇਮਾਰਤੀ ਲੱਕੜੀ, ਈਂਧਨ, ਔਸ਼ਧੀਆਂ, ਸ਼ਿੰਗਾਰ ਦਾ ਸਾਮਾਨ ਆਦਿ । ਜੰਗਲ, ਕਈ ਪ੍ਰਕਾਰ ਦੇ ਜਾਨਵਰਾਂ ਦੇ ਨਿਵਾਸ ਸਥਾਨਾਂ ਵਜੋਂ ਕਾਰਜ ਕਰਦੇ ਹਨ ਅਤੇ ਵਣਾਂ ਵਿਚ ਕਈ ਪ੍ਰਕਾਰ ਦੇ ਕੀਟ, ਰੀਂਗਣ ਵਾਲੇ ਜਾਨਵਰ ਅਤੇ ਥਣਧਾਰੀ ਜੀਵ ਪਾਏ ਜਾਂਦੇ ਹਨ ।
ਵਣ ਕਾਰਬਨ ਡਾਈਆਕਸਾਈਡ ਅਤੇ ਆਕਸੀਜਨ ਦਾ ਹਰ ਰੋਜ਼ ਪੁਨਰ ਚੱਕਰਣ ਕਰਦੇ ਹਨ । ਵਰਖਾ ਵਣ (Rain forests) ਪਾਣੀ ਦੇ ਚੱਕਰ ਵਿਚ ਭੂਮਿਕਾ ਨਿਭਾਉਂਦੇ ਹਨ ।
ਪ੍ਰਸ਼ਨ 5.
ਜੈਵਿਕ-ਵਿਭਿੰਨਤਾ ਦੀ ਪਰਿਸਥਿਤੀ ਸੰਬੰਧੀ ਕੀ ਭੂਮਿਕਾ ਹੈ ?
ਉੱਤਰ-
ਕਿਸੇ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਦੀ ਜੀਵਕਾ ਉਸ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਵਿਚ ਮੌਜੂਦ ਜਾਤੀ ਵਿਭਿੰਨਤਾ ‘ਤੇ ਨਿਰਭਰ ਕਰਦੀ ਹੈ । ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਵਿਚ ਮੌਜੂਦ ਹਰੇਕ ਜਾਤੀ ਦੀ ਵਿਸ਼ੇਸ਼ ਭੂਮਿਕਾ ਹੈ ਜਿਸ ਨੂੰ ਪਰਿਸਥਿਤਿਕ ਛੋਟਾ ਠਿਕਾਣਾ ਜਾਂ ਪਰਿਸਥਿਤਿਕ ਨਿਚ (Ecological niche) ਕਹਿੰਦੇ ਹਨ | ਆਪਣੀ ਹੋਂਦ ਦੇ ਲਈ ਹਰੇਕ ਜਾਤੀ ਦੇ ਵਾਸਤੇ ਯੋਗ ਨਿਵਾਸ ਸਥਾਨ ਦੇ ਇਲਾਵਾ ਉੱਥੇ ਪਾਈਆਂ ਜਾਣ ਵਾਲੀਆਂ ਦੂਸਰੀਆਂ ਜਾਤੀਆਂ ਦੇ ਇਲਾਵਾ ਭੌਤਿਕ ਵਾਤਾਵਰਣ ਦਾ ਹੋਣਾ ਵੀ ਜ਼ਰੂਰੀ ਹੁੰਦਾ ਹੈ ।
ਵੱਖ-ਵੱਖ ਕਿਸਮਾਂ ਦੇ ਵਾਤਾਵਰਣ ਵਿਚਲੇ ਸਮੁਦਾਇਆਂ ਵਿਚ ਪਾਈਆਂ ਜਾਣ ਵਾਲੀਆਂ ਜਾਤੀਆਂ ਦੇ ਲੱਛਣ ਇਕ ਸਮਾਨ ਹੋਣ ਦੀ ਵਜ੍ਹਾ ਕਰਕੇ ਇਨ੍ਹਾਂ ਨੂੰ ਸਥਿਰਤਾ ਮਿਲਦੀ ਹੈ । ਉਦਾਹਰਣ ਵਜੋਂ ਕਿਸੇ ਸਮੁਦਾਇ ਵਿਚ ਪਾਏ ਜਾਂਦੇ ਰੁੱਖਾਂ (ਪੌਦਿਆਂ) ਦੀਆਂ ਪਾਣੀ ਪ੍ਰਾਪਤੀ ਸੰਬੰਧੀ ਜ਼ਰੂਰਤਾਂ ਇਕ ਸਮਾਨ ਹੁੰਦੀਆਂ ਹਨ ਅਤੇ ਜੇਕਰ ਵਰਖਾ ਘੱਟ ਹੋਵੇ ਜਾਂ ਨਾ ਹੋਵੇ ਤਾਂ ਪਾਣੀ ਦੀ ਘਾਟ ਕਾਰਨ ਇਨ੍ਹਾਂ ਸਾਰੇ ਦੇ ਸਾਰੇ ਰੁੱਖਾਂ ਨੂੰ ਪਾਣੀ ਦੀ ਘਾਟ ਹੋ ਜਾਣ ਦੇ ਫਲਸਰੂਪ ਹਾਨੀ ਪੁੱਜ ਸਕਦੀ ਹੈ ਅਤੇ ਅਜਿਹੀ ਹਾਲਤ ਦੇ ਉਤਪੰਨ ਹੋ ਜਾਣ ਨਾਲ ਉਪਜ ‘ਤੇ ਵੀ ਮਾੜਾ ਪ੍ਰਭਾਵ ਪੈ ਜਾਵੇਗਾ । ਇਸ ਦੇ ਉਲਟ ਜੇਕਰ ਇੱਕੋ ਹੀ ਜਾਤੀ ਦੇ ਵੱਖ-ਵੱਖ ਮੈਂਬਰਾਂ ਵਿਚਲੇ ਜੀਨਾਂ ਵਿਚ ਵਿਭਿੰਨਤਾਵਾਂ (Variation) ਦੀ ਮੌਜੂਦਗੀ ਦੇ ਕਾਰਨ, ਇਨ੍ਹਾਂ ਪੌਦਿਆਂ ਵਿਚ ਪਾਣੀ ਦੀਆਂ ਜ਼ਰੂਰਤਾਂ ਸੰਬੰਧੀ ਅੰਤਰ ਹੋਣਗੇ । ਅਜਿਹਾ ਹੋਣ ਦੀ ਸੂਰਤ ਵਿਚ ਇਨ੍ਹਾਂ ਪੌਦਿਆਂ ਨੂੰ ਹਰ ਸਾਲ ਵਾਤਾਵਰਣ ਦੇ ਮਾੜੇ ਪ੍ਰਭਾਵਾਂ ਤੋਂ ਕੋਈ ਹਾਨੀ ਨਹੀਂ ਪੁੱਜੇਗੀ ਅਤੇ ਹਰ ਸਾਲ ਇਨ੍ਹਾਂ ਤੋਂ ਪ੍ਰਾਪਤ ਹੋਣ ਵਾਲੀ ਉਪਜ (ਝਾੜ) ਵੀ ਤਸੱਲੀਬਖ਼ਸ਼ ਹੋਵੇਗੀ ।
ਪ੍ਰਸ਼ਨ 6.
ਤੁਹਾਡਾ ਪਿੰਡ/ਕਸਬਾ/ਸ਼ਹਿਰ ਕਿਸ ਪ੍ਰਕਾਰ ਦੇ ਪੌਦੇ ਅਤੇ ਜੰਤੂ ਵਿਭਿੰਨਤਾ ਜਾਤੀ ਲਈ ਮਸ਼ਹੂਰ ਹੈ ?
ਉੱਤਰ-
ਸਾਡਾ ਕਸਬਾ ਅੱਗੇ ਲਿਖੇ ਪੌਦਿਆਂ ਅਤੇ ਪ੍ਰਾਣੀਆਂ ਦੀ ਜਾਤੀ ਵਿਭਿੰਨਤਾ ਲਈ ਜਾਣਿਆ ਜਾਂਦਾ ਹੈ ।
ਪੌਦੇ (Plants) – ਅੰਬਾਂ ਦੇ ਬਾਗ, ਕੁੱਝ ਇਕ ਥਾਂਵਾਂ ਤੇ ਬੇਰੀਆਂ ਦੇ ਝੁੰਡ, ਟਾਹਲੀਆਂ ਦੇ ਅਤੇ ਸਫੈਦਿਆਂ ਦੇ ਜ਼ਖੀਰੇ, ਖਜੂਰਾਂ ਦੇ ਰੁੱਖ ਆਦਿ ।
ਪ੍ਰਾਣੀ – ਸੁਧਰੀਆਂ ਹੋਈਆਂ ਕਿਸਮਾਂ ਦੀਆਂ ਮੱਝਾਂ, ਗਾਵਾਂ, ਕੁੱਤੇ, ਬਿੱਲੀਆਂ, ਮੁਰਗੇ-ਮੁਰਗੀਆਂ ਅਤੇ ਵੱਖ-ਵੱਖ ਤਰ੍ਹਾਂ ਦੇ ਪੰਛੀ ਆਦਿ ।
![]()
ਪ੍ਰਸ਼ਨ 7.
ਜੈਵਿਕ ਅਨੇਕਰੂਪਤਾ ਦੀ ਸੁਹਜਾਤਮਕ ਮਹੱਤਤਾ ਦਾ ਵਰਣਨ ਕਰੋ ।
ਉੱਤਰ-
ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ/ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਦੀ ਸੁਹਜਾਤਮਕ ਪੱਖੋਂ ਬੜੀ ਮਹੱਤਤਾ ਹੈ । ਲੋਕ ਇਸ ਅਨੇਕਰੂਪਤਾ ਨੂੰ ਮਾਨਣ ਦੇ ਲਈ ਸੈਰ ਸਪਾਟਾ ਕਰਦੇ ਹਨ । ਰੁੱਖਾਂ ਅਤੇ ਵਣਾਂ ਵਿਚ ਜਾ ਕੇ ਜੰਗਲੀ ਜੀਵਨ ਦਾ ਆਨੰਦ ਮਾਣਦੇ ਹਨ । ਰੁੱਖਾਂ ਵਿਚ ਰੱਖੇ ਗਏ ਜੰਗਲੀ ਜੀਵਨ ਨੂੰ ਵੇਖ ਕੇ ਕਿਸ ਦਾ ਮਨ ਪਸੰਨ ਨਹੀਂ ਹੁੰਦਾ । ਨੱਚਦੇ ਹੋਏ ਮੋਰ ਨੂੰ ਵੇਖ ਕੇ ਹਰੇਕ ਮਨੁੱਖ ਕੁਦਰਤ ਦੀ ਤਾਰੀਫ਼ ਕਰਨ ਤੋਂ ਨਹੀਂ ਰਹਿ ਸਕਦਾ ।
ਕਲਕੱਤਾ ਦੇ ਬੋਟੈਨੀਕਲ ਬਾਗ਼ ਵਿੱਚ ਬੋਹੜ ਦੇ ਰੁੱਖ (Banyan Tree) ਦੇ ਵਿਕਾਸ ਅਤੇ ਵਿਸਤਾਰ ਨੂੰ ਵੇਖ ਕੇ ਆਦਮੀ ਦੇਖਦਾ ਹੀ ਰਹਿ ਜਾਂਦਾ ਹੈ ਅਤੇ ਇਹ ਵੇਖਣ ਦੀ ਕੋਸ਼ਿਸ਼ ਵੀ ਕਰਦਾ ਹੈ ਕਿ ਇਸ ਰੁੱਖ ਦਾ ਮੁੱਖ ਤਣਾ ਕਿੱਥੇ ਹੈ ?
ਪ੍ਰਸ਼ਨ 8.
ਕੁਨੀਨ ਕਿਸ ਪੌਦੇ ਤੋਂ ਪ੍ਰਾਪਤ ਹੁੰਦੀ ਹੈ ਅਤੇ ਕਿਸ ਕੰਮ ਆਉਂਦੀ ਹੈ ?
ਉੱਤਰ-
ਕੁਨੀਨ ਪੀਲੇ ਸਿਨਕੌਨਾ (Yellow Cinchona) ਨਾਂ ਦੇ ਰੁੱਖ ਦੀ ਛਾਲ (Bark) ਤੋਂ ਪ੍ਰਾਪਤ ਕੀਤੀ ਜਾਂਦੀ ਹੈ ਅਤੇ ਇਸ ਦਵਾਈ ਦੀ ਵਰਤੋਂ ਮਲੇਰੀਆ ਬੁਖਾਰ ਦੇ ਇਲਾਜ ਲਈ ਕੀਤੀ ਜਾਂਦੀ ਹੈ ।
ਵੱਡੇ ਉੱਤਰਾਂ ਵਾਲੇ ਪ੍ਰਸ਼ਨ (Long Answer Type Questions)
ਪ੍ਰਸ਼ਨ 1.
ਜੈਵਿਕ-ਵਿਭਿੰਨਤਾ/ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਦੀਆਂ ਕਦਰਾਂ-ਕੀਮਤਾਂ ਕੀ ਹਨ ?
ਜਾਂ
ਪੌਦਿਆਂ ਦੀ ਆਰਥਿਕ ਮਹੱਤਤਾ ਦੇ ਕੋਈ ਦੋ ਪਹਿਲੂ ਲਿਖੋ ।
ਉੱਤਰ-
ਮਨੁੱਖ ਜਾਤੀ ਲਈ ਜੈਵਿਕ-ਵਿਭਿੰਨਤਾ ਇਕ ਵੱਡਮੁੱਲੀ ਸੰਪਦਾ ਹੈ । ਜ਼ਿੰਦਗੀ ਦੇ ਸਫਰ ਦਾ ਕੋਈ ਵੀ ਪੜਾਅ ਅਜਿਹਾ ਨਹੀਂ ਹੈ ਜਿਸ ਵਿਚ ਜੈਵਿਕ-ਵਿਭਿੰਨਤਾ/ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਨੇ ਮਨੁੱਖ ਜਾਤੀ ਦੇ ਵਿਕਾਸ ਅਤੇ ਭਲਾਈ ਵਿਚ । ਆਪਣੀ ਭੁਮਿਕਾ ਨਾ ਨਿਭਾਈ ਹੋਵੇ ।
(ਉ) ਪਰਿਸਥਿਤਿਕੀ ਕਦਰਾਂ-ਕੀਮਤਾਂ (Ecological Value).

- ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਨੇ ਮਨੁੱਖ ਜਾਤੀ ਨੂੰ ਆਕਸੀਜਨ ਦੀ ਉਤਪੱਤੀ, ਕਾਰਬਨ । ਡਾਈਆਕਸਾਈਡ ਦੀ ਮਾਤਰਾ ਨੂੰ ਘਟਾਉਣ, ਜਲ-ਚੱਕਰ ਨੂੰ ਕਾਇਮ ਰੱਖਣ ਅਤੇ ਥੋਂ ਦੇ ਸੁਰੱਖਿਅਣ ਵਰਗੀਆਂ ਵਾਤਾਵਰਣੀ ਸੇਵਾਵਾਂ ਕਿਸੇ ਪ੍ਰਕਾਰ ਦੇ ਖ਼ਰਚੇ ਤੋਂ ਬਿਨਾਂ ਉਪਲੱਬਧ ਅਲੋਪ ਹੋਇਆ ਪੰਛੀ । ਕਰਵਾਈਆਂ ਹਨ ।
- ਅਸੀਂ ਜਾਣਦੇ ਹਾਂ ਕਿ ਇਕ ਜਾਤੀ ਦੀ ਉੱਤਰਜੀਵਤਾ (Survival) ਕਈ ਹੋਰ ਕਾਰਨਾਂ ਕਰਕੇ ਦੂਸਰੀਆਂ ਜਾਤੀਆਂ ਉੱਤੇ ਨਿਰਭਰ ਕਰਦੀ ਹੈ । ਕਿਸੇ ਇਕ ਜਾਤੀ ਦੇ ਅਲੋਪ ਹੋ ਜਾਣ ਦੇ ਫਲਸਰੂਪ ਕਿਸੇ ਵੀ ਪ੍ਰਬੰਧ (System) ਜਾਂ ਪ੍ਰਣਾਲੀ ਦੇ ਵਿਚ ਤਬਦੀਲੀ ਆ ਸਕਦੀ ਹੈ ਜਾਂ ਇਹ ਪ੍ਰਣਾਲੀ ਖ਼ਤਮ ਹੋ ਸਕਦੀ ਹੈ । ਇਸ ਕਥਨ ਨੂੰ ਡੋ-ਡੋ (Do-Do) ਪੰਛੀ ਅਤੇ ਕੈਲਵੇਰਸ (Calvaris) ਦਾ ਉਦਾਹਰਨ ਦੇ ਕੇ ਸਪੱਸ਼ਟ ਕੀਤਾ ਜਾ ਸਕਦਾ ਹੈ ।
(ਅ) ਅਨੁਵੰਸ਼ਿਕ ਕਦਰਾਂ-ਕੀਮਤਾਂ (Genetic Values) – ਪ੍ਰਾਚੀਨ ਕਾਲ ਤੋਂ ਕਿਸਾਨ ਬਨਾਉਟੀ ਚੋਣ (Artificial selection) ਦੁਆਰਾ ਫ਼ਸਲਾਂ ਅਤੇ ਘਰੇਲੂ ਜਾਨਵਰਾਂ ਦੀਆਂ ਚੰਗੀਆਂ ਕਿਸਮਾਂ ਦਾ ਵਿਕਾਸ ਕਰ ਰਹੇ ਹਨ । ਅਜਿਹਾ ਕਰਨ ਦੇ ਵਾਸਤੇ ਉਹ ਜੰਗਲੀ ਕਿਸਮਾਂ ਦੇ ਲਾਹੇਵੰਦ ਜੀਨਾਂ ਦੀ ਚੋਣ ਕਰਕੇ ਘਰੇਲੂ ਕੀਤੀਆਂ ਗਈਆਂ (Domesticated) Histni nied ਦਾਖ਼ਿਲ ਕਰਦੇ ਹਨ । ਜੀਨਾਂ ਦੇ ਇਸ ਵਟਾਂਦਰੇ (Gene exchange) ਦੇ ਸਿੱਟੇ ਵਜੋਂ ਅਸੀਂ ਵਧੇਰੇ ਉਪਜ ਦੇਣ ਵਾਲੀਆਂ ਅਤੇ ਬੀਮਾਰੀਆਂ ਦਾ ਮੁਕਾਬਲਾ ਕਰ ਸਕਣ ਵਾਲੀਆਂ ਜਾਤੀਆਂ ਨੂੰ ਵਿਕਸਿਤ ਕਰਨ ਵਿਚ ਸਫਲ ਹੋਏ ਹਾਂ । ਭਾਰਤ ਵਿਚ ਪਾਈ ਜਾਂਦੀ ਧਾਨ ਦੀ ਜੰਗਲੀ ਕਿਸਮ (Wild variety of Orya sativa) ਤੋਂ ਜੀਨਜ਼ ਪ੍ਰਾਪਤ ਕਰਕੇ ਧਾਨ ਦੀ ਅਜਿਹੀ ਕਿਸਮ, ਜਿਸਦੀ ਖੇਤੀ ਏਸ਼ੀਆ ਵਿਚ ਕੀਤੀ ਜਾਂਦੀ ਹੈ, ਚਾਰ ਕਿਸਮਾਂ ਦੀਆਂ ਬਿਮਾਰੀਆਂ ਤੋਂ ਮੁਕਤ ਹੈ । ਖੇਤੀ ਵਿਗਿਆਨੀਆਂ ਦੇ ਲਈ ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਇਕ ਪ੍ਰਕਾਰ ਦਾ ਜੀਨ ਸੰਮ੍ਹਾਲਿਆਂ ਜੀਨ ਬੈਂਕ (Gene bank) ਹੈ, ਜਿਸ ਦੀ ਵਰਤੋਂ ਸੁਧਰੀਆਂ ਹੋਈਆਂ ਕਿਸਮਾਂ ਦਾ ਵਿਕਾਸ ਕਰਨ ਲਈ ਕੀਤੀ ਜਾਂਦੀ ਹੈ ।

(ੲ) ਆਰਥਿਕ ਕਦਰਾਂ-ਕੀਮਤਾਂ (Economic Values) – ਜੀਵ-ਮੰਡਲ ਦੀ ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਦਾ ਮਨੁੱਖ ਜਾਤੀ ਦੀ ਆਰਥਿਕਤਾ ਨਾਲ ਸਿੱਧਾ ਜਾਂ ਅਸਿੱਧਾ ਸੰਬੰਧ ਹੈ । ਵਣਾਂ ਦੇ ਵਾਸੀ ਆਪਣੀਆਂ ਜ਼ਰੂਰਤਾਂ, ਜਿਵੇਂ-ਲੱਕੜੀ, ਭੋਜਨ, ਤਨ ਢੱਕਣ ਲਈ ਪਦਾਰਥ, ਭਵਨ ਨਿਰਮਾਣ ਲਈ ਵਰਤੀਆਂ ਜਾਣ ਵਾਲੀਆਂ ਚੀਜ਼ਾਂ, ਪਰੰਪਰਾਗਤ ਦਵਾਈਆਂ ਅਤੇ ਫਲਾਂ ਲਈ ਵਣਾਂ ’ਤੇ ਨਿਰਭਰ ਕਰਦੇ ਹਨ | ਖਾਧ ਪਦਾਰਥਾਂ ਦੀ ਪ੍ਰਾਪਤੀ ਲਈ ਮਛੇਰੇ ਮੱਛੀਆਂ ਅਤੇ ਜਲ ਜੀਵਾਂ ਉੱਪਰ ਨਿਰਭਰ ਕਰਦੇ ਹਨ । ਇਕ ਰੁੱਖ ਤੋਂ ਆਰਥਿਕ ਮਹੱਤਤਾ ਵਾਲੇ ਅਨੇਕਾਂ ਪਦਾਰਥ ਪ੍ਰਾਪਤ ਹੁੰਦੇ ਹਨ ਅਤੇ ਇਹ ਕਈ ਪ੍ਰਕਾਰ ਦੇ ਜੰਤੂਆਂ ਦੇ ਨਿਵਾਸ ਸਥਾਨਾਂ ਵਜੋਂ ਭੂਮਿਕਾ ਨਿਭਾਉਂਦੇ ਹਨ । ਇਹ ਰੁੱਖ ਤੋਂ ਅਤੇ ਪਾਣੀ ਦੇ ਸੁਰੱਖਿਅਣ ਵਿਚ ਵੀ ਆਪਣਾ ਯੋਗਦਾਨ ਪਾਉਂਦਾ ਹੈ ।
(ਸ) ਵਿਗਿਆਨਿਕ ਕਦਰਾਂ-ਕੀਮਤਾਂ (Scientific values) – ਵੱਖ-ਵੱਖ ਸਮਯੋਗਤਾ ਵਰਗ ਵਾਲੇ ਵਿਗਿਆਨੀਆਂ ਲਈ ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਦੀਆਂ ਕਦਰਾਂਕੀਮਤਾਂ ਅਲੱਗ-ਅਲੱਗ ਕਿਸਮਾਂ ਦੀਆਂ ਹਨ । ਜਣਨਿਕ ਪਦਾਰਥਾਂ ਦੀ ਵਰਤੋਂ ਕਰਕੇ ਬਾਇਓ-ਤਕਨੋਲੋਜਿਸਟ ਵਧੇਰੇ ਉਪਜ ਦੇਣ ਵਾਲੀਆਂ ਅਤੇ ਹਾਨੀਕਾਰਕ ਕੀਟਾਂ ਦਾ ਟਾਕਰਾ ਕਰ ਸਕਣ ਵਾਲੇ ਪੌਦਿਆਂ ਅਤੇ ਜੰਤੂਆਂ ਦਾ ਵਿਕਾਸ ਕਰ ਸਕੇ ਹਨ । ਵਧੇਰੇ ਝਾੜ ਦੇਣ ਵਾਲੀ ਅਤੇ ਕੀਟਾਂ ਦਾ ਟਾਕਰਾ ਕਰਨ ਵਾਲੀ ਬੀਟੀ-ਕਪਾਹ (Bt-cotton) ਦਾ ਬਾਇਓਟੈਕਨੋਲੋਜਿਸਟਾਂ ਵੱਲੋਂ ਕੀਤਾ ਗਿਆ ਵਿਕਾਸ ਇਸ ਦਾ ਇਕ ਉਦਾਹਰਨ ਹੈ ।

(ਹ) ਦਵਾਈਆਂ ਸੰਬੰਧੀ ਕਦਰਾਂ-ਕੀਮਤਾਂ (Medicinal Value) – ਪੌਦਿਆਂ ਤੋਂ ਬਹੁਤ ਕੀਮਤੀ ਦਵਾਈਆਂ ਪ੍ਰਾਪਤ ਕੀਤੀਆਂ ਜਾਂਦੀਆਂ ਹਨ । ਅਜਿਹੀਆਂ ਕੁੱਝ ਦਵਾਈਆਂ ਦੇ ਉਦਾਹਰਨ ਹੇਠਾਂ ਦਿੱਤੇ ਜਾਂਦੇ ਹਨ ।
- ਕੁਕੀਨ (Quinine) – ਇਸ ਦਵਾਈ ਦਾ ਸਰੋਤ ਪੀਲਾ ਸਿਨਕੋਨਾ (Yellow (Cinchona) ਰੁੱਖ ਹੈ ਅਤੇ ਇਸ ਦਵਾਈ ਦੀ ਵਰਤੋਂ ਮਲੇਰੀਆ ਤਾਪ ਦੇ ਇਲਾਜ ਲਈ ਕੀਤੀ ਜਾਂਦੀ ਹੈ ।
- ਪੈਨਸੀਲੀਨ (Penicillin) – ਇਹ ਸਭ ਤੋਂ ਪਰਾਣਾ ਐਂਟੀਬਾਇਓਟਿਕ (Anti-biotic) ਹੈ ਅਤੇ ਇਹ ਦਵਾਈ ਪੈਨੀਸੀਲੀਅਮ (Penicillium) ਨਾਂ ਦੀ ਉੱਲੀ (Fungus) ਤੋਂ ਤਿਆਰ ਕੀਤੀ ਜਾਂਦੀ ਹੈ ।
- ਡਿਜੀਟੋਕਸਿਨ (Digitoxin) – ਦਿਲ ਦੀਆਂ ਬੀਮਾਰੀਆਂ ਦੇ ਇਲਾਜ ਲਈ ਵਰਤੀ ਜਾਂਦੀ ਇਹ ਦਵਾਈ ਆਮ ਮਿਲਣ ਵਾਲੇ ਫੋਕਸਗਲੋਵ (Foxglove) ਪੌਦੇ ਤੋਂ ਤਿਆਰ ਕੀਤੀ ਜਾਂਦੀ ਹੈ ।
- ਤੁਲਸੀ (Tulsi) – ਪ੍ਰਾਚੀਨ ਕਾਲ ਤੋਂ ਤੁਲਸੀ ਦੀ ਵਰਤੋਂ ਤਾਪ ਨਿਵਾਰਨ ਅਤੇ ਖ਼ਰਾਬ ਗਲੇ ਨੂੰ ਠੀਕ ਕਰਨ ਦੇ ਲਈ ਕੀਤੀ ਜਾ ਰਹੀ ਹੈ ।
- ਟੈਕਸੋਲ (Taxol) – ਇਹ ਦਵਾਈ ਥਿਊ ਪੌਦੇ (Yew plant, Taxus spp) ਦੀ ਛਿਲੜ/ਛਿਲਕੇ (Bark) ਤੋਂ ਪ੍ਰਾਪਤ ਹੁੰਦੀ ਹੈ ਅਤੇ ਇਹ ਕੈਂਸਰ ਦੇ ਇਲਾਜ ਲਈ ਵਰਤੀ ਜਾਂਦੀ ਹੈ ।

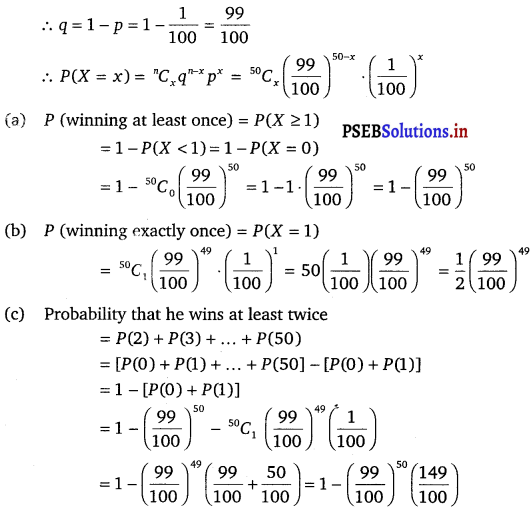
(ਕ) ਸਮਾਜਿਕ ਕਦਰਾਂ (Social values) – ਪਰੰਪਰਾਗਤ ਸਮੁਦਾਇ ਨਾਲ ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਦਾ ਸੰਬੰਧ ਬੜਾ ਪੁਰਾਣਾ ਹੈ ਅਤੇ ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਨੂੰ ਇਹ ਸਮੁਦਾਇ ਆਪਣੀ ਜ਼ਿੰਦਗੀ ਦਾ ਇਕ ਅੰਗ ਮੰਨਦੇ ਹਨ ਅਤੇ ਆਪਸੀ ਉਤਰਜੀਵਤਾ ਲਈ ਬਹੁਤ ਜ਼ਰੂਰੀ ਸਮਝਦੇ ਹਨ । ਉਹ ਕਈ ਫ਼ਸਲਾਂ ਪਰੰਪਰਾਗਤ ਤਰੀਕਿਆਂ ਨਾਲ ਕਾਸ਼ਤ ਕਰਦੇ ਹਨ ਤਾਂ ਜੋ ਫ਼ਸਲਾਂ ਫੇਲ ਨਾ ਹੋਣ ।
![]()
(ਖ) ਸੱਭਿਆਚਾਰਕ ਕਦਰਾਂ-ਕੀਮਤਾਂ (Cultural Values) – ਕਈ ਸਦੀਆਂ ਤੋਂ ਪ੍ਰਾਣੀ ਅਤੇ ਪੌਦੇ ਸਾਡੀ ਸਭਿਅਤਾ ਦਾ ਇਕ ਵਿਸ਼ੇਸ਼ ਅੰਗ ਬਣ ਚੁੱਕੇ ਹਨ, ਜਿਵੇਂ ਕਿ ਤੁਲਸੀ, ਪਿੱਪਲ । ਇਨ੍ਹਾਂ ਦੀ ਕਈ ਲੋਕ ਪੂਜਾ ਵੀ ਕਰਦੇ ਹਨ | ਕਈ ਪੰਛੀਆਂ ਅਤੇ ਸੱਪਾਂ ਨੂੰ ਵੀ ਲੋਕ ਪੁਜਦੇ ਹਨ । ਪੰਜਾਬ, ਹਰਿਆਣਾ, ਅਤੇ ਰਾਜਸਥਾਨ ਵਿਚ ਸੱਪਾਂ ਦਾ ਪੂਜਨ ਕਰਨ ਦੇ ਮੰਤਵ ਨਾਲ ਨਾਗ ਪੰਚਮੀ ਦਾ ਉਤਸਵ ਬੜੀ ਸ਼ਰਧਾ ਅਤੇ ਧੂਮ ਧਾਮ ਨਾਲ ਮਨਾਇਆ ਜਾਂਦਾ ਹੈ । ਸਾਡੇ ਦੇਸ਼ ਵਿਚ ਕਈ ਥਾਂਵਾਂ ‘ਤੇ ਛੋਟੇ ਵਣਾਂ (Groves) ਜਾਂ ਝਿੜੀਆਂ ਨੂੰ ਲੋਕ ਪਵਿੱਤਰ ਮੰਨਦੇ ਹਨ ।

(ਗ) ਸੁਹਜਾਤਮਕ ਅਤੇ ਮਨੋਰੰਜਨ ਸੰਬੰਧੀ ਕਦਰਾਂ-ਕੀਮਤਾਂ (Aesthetic and Recreational Values) ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਦੀ ਸੁਹਜਾਤਮਕ ਅਤੇ ਮਨੋਰੰਜਨ ਦੇ ਪੱਖ ਤੋਂ ਬੜੀ ਮਹੱਤਤਾ ਹੈ । ਲੋਕਾਂ ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਦੀ ਰੰਗਤ, ਆਦਿ ਨੂੰ ਵੇਖ ਕੇ ਬੜੇ ਖੁਸ਼ ਹੁੰਦੇ ਹਨ । ਨੱਚਦਾ ਹੋਇਆ ਮੋਰ ਸਭ ਨੂੰ ਆਪਣੇ ਵੱਲ ਖਿੱਚ ਲੈਂਦਾ ਹੈ ।
ਸੈਰ ਸਪਾਟਾ ਕਰਨ ਵਿਚ ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਦੀ ਬੜੀ ਮਹੱਤਤਾ ਹੈ । ਸੈਰ ਸਪਾਟੇ ਲਈ ਰਮਣੀਕ ਪਹਾੜ, ਝੀਲਾਂ ਅਤੇ ਇਤਿਹਾਸਿਕ ਪ੍ਰਸਿੱਧੀ ਵਾਲੇ ਅਤੇ ਧਰਮ ਨਾਲ ਸੰਬੰਧਿਤ ਸਥਾਨ ਖਿੱਚ ਦੇ ਕੇਂਦਰ ਹਨ । ਸੈਰ ਸਪਾਟਾ ਕਰਨ ਨਾਲ ਲੋਕਾਂ ਨੂੰ ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਦੀ ਮਹੱਤਤਾ ਬਾਰੇ ਕਾਫੀ ਸੁਨੇਹ ਪੈਦਾ ਹੋ ਜਾਂਦਾ ਹੈ ।

ਨੈਤਿਕ ਮਹੱਤਤਾ (Ethical Value) – ਧਰਤੀ ‘ਤੇ ਮੌਜੂਦ ਜੀਵਨ ਪਾਣੀ ਅਤੇ ਪੌਦੇ ਦੀ ਵਜ਼ਾ ਜੀਵ ਵਿਕਾਸ (Organic evolution) ਹੈ ਅਤੇ ਇਹ ਵਿਕਾਸ ਦੇ ਹੋਣ ਵਿਚ ਲੱਖਾਂ ਸਾਲਾਂ ਦਾ ਸਮਾਂ ਲਗਿਆ । ਇਸ ਲਈ ਸਾਡਾ ਸਾਰਿਆਂ ਦਾ ਇਹ ਫ਼ਰਜ਼ ਬਣਦਾ ਹੈ ਕਿ ਅਸੀਂ ਇਸ ਜੀਵਨ ਨੂੰ ਸੁਰੱਖਿਅਤ ਰੱਖੀਏ ਤਾਂ ਜੋ ਸਾਡੀਆਂ ਆਉਣ ਵਾਲੀਆਂ ਪੀੜੀਆਂ ਵੀ ਸਾਡੀ ਤਰ੍ਹਾਂ ਇਨ੍ਹਾਂ ਨੂੰ ਮਾਣ ਸਕਣ ।
ਪ੍ਰਸ਼ਨ 2.
ਪਰਿਸਥਿਤਿਕ ਵਿਭਿੰਨਤਾ/ਜੀਵ ਅਨੇਕਰੂਪਤਾ ਦੇ ਤਿੰਨ ਪੱਧਰ ਕਿਹੜੇ-ਕਿਹੜੇ ਹਨ ?
ਉੱਤਰ-
ਪਦਮੀ ਤਰਤੀਬ (Hierarchial level) ਦੀ ਪੱਧਰ ‘ਤੇ ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ ਨੂੰ ਤਿੰਨ ਸ਼੍ਰੇਣੀਆਂ ਵਿਚ ਵੰਡਿਆ ਗਿਆ ਹੈ ਅਤੇ ਇਹ ਹਨ
(i) ਜਣਨਿਕ ਵਿਭਿੰਨਤਾ/ਜੀਵ ਅਨੇਕਰੂਪਤਾ,
(ii) ਜਾਤੀ ਵਿਭਿੰਨਤਾ /ਜਾਤੀ ਅਨੇਕਰੂਪਤਾ ਅਤੇ
(iii) ਪਰਿਆਵਰਣ ਵਿਭਿੰਨਤਾ ।
(i) ਜਣਨਿਕ ਵਿਭਿੰਨਤਾ/ਜੀਵ ਅਨੇਕਰੂਪਤਾ (Genetic diversity) – ਕਿਸੇ ਜਾਤੀ ਵਿਚ ਮੌਜੂਦ ਜੀਨਾਂ ਵਿਚ ਪਾਈਆਂ ਜਾਂਦੀਆਂ ਵਿਭਿੰਨਤਾਵਾਂ ਦਾ ਉਲੇਖ ਜਣਨਿਕ ਅਨੇਕਰੂਪਤਾ ਜਣਨਿਕ ਵਿਭਿੰਨਤਾਵਾਂ) ਕਰਦਾ ਹੈ । ਮੋਸੋਮਜ਼ (Chromosomes) ਜੀਨਾਂ ਵਿਚ ਪੈਦਾ ਹੋਣ ਵਾਲੀ ਅਨੇਕਰੂਪਤਾ ਦੇ ਕਾਰਨ ਵੱਖ-ਵੱਖ ਤਰ੍ਹਾਂ ਦੀਆਂ ਕਿਸਮਾਂ ਅਤੇ ਉਪ-ਜਾਤੀਆਂ (Subspecies) ਅਤੇ ਜਾਤੀਆਂ ਪੈਦਾ ਹੋ ਸਕਦੀਆਂ ਹਨ ।
(ii) ਜਾਤੀ ਵਿਭਿੰਨਤਾ /ਜਾਤੀ ਅਨੇਕਰੂਪਤਾ (Species diversity) – ਕਿਸੇ ਖਿੱਤੇ (Region) ਵਿਚ ਮਿਲਣ ਵਾਲੇ ਪੌਦਿਆਂ ਅਤੇ ਜੰਤੂਆਂ ਦੀ ਸੰਖਿਆ ਦੇ ਉਲੇਖ ਨੂੰ ਜਾਤੀ ਵਿਭਿੰਨਤਾ ਕਹਿੰਦੇ ਹਨ । ਵਿਗਿਆਨੀ ਧਰਤੀ ਉੱਤੇ ਮੌਜੂਦ 1.8 ਮਿਲੀਅਨ ਜੀਵਤ ਜਾਤੀਆਂ ਦੀ ਪਛਾਣ ਕਰ ਚੁੱਕੇ ਹਨ ਅਤੇ ਧਰਤੀ ਉੱਪਰ ਮੌਜੂਦ ਸਜੀਵਾਂ ਦਾ ਕੇਵਲ ਇਕ ਮਾਮੂਲੀ ਜਿਹਾ ਅੰਸ਼ ਹੀ ਹੈ । ਦੁਨੀਆਂ ਭਰ ਵਿਚ ਖੋਜਾਂ ਦੁਆਰਾ ਵਿਗਿਆਨੀ ਨਵੀਆਂ-ਨਵੀਆਂ ਜਾਤੀਆਂ ਲੱਭ ਰਹੇ ਹਨ । ਜਾਤੀ ਵਿਭਿੰਨਤਾ ਨੂੰ ਕੁਦਰਤੀ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਜਾਂ ਜ਼ਰਾਇਤੀ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਵਿਚ ਵੇਖਿਆ ਜਾ ਸਕਦਾ ਹੈ ।
(iii) ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਬੰਧਾਂ ਵਿਚਲੀ ਜੈਵਿਕ ਵਿਭਿੰਨਤਾ/ਜੀਵ ਅਨੇਕਰੂਪਤਾ (Eco-system diversity) – ਕਿਸੇ ਸਮੁਦਾਇ ਅਤੇ ਇਸ ਦੇ ਵਾਤਾਵਰਣ ਦੇ ਵਿਚਾਲੇ ਹੋਣ ਵਾਲੀਆਂ ਅੰਤਰਕਿਰਿਆਵਾਂ ਦੇ ਕਾਰਨ ਰਚੀ ਗਈ ਰਚਨਾ ਨੂੰ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਬੰਧਾਂ ਵਿਚਲੀ ਅਨੇਕਰੂਪਤਾ ਆਖਦੇ ਹਨ । ਧਰਤੀ ਉੱਪਰ ਪਾਈਆਂ ਜਾਣ ਵਾਲੀਆਂ ਪਰਿਆਵਰਣ ਅਨੇਕਰੂਪਤਾਂਵਾਂ ਵਿਚ ਦਰਿਆ, ਝੀਲਾਂ ਅਤੇ ਸਾਗਰ ਸ਼ਾਮਿਲ ਹਨ । ਹਰੇਕ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਬੰਧਾਂ ਵਿਚਲੀ ਅਨੇਕਰੂਪਤਾ ਵਿਚ ਨਿਵਾਸ ਸਥਾਨ ਤੇ ਰਹਿਣ ਵਾਲੀਆਂ ਜਾਤੀਆਂ ਦੇ ਆਪਸੀ ਸੰਬੰਧਾਂ ਦੇ ਪੂਰਕ ਮੌਜੂਦ ਹਨ। ਜੇਕਰ ਕਿਸੇ ਵਾਤਾਵਰਣ ਵਿਚ ਮਨੁੱਖੀ ਦਖ਼ਲ-ਅੰਦਾਜ਼ੀ ਨਾ ਹੁੰਦੀ ਹੋਵੇ ਜਾਂ ਨਾ ਕੀਤੀ ਗਈ ਹੋਵੇ, ਤਾਂ ਅਜਿਹੇ ਵਾਤਾਵਰਣ ਨੂੰ ਕੁਦਰਤੀ ਵਾਤਾਵਰਣ ਕਹਿੰਦੇ ਹਨ । ਜੇਕਰ ਵਾਤਾਵਰਣ ਨੂੰ ਕਿਸੇ ਹੋਰ ਪ੍ਰਕਾਰ ਦੀ ਵਰਤੋਂ ਕਰਨ ਦੇ ਲਈ ਬਦਲਿਆ ਜਾਵੇ ਤਾਂ ਅਜਿਹਾ ਵਾਤਾਵਰਣ ਸੋਧਿਆ ਹੋਇਆ (Modified) ਪਰਿਆਵਰਣ ਅਖਵਾਉਂਦਾ ਹੈ ।
ਪ੍ਰਸ਼ਨ 3.
ਕੁਦਰਤ ਵਿੱਚ ਸੰਤੁਲਨ ਤੋਂ ਕੀ ਭਾਵ ਹੈ ਅਤੇ ਇਹ ਕਿਵੇਂ ਬਣਾਇਆ ਜਾਂਦਾ ਹੈ ?
ਜਾਂ
‘ਕੁਦਰਤ ਵਿਚ ਸੰਤੁਲਨ’ ਉੱਤੇ ਇਕ ਨੋਟ ਲਿਖੋ
ਉੱਤਰ-
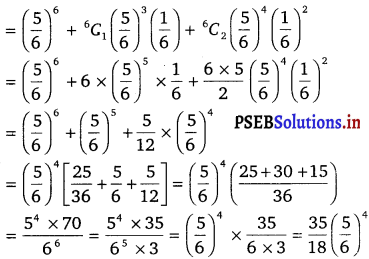
ਕੁਦਰਤ ਵਿੱਚ ਸੰਤੁਲਨ (Balance in Nature) – ਜਦੋਂ ਪ੍ਰਕਿਰਤੀ ਵੱਖ-ਵੱਖ ਕਿਸਮਾਂ ਦੀਆਂ ਚੀਜ਼ਾਂ ਇੱਕ ਸਮਾਨ ਸੰਖਿਆ ਵਿੱਚ ਮੌਜੂਦ ਹੋਣ, ਤਾਂ ਅਜਿਹੀ ਅਵਸਥਾ ਨੂੰ ਕੁਦਰਤ ਵਿੱਚ ਸੰਤੁਲਨ ਆਖਦੇ ਹਨ । ਪ੍ਰਿਥਵੀ ‘ਤੇ ਮੌਜੂਦ ਹਰੇਕ ਪ੍ਰਣਾਲੀ ਸੰਤੁਲਨ ਦੀ ਅਵਸਥਾ ਵਿੱਚ ਹੈ ਅਤੇ ਇਸ ਸੰਤੁਲਨ ਵਿੱਚ ਆਈ ਕੋਈ ਵੀ ਤਬਦੀਲੀ ਕੁਦਰਤ ਵਿੱਚ ਅਸੰਤੁਲਨ ਪੈਦਾ ਕਰ ਦਿੰਦੀ ਹੈ ।
ਕੁਦਰਤ ਵਿੱਚ ਸੰਤੁਲਨ ਨੂੰ ਕਾਇਮ ਕਰਨ ਤੋਂ ਪਹਿਲਾਂ ਕੁਦਰਤ ਦੇ ਅੰਸ਼ਾਂ ਬਾਰੇ ਜਾਣਕਾਰੀ ਪ੍ਰਾਪਤ ਕਰ ਲੈਣੀ ਬਹੁਤ ਜ਼ਰੂਰੀ ਹੈ । ਕੁਦਰਤ ਦੇ ਜੈਵਿਕ ਅਤੇ ਅਜੈਵਿਕ ਦੋ ਹੀ ਅੰਸ਼ ਹਨ ।
ਜੈਵਿਕ ਅੰਸ਼ (Biotic Components) – ਇਨ੍ਹਾਂ ਅੰਗਾਂ ਵਿੱਚ ਸਾਰੇ ਦੇ ਸਾਰੇ ਜੀਵ ਜਿਵੇਂ ਕਿ ਸਾਰੇ ਪੌਦੇ, ਸਾਰੇ ਪ੍ਰਾਣੀ, ਪੰਛੀ, ਬੈਕਟੀਰੀਆ ਆਦਿ ਸ਼ਾਮਿਲ ਹਨ। | ਜੇਕਰ ਪਰਿਸਥਿਤੀ ਵਿੱਚ ਮਨੁੱਖੀ ਛੇੜ-ਛਾੜ ਨਾ ਹੋਵੇ ਤਾਂ ਕੁਦਰਤ ਵਿੱਚ ਸੰਤੁਲਨ ਬਣਿਆ ਰਹਿ ਸਕਦਾ ਹੈ, ਪਰ ਵਾਤਾਵਰਣ ਦੇ ਅੰਸ਼ਾਂ ਨਾਲ ਮਨੁੱਖੀ ਛੇੜ-ਛਾੜ ਦਾ ਹੀ ਸਿੱਟਾ ਹੈ ਕਿ ਵਾਤਾਵਰਣ ਵਿੱਚ ਅਸੰਤੁਲਨ ਪੈਦਾ ਹੋ ਗਿਆ ਅਤੇ ਅਜੇ ਵੀ ਹੋ ਰਿਹਾ ਹੈ ।
ਅਜੈਵਿਕ ਅੰਸ਼ (Abiotic Components) – ਇਸ ਵਿੱਚ ਸਾਰੀਆਂ ਨਿਰਜੀਵ ਵਸਤਾਂ ਜਿਵੇਂ ਕਿ ਮਿੱਟੀ, ਪਾਣੀ, ਖਣਿਜ, ਹਵਾ, ਪ੍ਰਕਾਸ਼ ਅਤੇ ਸਿੱਲ੍ਹ ਆਦਿ ਸ਼ਾਮਿਲ ਹਨ । ਇਨ੍ਹਾਂ ਅੰਸ਼ਾਂ ਵਿੱਚੋਂ ਕਿਸੇ ਇਕ ਅੰਸ਼ ਵਿਚ ਕਿਸੇ ਵੀ ਵਜ਼ਾ ਕਰਕੇ ਆਇਆ ਪਰਿਵਰਤਨ ਵਾਤਾਵਰਣ ਵਿੱਚ ਅਸੰਤੁਲਨ ਪੈਦਾ ਕਰ ਦਿੰਦਾ ਹੈ ।
ਕੁਦਰਤ ਵਿੱਚ ਸੰਤੁਲਨ ਨੂੰ ਬਣਾਈ ਰੱਖਣ ਦੇ ਲਈ ਜੈਵਿਕ ਅਤੇ ਅਜੈਵਿਕ ਅੰਸ਼ਾਂ ਵਿਚਾਲੇ ਸੰਤੁਲਨ ਨੂੰ ਬਣਾਈ ਰੱਖਣਾ ਬੜਾ ਜ਼ਰੂਰੀ ਹੈ । ਹਰੇਕ ਪਰਿਸਥਿਤਿਕ ਪ੍ਰਣਾਲੀ ਦੇ ਜੀਵ ਭਾਵੇਂ ਸਵੈ-ਨਿਰਭਰ ਹੀ ਹਨ, ਪਰ ਇਹ ਇੱਕ-ਦੂਜੇ ਉੱਤੇ ਨਿਰਭਰ ਵੀ ਕਰਦੇ ਹਨ ਅਤੇ ਇਨ੍ਹਾਂ ਦੀ ਇਹ ਆਪਸੀ ਨਿਰਭਰਤਾ ਹੀ ਸੰਤੁਲਨ ਨੂੰ ਬਣੇ ਰਹਿਣ ਵਿੱਚ ਸਹਾਈ ਹੁੰਦੀ ਹੈ ।
ਵਾਤਾਵਰਣ ਵਿੱਚ ਅਸੰਤੁਲਨ ਦਾ ਸਭ ਤੋਂ ਵੱਡਾ ਕਾਰਨ ਵਸੋਂ ਵਿੱਚ ਹੋ ਰਿਹਾ ਵਾਧਾ ਹੈ । ਜੇਕਰ ਅਸੀਂ ਆਪਣੇ ਲਈ ਅਤੇ ਆਪਣੀਆਂ ਆਉਣ ਵਾਲੀਆਂ ਪੀੜੀਆਂ ਦਾ ਉੱਜਲ ਭਵਿੱਖ ਲੋਚਦੇ ਹਾਂ ਤਾਂ ਸਾਨੂੰ ਵਾਤਾਵਰਣ ਵਿੱਚ ਬੇਲੋੜੀ ਛੇੜ-ਛਾੜ ਅਤੇ ਸ਼ੋਸ਼ਣ ਨੂੰ ਬੰਦ ਕਰਨਾ ਹੋਵੇਗਾ, ਨਹੀਂ ਤਾਂ ਇਸ ਦੇ ਬੜੇ ਗੰਭੀਰ ਸਿੱਟੇ ਨਿਕਲ ਸਕਦੇ ਹਨ ।
![]()
ਪ੍ਰਸ਼ਨ 4.
ਕੁਦਰਤ ਵਿੱਚ ਮਿਲਣ ਵਾਲੇ ਸੀਮਿਤ ਸਰੋਤਾਂ ਦੇ ਨਾਂ ਲਿਖੋ । ਪਾਣੀ ਦੀ ਸਾਂਭਸੰਭਾਲ ਕਿਵੇਂ ਕੀਤੀ ਜਾ ਸਕਦੀ ਹੈ ? .
ਉੱਤਰ-
ਕੁਦਰਤ ਵਿੱਚ ਮਿਲਣ ਵਾਲੇ ਸੀਮਿਤ ਸਰੋਤ :
- ਮਿੱਟੀ ਤੋਂ),
- ਪਾਣੀ,
- ਖਣਿਜ,
- ਪਥਰਾਟ ਈਂਧਨ ।
ਪਾਣੀ ਦੀ ਸਾਂਭ-ਸੰਭਾਲ ਦੇ ਤਰੀਕੇ – ਪਾਣੀ ਨਾ ਕੇਵਲ ਮਨੁੱਖੀ ਜੀਵਨ ਲਈ ਹੀ ਜ਼ਰੂਰੀ ਹੈ, ਸਗੋਂ ਇਹ ਪਾਣੀਆਂ ਅਤੇ ਪੌਦਿਆਂ ਦੇ ਲਈ ਵੀ ਜ਼ਰੂਰੀ ਹੈ । ਜੀਵਨ ਦੀ ਉਤਪੱਤੀ ਪਾਣੀ ਤੋਂ ਹੀ ਹੋਈ ਹੈ ਅਤੇ ਪਾਣੀ ਕਾਰਨ ਹੀ ਕਾਇਮ ਹੈ । ਧਰਤੀ ਦਾ 70.8% ਭਾਗ ਪਾਣੀ ਨੇ ਮੱਲਿਆ ਹੋਇਆ ਹੈ ਅਤੇ ਇਸ ਵਿੱਚੋਂ ਸਮੁੰਦਰ ਦਾ 97% ਹਿੱਸਾ ਹੈ । ਜਿਹੜਾ ਪਾਣੀ ਮਨੁੱਖ ਜਾਤੀ ਦੇ ਵੱਖ-ਵੱਖ ਕੰਮਾਂ ਲਈ ਉਪਲੱਬਧ ਹੈ, ਉਸ ਦੀ ਮਾਤਰਾ ਕੇਵਲ 1.0% ਹੀ ਹੈ ।2% ਪਾਣੀ ਹਿਮ ਟੋਪੀਆਂ ਅਤੇ ਹਿਮ ਨਦੀਆਂ ਵਿਚ ਕੈਦ ਹੈ ।
ਜਿਸ ਬੇਰਹਿਮੀ ਨਾਲ ਪਾਣੀ ਦੀ ਵਰਤੋਂ ਕੀਤੀ ਜਾ ਰਹੀ ਹੈ, ਇਸ ਨੇ ਇਹ ਡਰ ਪੈਦਾ ਕਰ ਦਿੱਤਾ ਹੈ ਕਿ ਪਾਣੀ ਬਹੁਤ ਛੇਤੀ ਦੁਰਲੱਭ ਹੋ ਜਾਵੇਗਾ ਅਤੇ ਭਵਿੱਖ ਵਿੱਚ ਵਿਸ਼ਵ ਯੁੱਧ ਦਾ ਮੁੱਖ ਕਾਰਨ ਪਾਣੀ ਹੀ ਹੋਵੇਗਾ ।
ਪਾਣੀ ਦੀ ਸਾਂਭ-ਸੰਭਾਲ – ਪਾਣੀ ਦੀ ਸਾਂਭ-ਸੰਭਾਲ ਬਹੁਤ ਜ਼ਰੂਰੀ ਹੋ ਗਈ ਹੈ । ਇਸ ਸੰਬੰਧ ਵਿੱਚ ਕੁੱਝ ਸੁਝਾਅ ਹੇਠਾਂ ਲਿਖੇ ਗਏ ਹਨ-
- ਪਾਣੀ ਦੀ ਵਰਤੋਂ ਬੜੀ ਸੋਚ ਅਤੇ ਸਮਝਦਾਰੀ ਨਾਲ ਕਰਨੀ ਚਾਹੀਦੀ ਹੈ ।
- ਪੀਣ ਵਾਲਾ ਪਾਣੀ ਸ਼ੁੱਧ ਅਤੇ ਕੀਟਾਣੂ ਰਹਿਤ ਹੋਣਾ ਚਾਹੀਦਾ ਹੈ ।
- ਸਿੰਚਾਈ ਕਰਨ ਸਮੇਂ ਵੀ ਪਾਣੀ ਦੀ ਵਰਤੋਂ ਬੜੇ ਧਿਆਨ ਨਾਲ ਕਰਨ ਦੀ ਜ਼ਰੂਰਤ ਹੈ ।
- ਘਰੇਲੂ ਨਲਕਿਆਂ ਦੀਆਂ ਟੂਟੀਆਂ ਖੁੱਲ੍ਹੀਆਂ ਨਹੀਂ ਰਹਿਣੀਆਂ ਚਾਹੀਦੀਆਂ । ਪਾਣੀ ਪ੍ਰਾਪਤ ਕਰਨ ਉਪਰੰਤ ਟੂਟੀਆਂ ਬੰਦ ਕਰ ਦੇਣੀਆਂ ਚਾਹੀਦੀਆਂ ਹਨ ।
- ਫੈਕਟਰੀਆਂ ਆਦਿ ਦੇ ਵਹਿਣਾਂ ਦਾ ਨਿਰੂਪਣ ਕਰਨ ਉਪਰੰਤ ਇਸ ਪਾਣੀ ਨੂੰ ਸਿੰਚਾਈ ਆਦਿ ਲਈ ਵਰਤਿਆ ਜਾ ਸਕਦਾ ਹੈ ।
- ਧਰਤੀ ਹੇਠਲੇ ਪਾਣੀ ਦੀ ਪੁਨਰ ਸੁਰਜੀਤੀ ਲਈ ਸੇਜਲ ਜ਼ਮੀਨਾਂ ਆਦਿ ਦੀ ਸੁਰੱਖਿਆ ਕੀਤੀ ਜਾਣੀ ਚਾਹੀਦੀ ਹੈ । ਇਸ ਕੰਮ ਦੇ ਲਈ ਮੀਂਹ ਦਾ ਪਾਣੀ ਇਕੱਠਾ ਕੀਤਾ ਜਾ ਸਕਦਾ ਹੈ ।
- ਭੂਮੀਗਤ ਪਾਣੀ ਦੀ ਪੁਨਰ ਸੁਰਜੀਤੀ ਲਈ ਛੱਪੜਾਂ ਦੀ ਸੰਭਾਲ ਕੀਤੀ ਜਾਣੀ ਚਾਹੀਦੀ ਹੈ ।
- ਮੀਂਹ ਆਦਿ ਦਾ ਵਾਧੂ ਪਾਣੀ, ਉਨ੍ਹਾਂ ਇਲਾਕਿਆਂ ਵਿੱਚ ਭੇਜੇ ਜਾਣ ਦੀਆਂ ਯੋਜਨਾਵਾਂ ਤਿਆਰ ਕੀਤੀਆਂ ਜਾਣੀਆਂ ਚਾਹੀਦੀਆਂ ਹਨ, ਜਿਨ੍ਹਾਂ ਇਲਾਕਿਆਂ ਵਿੱਚ ਇਸ ਦੀ ਥੁੜ੍ਹ ਹੈ ।
ਗੁਰਬਾਣੀ ਵਿੱਚ ਅੰਕਿਤ ਹੈ ‘ਪਹਿਲਾ ਪਾਣੀ ਜਿਉ ਹੈ ਜਿਤ ਹਰਿਆ ਸਭ ਕੋਇ ।’
ਇਸ ਤੁਕ ਤੋਂ ਪਾਣੀ ਦੀ ਮਹੱਤਤਾ ਸਪੱਸ਼ਟ ਹੋ ਜਾਂਦੀ ਹੈ ।
ਪ੍ਰਸ਼ਨ 5.
ਆਪਣੇ ਖੇਤਰ ਦੇ 5 ਦਵਾਈਆਂ ਦੇਣ ਵਾਲੇ ਪੌਦੇ ਲੱਭੋ । ਇਨ੍ਹਾਂ ਨਾਲ ਕਿਸ ਬਿਮਾਰੀ ਦਾ ਉਪਚਾਰ ਕੀਤਾ ਜਾਂਦਾ ਹੈ ? ਪੌਦੇ ਦੇ ਕਿਹੜੇ ਭਾਗ ਦੀ ਵਰਤੋਂ ਕੀਤੀ ਜਾਂਦੀ ਹੈ । ਆਯੁਰਵੈਦਿਕ ਦਵਾਈਆਂ ਦੀ ਬਜ਼ਾਰ ਵਿੱਚ ਕਾਫ਼ੀ ਮੰਗ ਹੈ । ਅਜਿਹੇ ਪੰਜ ਉਤਪਾਦਾਂ ਦਾ ਬਜ਼ਾਰ ਵਿੱਚ ਪਤਾ ਕਰੋ ।
ਉੱਤਰ-
| ਪੌਦੇ ਦਾ ਨਾਂ | ਵਰਤਿਆ ਜਾਂਦਾ ਹਿੱਸਾ | ਬਿਮਾਰੀ ਦਾ ਨਾਂ |
| 1. ਤੁਲਸੀ ਪੱਤੇ | ਪੱਤੇ | ਬੁਖਾਰ, ਗਲੇ ਦੀ ਖਰਾਬੀ |
| 2. ਬ੍ਰਹਮੀ ਬੂਟੀ | ਪੱਤੇ ਅਤੇ ਤਣਾ | ਦਿਮਾਗੀ ਅਤੇ ਸਰੀਰਕ ਕਮਜ਼ੋਰੀ |
| 3. ਅਜਵਾਇਣ | ਬੀਜ | ਪੇਟ ਦਰਦ ਅਤੇ ਜੀ ਮਤਲਾਉਣਾ |
| 4. ਨਿੰਮ | ਪੱਤੇ, ਫਲ ਅਤੇ ਬੀਜ | ਪੱਤਿਆਂ ਦਾ ਕਾਹੜਾ ਪੀਣ ਨਾਲ ਜਾਂ ਪੱਤੇ ਚੱਬਣ ਨਾਲ ਫੋੜੇ ਫਿਨਸੀਆਂ ਠੀਕ ਹੋ ਜਾਂਦੀਆਂ ਹਨ । ਫਲ ਖਾਣ ਨਾਲ ਖੂਨ ਸਾਫ ਹੋ ਜਾਂਦਾ ਹੈ । ਬੀਜਾਂ ਤੋਂ ਛਾਲ ਮੋਗਰਾ ਤੇਲ ਪ੍ਰਾਪਤ ਹੁੰਦਾ ਹੈ, ਜਿਸ ਦੀ ਵਰਤੋਂ ਕੁਸ਼ਟ ਰੋਗ (Leprosy) ਲਈ ਕੀਤੀ ਜਾਂਦੀ ਹੈ । |
| 5. ਸੌਂਫ | ਬੀਜ (ਫਲ) | ਪੇਟ ਦੀਆਂ ਬਿਮਾਰੀਆਂ ਲਈ |
| 6. ਪੁਦੀਨਾ | ਪੱਤੇ | ਪੇਟ ਦਰਦ ਦੇ ਲਈ ਅਤੇ ਪੇਸ਼ਾਬ ਦੀਆਂ ਬਿਮਾਰੀਆਂ ਲਈ |
| 7. ਫੋਕਸ ਗਲੋਵ | ਬੀਜ ਅਤੇ ਪੱਤੇ | ਦਿਲ ਦੀਆਂ ਬਿਮਾਰੀਆਂ ਦੇ ਲਈ ਮੁਫੀਦ ਹੈ । |
| 8. ਕੁਆਰ ਗੰਦਲ | ਗੁੱਦੇਦਾਰ ਪੱਤੇ | ਪੇਟ ਦੇ ਲਈ ਅਤੇ ਚਿਹਰੇ ਦੇ ਨਿਖਾਰ ਦੇ ਲਈ । |