Punjab State Board PSEB 11th Class Maths Book Solutions Chapter 16 Probability Ex 16.2 Textbook Exercise Questions and Answers.
PSEB Solutions for Class 11 Maths Chapter 16 Probability Ex 16.2
Question 1.
A die is rolled. Let E be the event “die shows 4” and F be the event “die shows even number”. Are E and F mutually exclusive?
Answer.
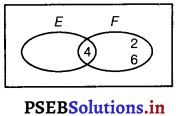
If we roll a die, then all the possible outcomes will be
S= {1, 2, 3, 4, 5, 6}
E = Die shows 4 = {4}
F = Die shows an even number = (2, 4, 6}
⇒ E ∩ F = {4} ∩ {2, 4, 6} = {4}
⇒ E ∩ F ≠ Φ
Hence, E and F are not mutually exclusive.
![]()
Question 2.
A die is thrown. Describe the following events:
(i) A: a number less than 7
(ii) B: a number greater than 7
(iii) C: a multiple of 3
(iv) D: a number less than 4
(v) E: an even number greater than 4
(vi) F: a number not less than 3.
Also find A ∪ B, A ∩ B, B ∪ C, E ∩ F, D ∩ E, A – C, D – E, E ∩ F, F.
Answer.
When a die is throw, then sample space S = {1, 2, 3, 4, 5, 6}
(i) A : a number less than 7 = {1, 2, 3, 4, 5, 6}
(ii) B: a number greater than 7 = {} = {Φ}
(iii) C : a multiple of 3 = {3, 6}
(iv) D: a number less than 4 = {1, 2, 3}
(v) E : an even number greater than 4 = {6}
(vi) F : a number not less than 3 = {3, 4, 5, 6}
Now, A ∪ B = The elements which are in both A and B
{1, 2, 3, 4, 5, 6} ∪ – Φ = {1, 2, 3, 4, 5, 6}
A ∩ B – The elements which are common in both A and B
= {1, 2, 3, 4, 5, 6} ∩ Φ = Φ
B ∪ C = The elements which are in both B and C
= { } ∪ {3, 6} = {3, 6}
E ∩ F = The elements which are common in both E and F
= {6} ∩ {3, 4, 5, 6} = {6}
D ∩ E = The elements which are common in both D and E
= {1, 2, 3,} ∩ {6} = Φ
A – C = The elements which are in A but not in C
= {1, 2, 3, 4, 5, 6} – {3, 6} = {1, 2, 4, 5}
D – C = The elements which in D but not in E
= {1, 2, 3} – {6} = {1, 2, 3}
F’ = (S – F) = {1, 2, 3, 4, 5, 6} – {3, 4, 5, 6} = {1, 2}
and E ∩ F’ = E ∩ (S – F)
= {6} ∩ {1, 2} = Φ.
![]()
Question 3.
An experiment involves rolling a pair of dice and recording the numbers that come up. Describe the following events:
A : the sum is greater than 8,
B : 2 occurs on either die
C : The sum is at least 7 and a multiple of 3.
Which pairs of these events are mutually exclusive?
Answer.
When a pair of dice is rolled, the sample space is given by S = {(x, y) : x, y = 1, 2, 3, 4, 5, 6}

Accordingly,
A = {(3, 6), (4, 5), (4, 6), (5, 4), (5, 5), (5, 6), (6, 3), (6, 4), (6, 5), (6, 6)}
B = {(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (1, 2), (3, 2), (4, 2), (5, 2), (6, 2)}
C = {(3, 6), (4, 5), (5, 4), (6, 3), (6, 6)}
It is observed that
A ∩ B = Φ
B ∩ C = Φ
C ∩ A = {(3, 6), (4, 5), (5, 4), (6, 3), (6, 6)}
Hence, events A and B and events B and C are mutually exclusive.
![]()
Question 4.
Three coins are tossed once. Let A denote the event “three heads show”, B denote the event “two heads and one tail show”, C denote the event “three tails show” and D denote the event “a head shows on the first coin”.
Which events are
(i) mutually exclusive?
(ii) simple?
(iii) compound?
Answer.
When three coins are tossed, the sample space is given by S = {HHH, HHT, HTH, HTT, THH, THT, TTH, TIT}
Accordingly,
A = {HHH},
B = {HHT, HTH, HTT},
C = (TIT),
D = {HHH, HHT, HTH, HTT}
We now observe that
A ∩ B = Φ A ∩ C = Φ; B ∩ C = Φ; C ∩ D = Φ
A ∩ B ∩ C = Φ
(i) Event A and B; event A and C; event B and C; event C and D and event A, B,C are all mutually exclusive.
(ii) If an event has only one sample point of a sample space, it is called a simple event, Thus, A and C are simple events.
(iii) If an event has more than one sample point of a sample space, it is called a compound event. Thus, B and D are compound events.
![]()
Question 5.
Three coins are tossed. Describe
(i) Two events which are mutually exclusive.
(ii) Three events which are mutually exclusive and exhaustive.
(iii) Two events, which are not mutually exclusive. 1
(iv) Two events which are mutually exclusive but not exhaustive,
(v) Three events which are mutually exclusive but not exhaustive.
Answer.
When three coins are tossed, then the sample space S is S={HHH, HHT, HTH, HTT, THH, THT, TTH, TIT}
(i) Two events A and B which are mutually exclusive are
A : “getting at least two heads” and
B : “getting at least two tails”.
(ii) Three events A, B and C which are mutually exclusive and exhaustive are
A : “getting at most one head”
B : “getting exactly two heads”
C : “getting exactly three heads”.
Alternatively A : “getting no head”
B : “getting exactly on head”
C : “getting at least two heads”.
(iii) Two events A and B which are not mutually exclusive are
A : “getting at most two tails” and
B : “getting exactly two heads” or “getting exacdy two tails”.
(iv) Two events A and B which are mutually exclusive but not exhaustive are
A : “getting exactly one head” and
B : “getting exactly two heads”.
(v) Three events A, B and C which are mutually exclusive but not exhaustive are
A : “getting exacdy one tail”
B : “getting exacdy two tails” and
C : “getting exacdy three tails”.
![]()
Question 6.
Two dice are thrown. The events A, B and C are as follows:
A: getting an even number on the first die.
B: getting an odd number on the first die.
C: getting the sum of the numbers on the dice ≤ 5
Describe the events
Answer.
When two dice are thrown, the sample space is given by
S = {(x, y): x, y 1, 2, 3, 4, 5, 6}
= [(1, 1), (1, 2), (1, 3),(1, 4), (1, 5) ,(1, 6)
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6)
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6)
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6)
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6)
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)]
Accordingly,
A = {(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (4, 1), (4, 2), (4, 3) (4, 4), (4, 5), (4, 6) (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}.
B = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6)}.
C = {(1 ,1), (1, 2), (1, 3), (1, 4), (2, 1), (2, 2), (2, 3), (3, 1), (3, 2), (4, 1)}
(i) A’ = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6)} = B
(ii) Not B = B’ = {(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
= A.
![]()
(iii) A or B = A ∪ B
= {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6)
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6)
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6)
(4, 1), (4, 2),(4, 3), (4, 4), (4, 5), (4, 6)
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6)
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)} = S
(iv) A and B = A ∩ B = Φ
(v) A but not C = A – C
= {(2, 4), (2, 5), (2, 6), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6,6)}
(vi) B or C = B ∪ C
= {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 1), (2, 2), (2, 3), (3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (4, 1), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6)}
(vii) B and C = B ∩ C = {(1, 1), (1, 2), (1, 3), (1, 4), (3, 1), (3, 2)}
= {(1, 5), (1, 6), (2, 4), (2, 5), (2, 6), (3, 3), (3, 4), (3, 5), (3, 6), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
∴ A ∩ B’ ∩ C’ = A ∩ A ∩ C’ = A ∩ C’
= {(2, 4), (2, 5), (2, 6), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}.
![]()
Question 7.
Refer to question 6 above state true or false (given reason for your answer).
(i) A and B are mutually exclusive.
(ii) A and B are mutually exclusive and exhaustive.
(iii) A = B’
(iv) A and C are mutually exclusive.
(v) A and B’ are mutually exclusive.
(vi) A’, B’ and C are mutually exclusive and exhaustive.
Answer.
(i) True
∵ A = getting an even number on the first die
B = getting an odd number on the first die
⇒ A ∩ B = Φ
∴ A and B are mutually exclusive events.
(ii) True
A ∪ B = S, i.e., exhaustive. Also, A ∩ B = Φ
(iii) True
∴ B = getting an odd number on the first die
⇒ B’ = getting an even number on the first die = A
∴ A = B’
(iv) False
∵ A ∩ C = {(2, 1), (2, 2), (2, 3), (4, 1)} ≠ Φ.
So A and C are not mutually exclusive.
(v) False
∵ B’ = A
A ∩ B’ = A ∩ A = A ≠ Φ (∵ B’ = A)
So, A and B’ are not mutually exclusive.
(vi) False
A’ ∩ B’ = Φ
A’ ∩ B’ ∩ C = Φ and A’ ∪ B’ ∪ C = S
But A’ ∩ C = B ∩ C = {(1, 1), (1, 2), (1, 3), (1, 4), (3, 1), (3, 2)} ≠ Φ
(∵ A’ = B)
and B’ ∩ C = A ∩ C = {(2, 1), (2, 2), (2, 3), (4, 1)} ≠ Φ (∵ B’ = A)
∴ A’, B’ and C are not mutually exclusive.
