PSEB 11th Class Maths Solutions Chapter 3 Trigonometric Functions Ex 3.2

Punjab State Board PSEB 11th Class Maths Book Solutions Chapter 3 Trigonometric Functions Ex 3.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.2

Question 1.
Find the values of other five trigonometric functions if cos x = – \(\frac{1}{2}\) x lies in third quadrant.
Answer.

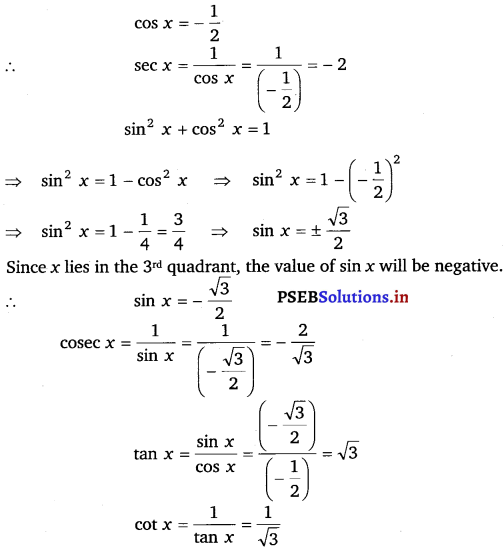
Question 2.
Find the values of other five trigonometric functions if sin x = \(\frac{3}{5}\), x lies in second quadrant.
Answer.
sin x = \(\frac{3}{5}\)

cosec x = \(\frac{1}{\sin x}=\frac{1}{\left(\frac{3}{5}\right)}=\frac{5}{3}\)
sin² x + cos² x = 1
⇒ cos² x = 1 – sin² x
⇒ cos² x = 1 – (\(\frac{3}{5}\))²

⇒ cos² x = 1 – \(\frac{9}{25}\)

⇒ cos² x = \(\frac{16}{25}\)

⇒ cos x = ± \(\frac{4}{5}\)
Since x lies in the 2nd quadrant, the value of cos x will be negative

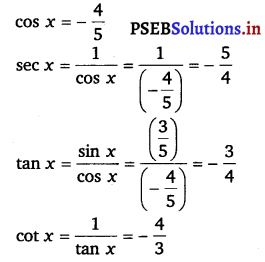
Question 3.
Find the values of other five trigonometric functions if cot x = \(\frac{3}{4}\), x lies in third quadrant.
Answer.

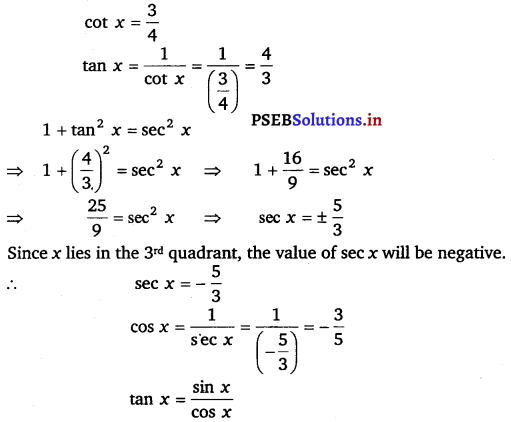
⇒ \(\frac{4}{3}=\frac{\sin x}{\frac{-3}{5}}\)

⇒ sin x = \(\left(\frac{4}{3}\right) \times\left(\frac{-3}{5}\right)=-\frac{4}{5}\)

⇒ cosec x = \(\frac{1}{\sin x}=-\frac{5}{4}\).

Question 4.
Find the values of other five trigonometric functions if sec x = \(\frac{13}{5}\), x lies in fourth quadrant.
Answer.

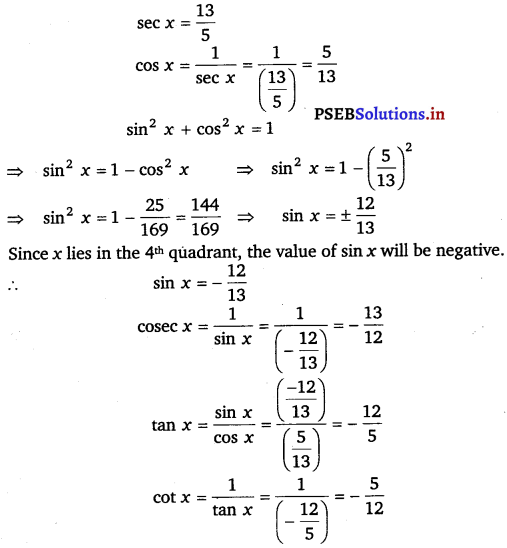
Question 5.
Find the values of other five trigonometric functions if tan x = \(\frac{5}{12}\), x lies in second quadrant.
Answer.
tan x = – \(\frac{5}{12}\)

cot x = \(\frac{1}{\tan x}=\frac{1}{\left(-\frac{5}{12}\right)}=-\frac{12}{5}\)

1 + tan² x = sec² x

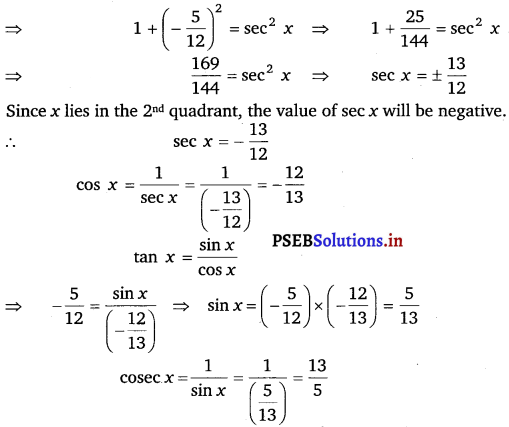
Question 6.
Find the value of the trigonometric function sin 765°.
Ans.
It is known that the values of sin x repeat after an interval of 2π or 360°.
∴ sin 765° = sin (2 × 360° + 45°)
= sin 45° = 1

Question 7.
Find the value of the trigonometric function cosec (- 1410°).
Answer.
It is known that the values of cosec x repeat after an interval of 2π or 360°.
∴ cosec (- 1410°) = cosec (- 1410° + 4 x 360°)
= cosec (- 1410° + 1440°)
= cosec 30° = 2.

Question 8.
Find the value of the trigonometric function tan \(\frac{19 \pi}{3}\).
Answer.
It is known that the values of tan x repeat after an interval of π or 180°.
∴ \(\tan \frac{19 \pi}{3}=\tan 6 \frac{1}{3} \pi\)

= \(\tan \left(6 \pi+\frac{\pi}{3}\right)=\tan \frac{\pi}{3}\)

= tan 60° = √3.

Question 9.
Find the value of the trigonometric function sin \(\left(-\frac{11 \pi}{3}\right)\).
Answer.
It is known that the values of cot x repeat after an interval of π or 180°.

∴ \(\sin \left(\frac{11 \pi}{3}\right)=\sin \left(-\frac{11 \pi}{3}+2 \times 2 \pi\right)\)

= \(\sin \left(\frac{\pi}{3}\right)=\sin 60^{\circ}=\frac{\sqrt{3}}{2}\)

Question 10.
Find the value of the trigonometric function cot \(\left(-\frac{15 \pi}{4}\right)\).
Answer.
It is known that the values of cot x repeat after an interval of ir or 1800.
∴ \(\cot \left(-\frac{15 \pi}{4}\right)=\cot \left(-\frac{15 \pi}{4}+4 \pi\right)=\cot \frac{\pi}{4}\) = 1.