PSEB 11th Class Maths Solutions Chapter 3 Trigonometric Functions Ex 3.4

Punjab State Board PSEB 11th Class Maths Book Solutions Chapter 3 Trigonometric Functions Ex 3.4 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.4

Question 1.
Find the principal and general solutions of the equation, tan x = √3
Answer.
tan x = √3
It is known that:
tan $\frac{\pi}{3}$ = √3 and
tan ($\frac{4 \pi}{3}$) = tan ( π + $\frac{\pi}{3}$)
= tan $\frac{\pi}{3}$ = √3
Therefore, the principal solutions are x = $\frac{\pi}{3}$ and $\frac{4 \pi}{3}$.
Now, tan x = tan $\frac{\pi}{3}$
⇒ x = nπ + $\frac{\pi}{3}$, where n ∈ Z
Therefore, the general solution is x = nπ + $\frac{\pi}{3}$, where n ∈ Z.

Question 2.
Find the principal and general solutions of the equation: sec x = 2
Answer.
sec x = 2
It is known that:
sec $\frac{\pi}{3}$ = 2 and
sec $\frac{5 \pi}{3}$ = sec (2π – $\frac{\pi}{3}$)
= sec $\frac{\pi}{3}$ = 2
Therefore, the principal solutions are x = $\frac{\pi}{3}$ and $\frac{5 \pi}{3}$.
Now, sec x = sec $\frac{\pi}{3}$
cos x = cos $\frac{\pi}{3}$ [∵ sec x = $\frac{1}{\cos x}$]
⇒ x = 2nπ ± $\frac{\pi}{3}$, where n e Z
Therefore, the general solution is x = 2nπ ± $\frac{\pi}{3}$, where n ∈ Z.

Question 3.
Find the principal and general solutions of the equation cot cot x = – √3.
Answer.
cot x = – √3

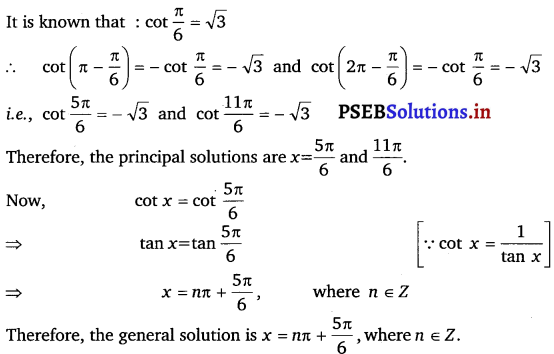
Question 4.
Find the principal and general solutions of cosec x = – 2
Answer.
cosec x = – 2
It is known that:
cosec $\frac{\pi}{6}$ = 2

Question 5.
Find the general solution of the equation: cos 4x = cos 2x
Answer.
cos 4x = cos 2x
cos 4x – cos 2x = 0
– 2 sin $\left(\frac{4 x+2 x}{2}\right)$ sin $\left(\frac{4 x-2 x}{2}\right)$ = 0

[∵ cos A – cos B = 2 \sin \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)]

sin 3x sin x = 0
sin 3x = 0or sin x = 0
3x = nπ or x = nπ, where n ∈ Z
x = $\frac{n \pi}{3}$ or x = nπ, where n ∈ Z.

Question 6.
Find the general solution of the equation cos 3x + cosx – cos 2x = 0
Answer.
cos 3x + cos x – cos 2x = 0
2 cos $\left(\frac{3 x+x}{2}\right)$ cos $\left(\frac{3 x-x}{2}\right)$ – cos 2x = 0

[∵ cos A + cos B = 2 $\cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$]

2 cos 2x cos x – cos 2x = 0
cos 2x (2 cos x – 1) = 0
cos 2x = 0 or 2 cos x – 1 = 0
cos 2x = 0 or cos x = $\frac{1{2}$
∴ 2x = (2n + 1) $\frac{\pi}{2}$ or cos x = cos $\frac{\pi}{3}$, where n ∈ Z
x = (2n + 1) $\frac{\pi}{4}$ or x = 2nπ ± $\frac{\pi}{3}$ where n ∈ Z.

Question 7.
Find the general solution of the equation sin 2x + cos x = 0
Answer.
sin 2x + cos x = 0
⇒ 2sin x cos x + cos x = 0
⇒ cos x (2 sin x + 1) = 0
⇒ cos x = 0 or 2 sin x + 1 = 0
Now, cos x = 0
⇒ x = (2n + 1) $\frac{\pi}{2}$ , where n ∈ Z.
or 2 sin x + 1 = 0
⇒ sin x = – $\frac{1}{2}$

= – sin $\frac{\pi}{6}$

= sin (π + $\frac{\pi}{6}$)

= sin $\frac{7 \pi}{6}$

x = nπ + (- 1)ⁿ $\frac{7 \pi}{6}$ where n ∈ Z
Therefore, the general solution is (2n + 1) $\frac{\pi}{2}$ or nπ + (- 1)ⁿ $\frac{7 \pi}{6}$ where n ∈ Z.

Question 8.
Find the general solution of the equation sec² 2x = 1 – tan 2x.
Answer.
sec² 2x = 1 – tan 2x
1 + tan² 2x = 1 – tan 2x
tan² x + tan 2x = 0
=> tan 2x (tan 2x + 1) = 0
=> tan 2x = 0 or tan 2x + 1 = 0
Now, tan 2x = 0
=> tan 2x = tan 0
2x = nπ + 0, where n ∈ Z
x = $\frac{n \pi}{2}$, where n ∈ Z
or tan 2x + 1 = 0
= tan 2x = – 1
= – tan $\frac{\pi}{4}$

= tan (π – $\frac{\pi}{4}$)

= tan $\frac{3 \pi}{4}$

2x = nπ + $\frac{3 \pi}{4}$ where n ∈ Z

x = $\frac{n \pi}{2}+\frac{3 \pi}{8}$, where n ∈ Z

Therefore, the general solution is $\frac{n \pi}{2}$ or $\frac{n \pi}{2}+\frac{3 \pi}{8}$ where n ∈ Z.

Question 9.
Find the general solution of the equation sin x + sin 3x + sin 5x = 0
Answer.
sin x + sin 3x + sin 5x = 0
⇒ (sin x + sin 5x) + sin 3x = 0

$\left[2 \sin \left(\frac{x+5 x}{2}\right) \cos \left(\frac{x-5 x}{2}\right)\right]$ + sin 3x = 0

[∵ sin A + sin B = 2 sin $\sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$]

2 sin 3x cos (2x) + sin 3x = 0
2 sin 3x cos 2x + sin 3x = 0
sin 3x (2 cos 2x +1) = 0
sin 3x = 0 or 2 cos 2x + 1 = 0
Now sin 3x = 0
⇒ 3x = nπ, where n ∈ Z
i.e., x = $\frac{n \pi}{3}$ where n ∈ Z
or 2 cos 2x + 1 = 0
cos 2x = $-\frac{1}{2}$

= – cos $\frac{\pi}{3}$

= cos (π – $\frac{\pi}{3}$)

cos 2x = cos $\frac{2 \pi}{3}$

⇒ 2x = 2nπ ± $\frac{2\pi}{3}$, where n ∈ Z

⇒ x = nπ ± $\frac{\pi}{3}$, where n ∈ Z

Therefore, the general solution is $\frac{n \pi}{3}$ or nπ ± $\frac{\pi}{3}$, where n ∈ Z.