PSEB 12th Class Maths Solutions Chapter 7 Integrals Ex 7.8

Punjab State Board PSEB 12th Class Maths Book Solutions Chapter 7 Integrals Ex 7.8 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 12 Maths Chapter 7 Integrals Ex 7.8

Direction (1 – 6): Evaluate the following definite integrals as limit of sums.

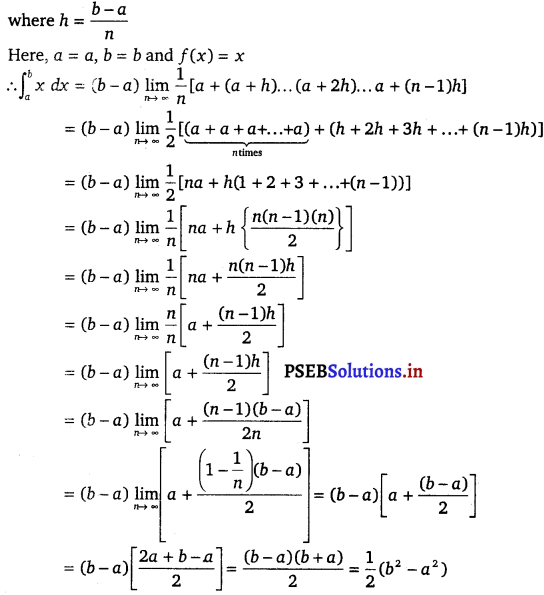
Question 1.
\(\int_{a}^{b}\) x dx
Solution.
We know that,
\(\int_{a}^{b}\) f(x) dx = (b – a) \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [f(a) + f(a + h) + ……… + f(a + (n – 1) h]

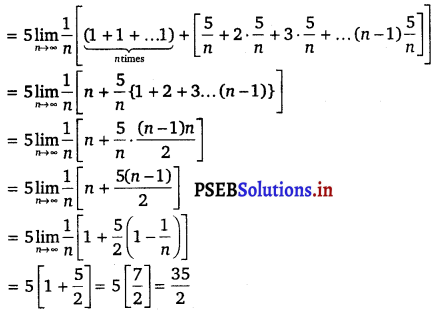
Question 2.
\(\int_{0}^{5}\) (x + 1)
Solution.
Let I = \([\int_{0}^{5}/latex] (x + 1) dx
We know that,
[latex]\int_{a}^{b}\) f(x) dx = (b – a) \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [f(a) + f(a + h) + ……… + f(a + (n – 1) h]

where h = \(\frac{b-a}{n}\)
Here, a = 0, b = 5 and f(x) = (x + 1)

⇒ h = \(\frac{5-0}{n}=\frac{5}{n}\)

∴ \([\int_{0}^{5}/latex] (x + 1) dx = [latex](5-0) \lim _{n \rightarrow \infty} \frac{1}{n}\left[f(0)+f\left(\frac{5}{n}\right)+\ldots+f\left((n-1) \frac{5}{n}\right)\right]\)

= \(5 \lim _{n \rightarrow \infty} \frac{1}{n}\left[1+\left(\frac{5}{n}+1\right)+\ldots+\left\{1+\left(\frac{5(n-1)}{n}\right)\right\}\right]\)

Question 3.
\(\int_{2}^{3}\) x² dx
Solution.
We know that,
\(\int_{a}^{b}\) f(x) dx = (b – a) \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [f(a) + f(a + h) + ……… + f(a + (n – 1) h]

where h = \(\frac{b-a}{n}\)
Here, a = 2, b – 3 and f(x) = x²
⇒ h = \(\frac{3-2}{n}=\frac{1}{n}\)
∴ \(\int_{2}^{3}\) x² dx

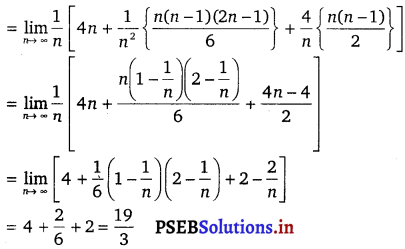
Question 4.
\(\int_{1}^{4}\) (x² – x) dx
Solution.
We know that,
\(\int_{a}^{b}\) f(x) dx = (b – a) \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [f(a) + f(a + h) + ……… + f(a + (n – 1) h]
where nh = b – a
Given, \(\int_{1}^{4}\) (x² – x) dx,
here a = 1, b = 4 and nh = 3 and
f(x) = x² – x = x (x – 1)
∴ \(\int_{1}^{4}\) (x² – x) dx = \(\lim _{h \rightarrow 0}\) h [f(1) + f(1 + h) + f (1 + 2h) +……… + f (1 + (n – 1) h)]

= \(\lim _{h \rightarrow 0}\) h [1(1 – 1) + (1 + h) h + (1 + 2h) (2h) + ……………. + {1 + (n – 1) h} {(n – 1) h}] [∵ f(x) = x (x – 1)]
f(1) = 1 (1 – 1);
f(1 + h) = (1 + h) (1 + h – 1) = (1 + h) h and so on]
= \(\lim _{h \rightarrow 0}\) h² [(1 + h) + 2 (1 + 2h) + 3 (1 + 3h) + ……… + (n – 1)(1 + (n – 1) h)]

= \(\lim _{h \rightarrow 0}\) h² [{1 + 2 + 3 + ………….. + (n – 1)} + {h + 2² h + 3² h + …………. +(n – 1)²h)}]

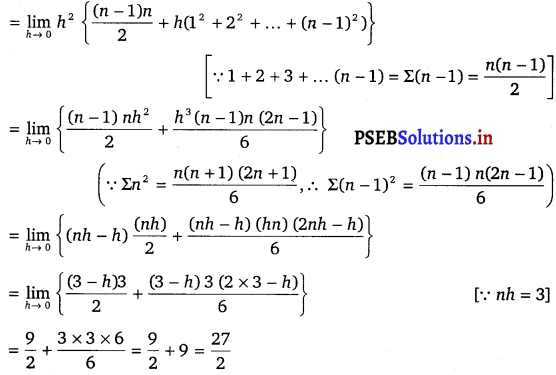
Question 5.
\(\int_{-1}^{1}\) e^x dx
Solution.
Let I = \(\int_{-1}^{1}\) e^x dx ………… (i)
We know that,
\(\int_{a}^{b}\) f(x) dx = (b – a) \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [f(a) + f(a + h) + ……… + f(a + (n – 1) h]
where h = \(\frac{b-a}{n}\)
Here, a = – 1, = 1 and f(x) = e^x

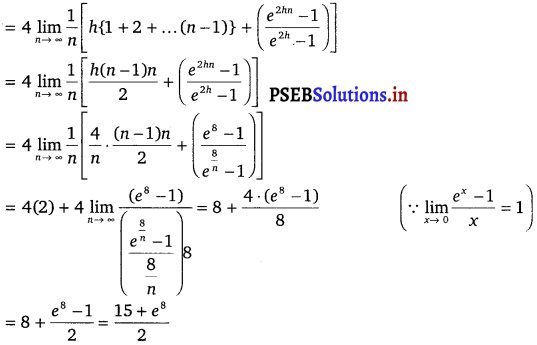
Question 6.
\(\int_{0}^{4}\) (x + e^2x)dx
Solution.
We know that,
\(\int_{a}^{b}\) f(x) dx = (b – a) \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [f(a) + f(a + h) + ……… + f(a + (n – 1) h]
where h = \(\frac{b-a}{n}\)
Here, a = 0, b = 4 and f(x) = x + e^2x
∴ h = \(\frac{4-0}{n}=\frac{4}{n}\)
⇒ \(\int_{0}^{4}\) (x + e^2x)dx = (4 – 0) \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [f(0) + f(h) + f(2h) + ……………. + f(n – 1) h)]

= 4 \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [(0 + e⁰) + (h + e^2h) + (2h + e^{2 . 2h}) + ……… {(n – 1) h + e^{2 (n – 1) h}}]

= 4 \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [1 + (h + e^2h) + (2h + e^4h) + …………. + (n – 1) h + e^{2 (n – 1) h}}] [∵ e⁰ = 1]

= 4 \(\lim _{n \rightarrow \infty} \frac{1}{n}\) [{h + 2h + 3h + ……….. + (n – 1) h} + (1 + e^2h + e^4h + ………….. + e^{2 (n – 1) h})}]