Punjab State Board PSEB 9th Class Maths Book Solutions Chapter 11 Constructions Ex 11.1 Textbook Exercise Questions and Answers.
PSEB Solutions for Class 9 Maths Chapter 11 Constructions Ex 11.1
Question 1.
Construct an angle of 90° at the initial point of a given ray and justify the construction.
Answer:
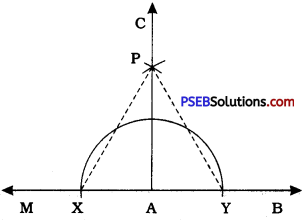
Steps of construction :
- Ray AB is given. Produce ray AB on the side of A to get line MAB.
- Taking A as centre and some radius, draw an arc of a circle to intersect line MAB at X and Y.
- Taking X and Y as centres and radius more than \(\frac{1}{2}\)XY, draw arcs to intersect at P on one side of line MAB.
- Draw ray AC passing through E
Thus, ∠CAB is the required angle of 90°.
Justification:
Draw PX and PY.
In ∆ PAX and ∆ PAY,
AX = AY (Radii of same arc)
PX = PY (Radii of congruent arcs)
PA = PA (Common)
∴ By SSS rule, ∆ PAX ≅ ∆ PAY
∴ ∠PAX = ∠PAY (CPCT)
But, ∠PAX + ∠PAY = 180° (Linear pair)
∴ ∠PAY = \(\frac{180^{\circ}}{2}\) = 90°
∴ ∠CAB = 90°
![]()
Question 2.
Construct an angle of 45° at the initial point of a given ray and justify the construction.
Answer:
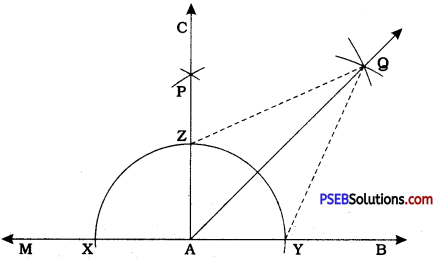
Steps of construction:
- Ray AB is given. Produce ray AB on the side of A to get line MAB.
- Taking A as centre and some radius, draw an arc of a circle to intersect line MAB at X and Y.
- Taking X and Y as centres and radius more than \(\frac{1}{2}\)XY, draw arcs to intersect at P on one side of line MAB.
- Draw ray AC passing through E Thus, ∠CAB of 90° is received.
- Name the point of intersection of the arc with centre A and ray AC as Z.
- Taking Y and Z as centres and radius more than \(\frac{1}{2}\)YZ, draw arcs to intersect each other at Q.
- Draw ray AQ.
Thus, ∠QAB is the required angle of 45°.
Justification:
In example 1, we have already justified that ∠CAB = 90°. So, we do not repeat that part’ here.
Draw QZ and QY.
In ∆ AYQ and ∆ AZQ,
AY = AZ (Radii of same arc)
YQ = ZQ (Radii of congruent arcs)
AQ = AQ (Common)
∴ By SSS rule, ∆ AYQ ≅ ∆ AZQ
∴ ∠QAY = ∠QAZ (CPCT)
But, ∠QAY + ∠QAZ = ∠ZAY = ∠CAB = 90°
∴ ∠QAY = \(\frac{90^{\circ}}{2}\) = 45°
∴ ∠QAB = 45°
![]()
Question 3.
Construct the angles of the following measurements:
(i) 30°
Answer:
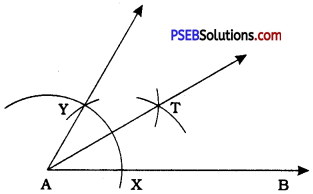
Steps of construction:
- Draw any ray AB. With centre A and any radius, draw an arc to intersect AB at X.
- With centre X and the same radius [as in step (1)], draw an arc to intersect the previous arc at Y. Draw ray AY. Then, ∠YAB = 60°.
- Draw ray AT, the bisector of ∠YAB.
Thus, ∠TAB is the required angle of 30°.
(ii) 22\(\frac{1}{2}\)°
Answer:
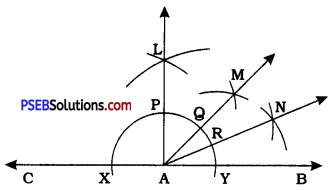
Steps of construction:
- Draw any ray AB. Produce AB on the side of A to get line CAB.
- Taking A as centre and any radius, draw an arc of a circle to intersect line CAB at X and Y.
- Taking X and Y as centres and radius more than \(\frac{1}{2}\)XY, draw arcs to intersect each other at L on one side of line CAB. Draw ray AL. Then, ∠LAB = 90°.
- Draw ray AM, the bisector of ∠LAB. Then, ∠MAB = 45°.
- Draw ray AN, the bisector of ∠MAB. Then, ∠NAB = 22\(\frac{1}{2}\)°.
Thus, ∠NAB is the required angle of 22\(\frac{1}{2}\)°.
![]()
(iii) 15°
Answer:
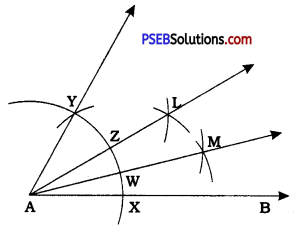
Steps of construction:
- Draw any ray AB. Taking A as centre and any radius, draw an arc of a circle to intersect AB at X.
- Taking X as centre and the same radius as before, draw an arc to intersect the previous arc at Y. Draw ray AY. Then, ∠YAB = 60°.
- Draw ray AL, the bisector of ∠YAB. Then, ∠LAB = 30°.
- Draw ray AM, the bisector of ∠LAB. Then, ∠MAB = 15°.
Thus, ∠MAB is the required angle of 15°.
Question 4.
Construct the following angles and verify by measuring them by a protractor:
(i) 75° and (ii) 105°
Answer:
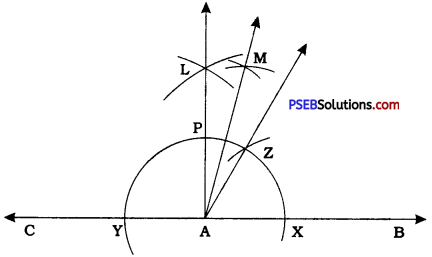
Steps of construction:
- Draw any ray AB and produce it on the side of A to get line CAB. Taking A as centre and any radius draw an arc of a circle to intersect line CAB at X and Y.
- Taking X and Y as centres and radius more than \(\frac{1}{2}\)XY, draw arcs to intersect each other at point L on one side of line CAB. Draw ray AL. Then, ∠LAB = 90°.
- Taking X as centre and radius AX, draw an arc of a circle to Intersect the first arc (arc XY) with centre A at Z.
- Draw ray AZ. Then, ∠ZAB = 60°.
- Now, draw ray AM, the bisector of ∠LAZ. Then, ∠MAB = 75° and ∠MAC = 105°.
Thus, ∠MAB and ∠MAC are the required angles of measure 75° and 105° respectively.
![]()
(iii) 135°
Answer:
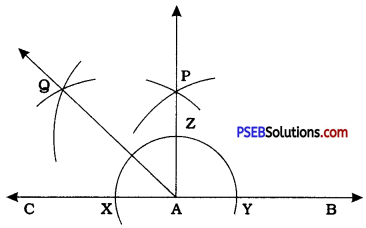
Steps of construction:
- Draw line CAB. Taking A as centre and any radius, draw an arc of a circle to Intersect line CAB at X and Y.
- Taking X and Y as centres and radius more them \(\frac{1}{2}\)XY, draw arcs to intersect each other at P on one side of line CAB.
- Draw ray AP Then, ∠PAB = ∠PAC = 90°.
- Draw ray AQ, the bisector of ∠PAC.
- Then, ∠QAB = 135°.
Thus, ∠QAB is the required angle of 135°.
![]()
Question 5.
Construct an equilateral triangle, given its side and justify the construction.
Answer:
Line segment XY is given. We have to construct an equilateral triangle with each side being equal to XY.

Steps of construction:
- Draw any ray BM.
- With centre B and radius XY, draw an arc of a circle to intersect BM at C.
- Taking B and C as centres and rhdius XY, draw arcs to intersect each other at A on one side of line AC.
- Draw AB and AC.
Thus, ∆ ABC is the required equilateral triangle with each side being equal to XY.
Justification:
The arc drawn with centre B and radius XY intersects ray BM at C. ∴ BC = XY. The arcs drawn with centres B and C and radius XY intersect at A.
∴ AB = XY and AC = XY.
Thus, in ∆ ABC, AB = BC = AC = XY.
Hence, ∆ ABC is an equilateral triangle in which all sides are equal to XY.
Note: If the measure of sides are given numerically, e.g., 4 cm, 5 cm, etc., then we have to use graduated scale instead of straight edge.
