PSEB 10th Class Maths Solutions Chapter 6 Triangles Ex 6.4

Punjab State Board PSEB 10th Class Maths Book Solutions Chapter 6 Triangles Ex 6.4 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.4

Question 1.
Let △ABC ~ △DEF and their areas be respectively 64 cm² and 121 cm². If EF = 15.4 cm, find BC.
Solution:
△ABC ~ △DEF ;
area of △ABC = 64 cm²;
area of △DEF = 121 cm²;
EF= 15.4 cm

△ABC ~ △DEF

∴ \(\frac{\text { ar. }(\Delta \mathrm{ABC})}{\text { ar. }(\Delta \mathrm{DEF})}=\frac{\mathrm{AB}^{2}}{\mathrm{DE}^{2}}=\frac{\mathrm{AC}^{2}}{\mathrm{DF}^{2}}=\frac{\mathrm{BC}^{2}}{\mathrm{EF}^{2}}\)
(If two traingles are similar, ratio of their area is square of corresponding sides }

\(\frac{64}{121}=\frac{\mathrm{BC}^{2}}{\mathrm{EF}^{2}}\) \(\left(\frac{8}{11}\right)^{2}=\left(\frac{\mathrm{BC}}{15.4}\right)^{2}\)

⇒ \(\frac{8}{11}=\frac{\mathrm{BC}}{15.4}\)

BC = \(\frac{8 \times 15.4}{11}\)
BC = 8 × 1.4
BC = 11.2 cm.

Question 2.
Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2 CD. Find the ratio of the areas of traingles AOB and COD.
Solution:
ABCD is trapezium AB || DC. Diagonals AC and BD intersects each other at the point O. AB = 2 CD

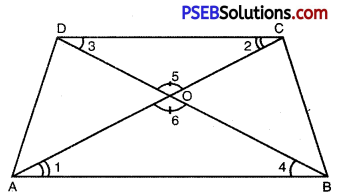
In △AOB and △COD,
∠1 = ∠2 (alternate angles)
∠3 = ∠4 (alternate angles)
∠5 = ∠6 (vertically opposite angle)
∴ △AOB ~ △COD [AA, A similarity criterion]

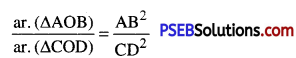
(If two triangles are similar ratio of their areas is square of corresponding sides)

= \(\frac{(2 \mathrm{CD})^{2}}{\mathrm{CD}^{2}}\) [∵ AB = 2 CD] (Given)

∴ Required ratio of ar △AOB and △COD = 4 : 1.

Question 3.
In the fig., △ABC and △DBC are two triangles on the same base BC. If AD intersects BC at O show that

Solution:
Given. ∆ABC and ∆DBC are the triangles on same base BC. AD intersects BC at O

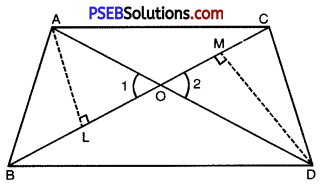
To Prove:
Construction: Draw AL ⊥ BC, DM ⊥ BC
Proof: In ∆ALO and ∆DMO.
∠1 = ∠2 (vertically opposite angle)
∠L = ∠M (each 90°)
∴ ∆ALO ~ ∆DMO [AA similarity criterion]
∴ \(\frac{\mathrm{AL}}{\mathrm{DM}}=\frac{\mathrm{AO}}{\mathrm{DO}}\) ……………(1)
[If two triangles are similar, corrosponding sides are proportional

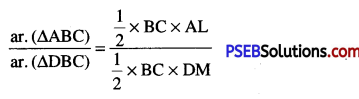
[∵ ∆ = \(\frac{1}{2}\) × b × p]

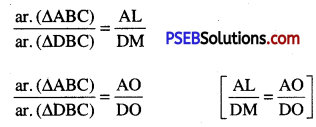
Hence proved.

Question 4.
If the areas of two similar triangles are equal, prove that they are congruent.
Solution:
Given: Two ∆s ABC and DEF are similar and equal in area.
To Prove : ∆ABC ≅ ∆DEF

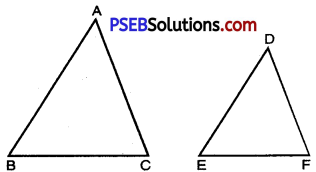
Proof: Since ∆ABC ~ ∆DEF,
∴ \(\frac{\text { area }(\Delta \mathrm{ABC})}{\text { area }(\Delta \mathrm{DEF})}=\frac{\mathrm{BC}^{2}}{\mathrm{EF}^{2}}\)

⇒ \(\frac{\mathrm{BC}^{2}}{\mathrm{EF}^{2}}=1\) [∵ area (∆ABC) = area (∆DEF)]
⇒ BC² = EF²
⇒ BC = EF.
Also, since ∆ABC ~ ∆DEF,
therefore they are equiangular and hence
∠B = ∠E
and ∠C = ∠F.
Now in ∆s ABC and DEF,
∠B = ∠E, ∠C = ∠F
and BC = EF
∴ ∆ABC ≅ ∆DEF (ASA congruence).

Question 5.
D, E and F are respectively the mid points of the sides BC, CA and AB of ∆ABC. Determine the ratio of the areas of triangles DEF and ABC.
Solution:

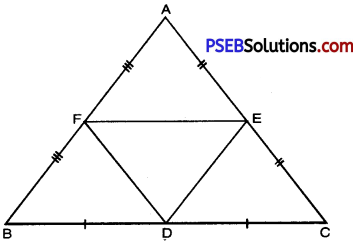
Given. D, E, F are the mid-point of the sides BC, CA and AB respectively of a tABC.
To find : ar (∆DEE) : ar (∆ABC)
Proof: In ∆ABC,
F is the mid-point of AB …(given)
E is the mid-point of AC …(given)
So, by the Mid-Foint Theorem
FE || BC and FE = \(\frac{1}{2}\) BC
⇒ FE || BD and FE = BD [∵ BD = \(\frac{1}{2}\) BC]
∴ BDEF is a || gm.
(∵ Opp. sides are || and equal)
In △s FBD and DEF,
FB = DE (opp. sides of || gm BDEF)
FD = FD .. .(common)
BD = FE
. ..(opp. sides of || gm BDEF)
∴ △FBD ≅ △DEF … (SSS Congruency Theorem)

Similary we can prove that:
△AFE ≅ △DEF
and △EDC ≅ △DEF
if △s are , then they are equal in area.
∴ ar (∆FBD) = ar. (∆DEF) ……………(1)
ar (∆AFE) = ar (∆DEF) ……………(2)
ar (∆EDC) = ar (∆DEF) ……………(3)
Now ar ∆ (ABC)
= ar (∆FBD) + ar (∆DEF) + ar (∆AFE) + ar (∆EDC)
= ar.(∆DEF) + ar (∆DEF) + ar (∆DEF) + ar. (∆DEF) [Using (1), (2) and (3)]
= 4 ar (∆DEF)
⇒ (∆DEF) = \(\frac{1}{4}\) ar(∆ABC)
⇒ \(\frac{{ar} .(\Delta \mathrm{DEF})}{{ar} .(\Delta \mathrm{ABC})}=\frac{1}{4}\)
∴ ar (∆DEF) : ar (∆ABC) = 1 : 4.

Question 6.
Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
Solution:
Given: ∆ABC ~ ∆DEF.
AX and DY are the medians to the side BC and EF respectively.

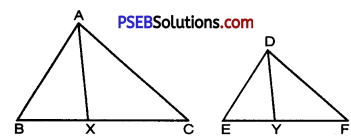
To prove: \(\frac{{ar} .(\Delta \mathrm{ABC})}{{ar} .(\Delta \mathrm{DEF})}=\frac{\mathrm{AX}^{2}}{\mathrm{DY}^{2}}\)
Proof: ∆ABC ~ ∆DEF (Given)
∴ \(\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{BC}}{\mathrm{EF}}=\frac{2 \mathrm{BX}}{2 \mathrm{EY}}\)
[∵ AX and DY are medians
∴ BC = 2BX and EF = 2EY]

⇒ \(\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{BX}}{\mathrm{EY}}\) ………………(1)
In ∆ABX and ∆DEY, [∵ ∆ABC ~ ∆DEF]
\(\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{BX}}{\mathrm{EY}}\) [Prove in (1)]
∴ ∆ABC ~ ∆DEY [By SAS criterion of similarity]
∴ \(\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{AX}}{\mathrm{DY}}\) …………(2)
As the areas of two similar triangles are proportional to the squares of the corresponding sides, so
∴ \(\frac{{ar} .(\Delta \mathrm{ABC})}{{ar} .(\Delta \mathrm{DEF})}=\frac{\mathrm{AB}^{2}}{\mathrm{DE}^{2}}=\frac{\mathrm{AX}^{2}}{\mathrm{D} \mathrm{Y}^{2}}\)
Hence proved.

Question 7.
Prove that the areas of the equilateral triangle described on the side of a square Is half the area of the equilateral triangle described on its diagonal.
Solution:
Given: ABCD is a square. Equilateral ∆ABE is described on the side AB of the square and equilateral ∆ACF is desribed on the diagonal AC.

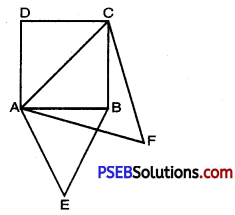
To prove: \(\frac{{ar} .(\Delta \mathrm{ABE})}{{ar} .(\Delta \mathrm{ACF})}=\frac{1}{2}\)
Proof: In rt. ∆ABC,
⇒ AB² + BC² = AC² [By Pathagoras theorem]
= AB² + AB² = AC² [∵ AB = BC, being the sides of the same square]
⇒ 2AB² = AC² ………….(1)
Now each of ∆ABE and ∆ACF are equilateral and therefore equiangular and hence similar.
i.e., ∆ABE ~ ∆ACF.
Here any side of one ∆ is proportional to any side of other.
∴ \(\frac{\text { ar. }(\Delta \mathrm{ABE})}{\text { ar. }(\Delta \mathrm{ACF})}=\frac{\mathrm{AB}^{2}}{\mathrm{AC}^{2}}\)

[∵ The ratio of the areas of two similar∆s is equal to their corresponding sides]
= \(\frac{\mathrm{AB}^{2}}{2 \mathrm{AB}^{2}}=\frac{1}{2}\) [Using (1)]

Question 8.
Tick the correct answer and justify: ABC and BDE are two equilateral triangles such that D is the mid point of BC. Ratio of the areas of triangles ABC and BDE is
(A) 2 : 1
(B) 1 : 2
(C) 4 : 1
(D) 1 : 4.
Solution:
∆ABC and ∆BDE are two equilateral thangles. D is mid point of BC.
∴ BD = DC = \(\frac{1}{2}\) BC,
Let each side of triangles are 2a
∴ ∆ABC ~ ∆BDE
∴ \(\frac{\text { ar. }(\Delta \mathrm{ABC})}{\text { ar. }(\triangle \mathrm{BDE})}=\frac{\mathrm{AB}^{2}}{\mathrm{BD}^{2}}\)

= \(\frac{(2 a)^{2}}{(a)^{2}}\)
= \(\frac{4 a^{2}}{a^{2}}\)
= \(\frac{4}{1}\) = 4 : 1
∴ Correct option is (C).

Question 9.
Tick the correct answer and justify: Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are ¡n the ratio
(A) 2 : 3
(B) 4 : 9
(C) 81 : 16
(D) 16 : 81.
Solution:

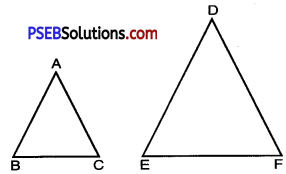
∆ABC ~ ∆DEF (given)

\(\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{AC}}{\mathrm{DF}}=\frac{\mathrm{BC}}{\mathrm{EF}}=\frac{4}{9}\)

∴ \(\frac{\text { ar. }(\Delta \mathrm{ABC})}{\text { ar. }(\Delta \mathrm{DEF})}=\frac{\mathrm{AB}^{2}}{\mathrm{DE}^{2}}\)

[If two triangles are similar ratio of their areas is equal to square of corresponding sides]
\(\frac{{ar} .(\Delta \mathrm{ABC})}{{ar} .(\Delta \mathrm{DEF})}=\left(\frac{4}{9}\right)^{2}\) = \(\frac{16}{81}\) = 16 : 81
∴ Correct option is (D).