PSEB 11th Class Maths Solutions Chapter 3 Trigonometric Functions Ex 3.3

Punjab State Board PSEB 11th Class Maths Book Solutions Chapter 3 Trigonometric Functions Ex 3.3 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.3

Question 1.
Prove that: sin² \(\frac{\pi}{6}\) + cos² \(\frac{\pi}{3}\) – tan² \(\frac{\pi}{4}\) = – \(\frac{1}{2}\)
Answer.
L.H.S.= sin² \(\frac{\pi}{6}\) + cos² \(\frac{\pi}{3}\) – tan² \(\frac{\pi}{4}\)

= \(\left(\frac{1}{2}\right)^{2}+\left(\frac{1}{2}\right)^{2}\) – (1)²

= \(\frac{1}{4}+\frac{1}{4}-1=-\frac{1}{2}\)

= R.H.S.
Hence proved.

Question 2.
Prove that: 2 sin² \(\frac{\pi}{6}\) + cosec² \(\frac{7 \pi}{6}\) cos² \(\frac{\pi}{3}\) = \(\frac{3}{2}\)
Ans.

Question 3.
Prove that :cot² \(\frac{\pi}{6}\) + cosec \(\frac{5 \pi}{6}\) + 3 tan² \(\frac{\pi}{6}\) = 6
Answer.

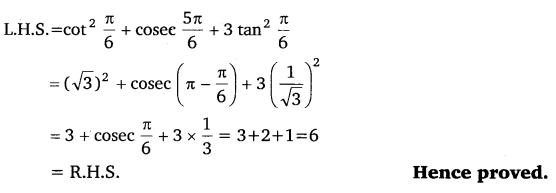
Question 4.
Prove that: 2 sin² \(\frac{3 \pi}{4}\) + 2 cos² \(\frac{\pi}{4}\) + 2 sec² \(\frac{\pi}{3}\) = 10
Answer.
L.H.S = \(2 \sin ^{2} \frac{3 \pi}{4}+2 \cos ^{2} \frac{\pi}{4}+2 \sec ^{2} \frac{\pi}{3}\)

= \(2\left\{\sin \left(\pi-\frac{\pi}{4}\right)\right\}^{2}+2\left(\frac{1}{\sqrt{2}}\right)^{2}+2(2)^{2}\)

= \(2\left\{\sin \frac{\pi}{4}\right\}^{2}+2 \times \frac{1}{2}+8\)

= 2 \(\left(\frac{1}{\sqrt{2}}\right)^{2}\) + 1 + 8

= 1 + 1 + 8
= 10 = R.H.S.
Hence proved.

Question 5.
Find the value of: (i) sin 75°,
(ii) tan 15°
Answer.
(i) sin 75° sin (45° + 30°)
= sin 45° cos 30° + cos 45° sin 30°
[∵ sin (x + y) = sin x cos y + cos x sin y]
= \(\left(\frac{1}{\sqrt{2}}\right)\left(\frac{\sqrt{3}}{2}\right)+\left(\frac{1}{\sqrt{2}}\right)\left(\frac{1}{2}\right)\)

= \(\frac{\sqrt{3}}{2 \sqrt{2}}+\frac{1}{2 \sqrt{2}}=\frac{\sqrt{3}+1}{2 \sqrt{2}}\)

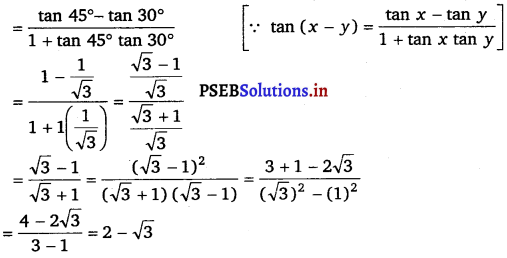
(ii) tan 15° = tan (45° – 30°)

Question 6.
\(\cos \left(\frac{\pi}{4}-x\right) \cos \left(\frac{\pi}{4}-y\right)-\sin \left(\frac{\pi}{4}-x\right) \sin \left(\frac{\pi}{4}-y\right)\) = sin (x + y)
Answer.

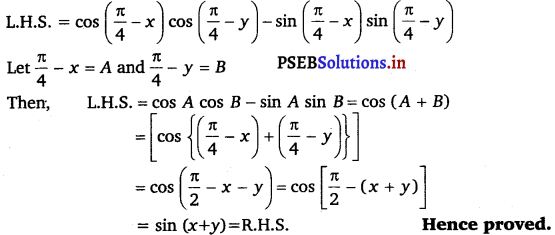
Question 7.
Prove that: \(\frac{\tan \left(\frac{\pi}{4}+x\right)}{\tan \left(\frac{\pi}{4}-x\right)}=\left(\frac{1+\tan x}{1-\tan x}\right)^{2}\)
Answer.

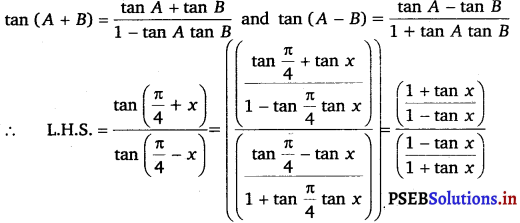
= \(\left(\frac{1+\tan x}{1-\tan x}\right)^{2}\)

= R.H.S
Hence proved.

Question 8.
Prove that: \(\frac{\cos (\pi+x) \cos (-x)}{\sin (\pi-x) \cos \left(\frac{\pi}{2}+x\right)}\) = cot² x
Answer.
L.H.S = \(\frac{\cos (\pi+x) \cos (-x)}{\sin (\pi-x) \cos \left(\frac{\pi}{2}+x\right)}\)

= \(\frac{[-\cos x][\cos x]}{(\sin x)(-\sin x)}=\frac{-\cos ^{2} x}{-\sin ^{2} x}\)

= cot² x

= R.H.S
Hence proved.

Question 9.
= 1
Answer.

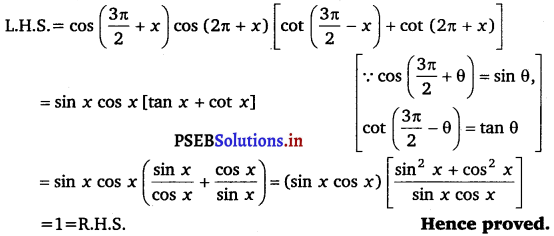
Question 10.
Prove that: sin (n + 1) x sin (n + 2) x + cos (n + 1) x cos (n + 2) x = cos x
Answer.
L.H. S. = sin (n + 1 )x sin (n + 2) x + cos (n +1) x cos (n + 2) x
[By the formula, cos (A – B) = cos A cos B + sin A sin B]
= cos [(n + 2) x + (n + 1) x]
= cos (4x + 2x – 4x – x)
= cos x = R.H.S.
Hence proved.

Question 11.
Prove that: \(\cos \left(\frac{3 \pi}{4}+x\right)-\cos \left(\frac{3 \pi}{4}-x\right)=-\sqrt{2} \sin x\)
Answer.
It is known that
cos A – cos B = \(-2 \sin \left(\frac{A+B}{2}\right) \cdot \sin \left(\frac{A-B}{2}\right)\)

∴ L.H.S.= \(=\cos \left(\frac{3 \pi}{4}+x\right)-\cos \left(\frac{3 \pi}{4}-x\right)\)

= \(– 2 \sin \left\{\frac{\left(\frac{3 \pi}{4}+x\right)+\left(\frac{3 \pi}{4}-x\right)}{2}\right\} \cdot \sin \left\{\frac{\left(\frac{3 \pi}{4}+x\right)-\left(\frac{3 \pi}{4}-x\right)}{2}\right\}\)

= – 2 sin (\(\frac{3 \pi}{4}\)) sin x

= – 2 sin (- \(\frac{\pi}{4}\)) sin x

= – √2 sin x = R.H.S.
Hence proved.

Question 12.
Prove that: sin² 6x – sin² 4x = sin 2x sin 10 x
Answer.
It is known that
sin A + sin B = 2 \(\sin \left(\frac{A-B}{2}\right) \cos \left(\frac{A-B}{2}\right)\)

sin A – sin B = 2 \(\cos \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)\)
L.H.S.= sin² 6x – sin² 4x
= (sin 6x + sin 4x) (sin 6x – sin 4x)
= (2 sin 5x cos x) (2 cos 5x sin x)
= (2 sin 5x cos 5x) (2 sin x cos x)
= sin 10x sin 2x = R.H.S.
Hence proved.

Question 13.
Prove that: cos² 2x cos² 6x = sin 4x sin 8x
Answer.
It is known that
cos A + cos B = 2 \(\cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)\)

cos A – cos = 2 \(\sin \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)\)

∴ L.H.S = cos² 2x – cos² 6x
= (cos 2x + cos 6x) (cos 2x – 6x)
= \(\left[2 \cos \left(\frac{2 x+6 x}{2}\right) \cos \left(\frac{2 x-6 x}{2}\right)\right]\left[-2 \sin \left(\frac{2 x+6 x}{2}\right) \sin \frac{(2 x-6 x)}{2}\right]\)
∴ L.H.S.= cos² 2x – cos² 6x
= (cos 2x + cos 6x) (cos 2x – 6x)
= [2 cos 4x cos (-2x)] [- 2 sin 4x sin (- 2x)]
= [2 cos 4x cos 2x] [- 2 sin 4x (- sin 2x)]
= (2 sin 4x cos 4x) (2 sin 2x cos 2x)
= sin 8x sin 4x
= R.H.S.
Hence proved.

Question 14.
Prove that: sin 2x + 2 sin 4x + sin 6x = 4 cos² x sin 4x
Answer.
L.H.S.= sin 2x + 2 sin 4x + sin 6x
= [sin 2x + sin 6x] + 2 sin 4x
= \(\left[2 \sin \left(\frac{2 x+6 x}{2}\right) \cos \left(\frac{2 x-6 x}{2}\right)\right]\) + 2 sin 4x
[∵ sin A + sin B = 2 \(\sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)\)]
= 2 sin 4x cos (- 2x) + 2 sin 4x
= 2 sin 4x cos 2x + 2 sin 4x
= 2 sin 4x (cos 2x + 1)
= 2 sin 4x (2 cos² x – 1 + 1)
= 2 sin 4x (2 cos² x)
= 4 cos² x sin 4x
= R.H.S.
Hence proved.

Question 15.
cot 4x (sin 5x + sin 3x) = cot x (sin 5x – sin 3x)
Answer.

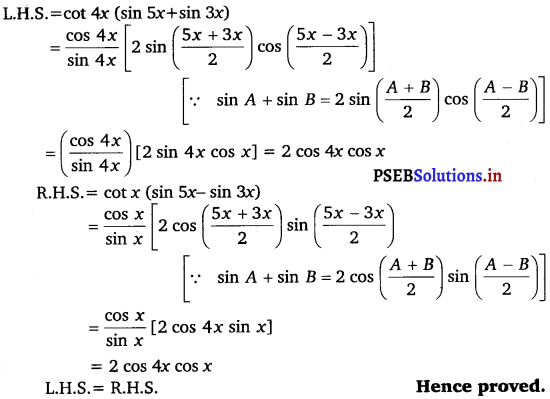
Question 16.
Prove that: \(\frac{\cos 9 x-\cos 5 x}{\sin 17 x-\sin 3 x}=-\frac{\sin 2 x}{\cos 10 x}\)
Answer.

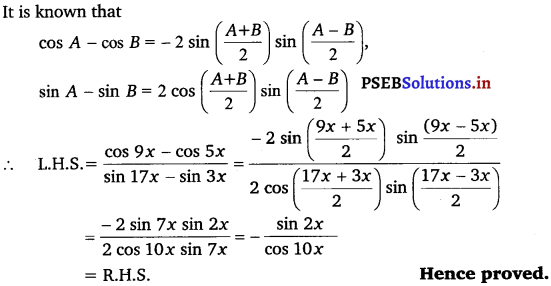
Question 17.
Prove that: \(\frac{\sin 5 x+\sin 3 x}{\cos 5 x+\cos 3 x}\) = tan 4x
Answer.
It is known that
sin A + sin = 2 \(\sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)\)

cos A + cos = 2 \(\cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)\)

∴ L.H.S = \(\frac{\sin 5 x+\sin 3 x}{\cos 5 x+\cos 3 x}\)

= \(\frac{2 \sin \left(\frac{5 x+3 x}{2}\right) \cos \left(\frac{5 x-3 x}{2}\right)}{2 \cos \left(\frac{5 x+3 x}{2}\right) \cos \left(\frac{5 x-3 x}{2}\right)}\)

= \(\frac{2 \sin 4 x \cos x}{2 \cos 4 x \cos x}=\frac{\sin 4 x}{\cos 4 x}\)

= tan 4x = R.H.S.
Hence proved.

Question 18.
Prove that: \(\frac{\sin x-\sin y}{\cos x+\cos y}=\tan \frac{x-y}{2}\).
Answer.

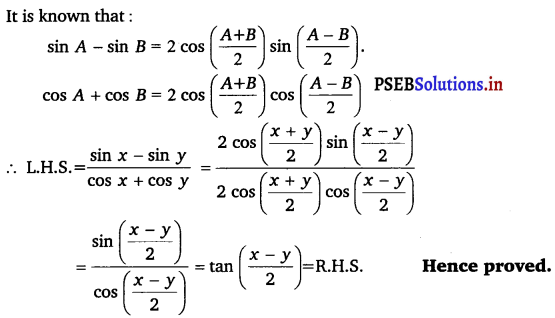
Question 19.
Prove that: \(\frac{\sin x+\sin 3 x}{\cos x+\cos 3 x}\) = tan 2x
Answer.

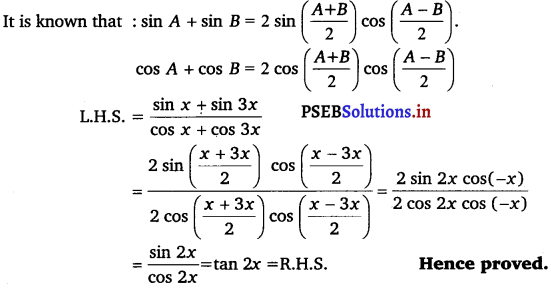
Question 20.
Prove that: \(\frac{\sin x-\sin 3 x}{\sin ^{2} x-\cos ^{2} x}\) = 2 sin x
Answer.
It is known that
sin A – sin B = 2 \(\cos \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)\)

cos² A – sin² A = cos 2A

∴ L.H.S. = \(=\frac{\sin x-\sin 3 x}{\sin ^{2} x-\cos ^{2} x}\)

= \(\frac{2 \cos \left(\frac{x+3 x}{2}\right) \sin \left(\frac{x-3 x}{2}\right)}{-\cos 2 x}\)

= \(\frac{2 \cos 2 x \sin (-x)}{-\cos 2 x}\)

= – 2 × (- sin x) = 2 sin x

= R.H.S

Hence proved.

Question 21.
Prove that: \(\frac{\cos 4 x+\cos 3 x+\cos 2 x}{\sin 4 x+\sin 3 x+\sin 2 x}\) = cot 3x
Answer.

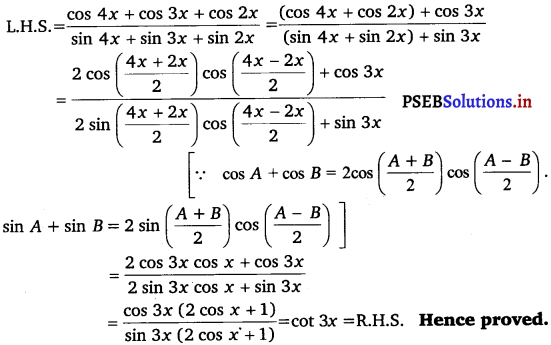
Question 22.
Prove that : cot x cot 2x – cot 2x cot 3x – cot 3x cot x = 1
Answer.
L.H.S.= cot x cot 2x – cot 2x cot 3x – cot 3x cot x
= cot x cot 2x – cot 3x (cot 2x + cot x)
= cot x cot 2x – cot (2x + x) (cot 2x + cot x)
= cot x cot 2x – \(\left[\frac{\cot 2 x \cot x-1}{\cot x+\cot 2 x}\right]\) (cot 2x + cot x)
[∵ cot (A + B) = \(\frac{\cot A \cot B-1}{\cot A+\cot B}\)]
= cot x cot 2x – (cot 2x cot x – 1)
= 1 = R.H.S.
Hence proved.

Question 23.
Prove that: tan 4x = \(\frac{4 \tan x\left(1-\tan ^{2} x\right)}{1-6 \tan ^{2} x+\tan ^{4} x}\).
Answer.
It is known that:
tan 2A = \(\frac{2 \tan A}{1-\tan ^{2} A}\)

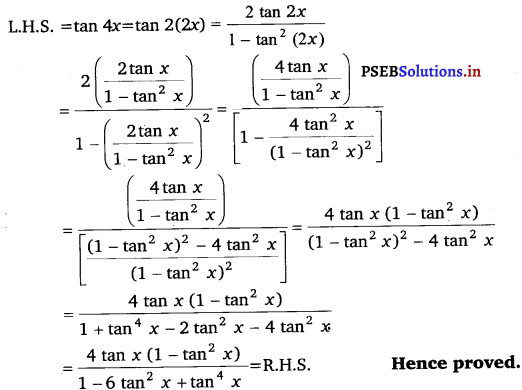
Question 24.
Prove that: cos 4x = 18 sin² x cos² x
Answer.
L.H.S. = cos 4x = cos 2(2x)
= 1 – 2 sin² 2x [∵ cos 2A = 1 – 2 sin² A]
= 1 – 2(2 sin x cos x)² [∵ sin 2A = 2 sin A cos A]
= 1 – 8 sin² x cos² x
= R.H.S.
Hence proved.

Question 25.
Prove that: cos 6x = 32 cos⁶ x – 48 cos⁴ x + 18 cos² x – 1
Answer.
We know that: cos 3x = 4 cos³ x – 3cos x
On replacing x by 2x, we get
cos 3(2x) = 4 cos³ (2x) – 3 cos 2x
⇒ cos 6x = 4 (2cos² x – 1)³ – 3 (2cos² x – 1)
[∵ cos 2x = 2cos² x – 1]
= 4 [8 cos⁶ x – 12 cos⁴ x + 6 cos² x – 1] – 6 cos² x + 3
[∵ (a – b)³ = a³ – 3a²b + 3ab² – b³]
= 32 cos⁶ x – 48 cos⁴ x + 24 cos² x – 4 – 6 cos² x + 3
⇒ cos 6x = 32 cos⁶ x – 48 cos⁴ x + 18 cos² x – 1
Hence proved.