Punjab State Board PSEB 11th Class Maths Book Solutions Chapter 6 Linear Inequalities Ex 6.3 Textbook Exercise Questions and Answers.
PSEB Solutions for Class 11 Maths Chapter 6 Linear Inequalities Ex 6.3
Question 1.
Solve the following system of inequalities graphically:
x ≥ 3,
y ≥ 2
Answer.
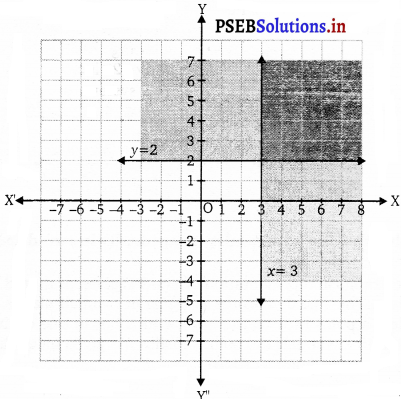
x ≥ 3 ……………(i)
y ≥ 2 ………….(ii)
The graph of the lines, x = 3 and y = 2, are drawn in the figure below.
Inequality (i) represents the region on the right hand side of the line, x = 3 (including the line x = 3) , and inequality (ii) represents the region above the line, y = 2 (including the line y = 2).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the respective lines as follows.
![]()
Question 2.
Solve the following system of inequalities graphically:
3x + 2y ≤ 12, x ≥ 1, y ≥ 2
Answer.
3x + 2y ≤ 12 …………..(i)
x > 1 ………….(ii)
y > 2 …………..(iii)
The graphs of the lines, 3x + 2y = 12, x = 1, and y = 2, are drawn in the figure below.
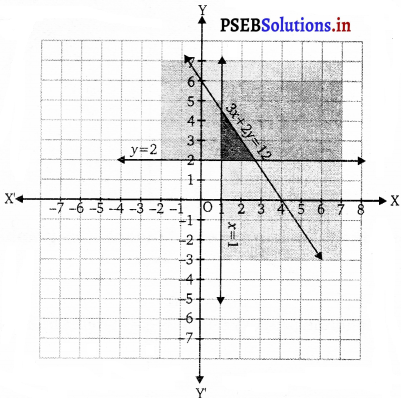
Inequality (i) represents the region below the line, 3x + 2y = 12 (including the line 3x + 2y = 12).
Inequality (ii) represents the region on the right side of the line, x = 1 (including the line x = 1).
Inequality (iii) represents the region above the line, y = 2 (including the line y = 2).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the respective lines as given figure.
Question 3.
Solve the following system of inequalities graphically :
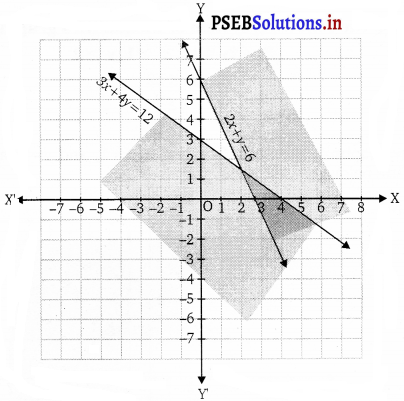
2x + y ≥ 6, 3x + 4y ≤ 12
Answer.
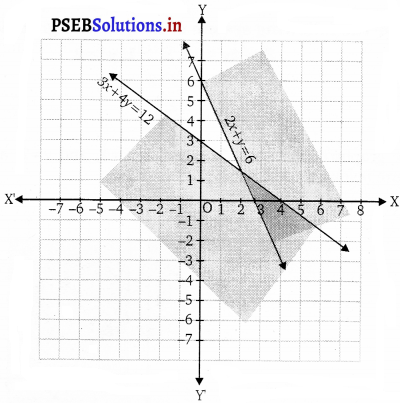
2x + y ≥ 6 …………..(i)
3x + 4y ≤ 12 ……………..(ii)
The graph of the lines, 2x + y = 6 and 3x + 4y = 12, are drawn in the figure below.
Inequality (i) represents the region above the line, 2x + y = 6 (including the line 2x + y = 6), and inequality (ii) represents the region below the line, 3x + 4y = 12 (including the line 3x + 4y = 12).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the respective lines as follows.
![]()
Question 4.
Solve the following system of inequalities graphically :
x + y ≥ 4, 2x – y > 0
Answer.
x + y ≥ 4 …………….(i)
2x – y > 0 …………….. (ii)
The graph of the lines, x + y = 4 and 2x – y = 0, are drawn in the figure below.
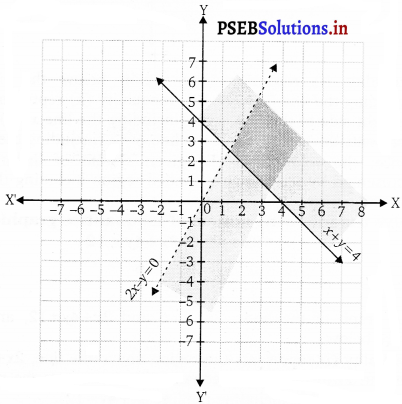
Inequality (i) represents the region above the line, x + y = 4 (including the line x + y = 4).
Inequality (i) represents the region above the line, x + y = 4 (including the line x + y = 4).
It is observed that (1, 0) satisfies the inequality, 2x – y > 0. [2(1) – 0 = 2 > 0]
Therefore, inequality (ii) represents the half plane corresponding to the line, 2x – y = 0, containing the point (1, 0) [excluding the line 2x – y > 0].
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on line x + y = 4 and excluding the points on line 2x – y = 0 as given figure.
![]()
Question 5.
Solve the following system of inequalities graphically:
2x – y > 1, x – 2y < – 1 Answer. 2x – y> 1 ………….(i)
x – 2y < – 1 …………….(ii)
The graph of the lines, 2x – y= 1 and x – 2y = – 1, are drawn in the figure below.
Inequality (i) represents the region below the line, 2x – y = 1 (excluding the line 2x – y = 1), and inequality (ii) represents the region above the line, x – 2y = – 1 (excluding the line x – 2y = – 1).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region excluding the points on the respective lines as follows.

![]()
Question 6.
Solve the following system of inequalities graphically : x + y ≤ 6, x + y > 4
Answer.
x + y ≤ 6 ……………..(i)
x + y ≥ 4 …………..(ii)
The graph of the lines, x + y = 6 and x + y = 4, are drawn in the figure below.
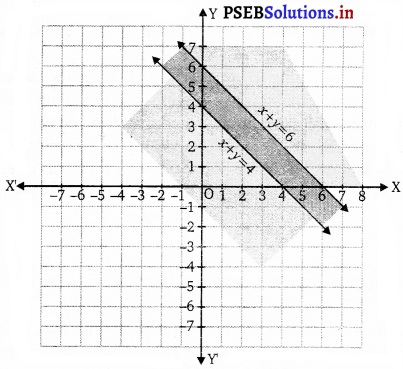
Inequality (i) represents the region below the line, x + y = 6 (including the line x + y = 6), and inequality (ii) represents the region above the line, x + y = 4 (including the line x + y = 4).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the respective lines as given in figure.
Question 7.
Solve the following system of inequalities graphically:
2x + y ≥ 8, x + 2y ≥ 10
Answer.
2x + y ≥ 8 …………..(i)
x + 2y ≥ 10 ……………(ii)
The graph of the lines, 2x + y = 8 and x + 2y = 10, are drawn in the figure below.
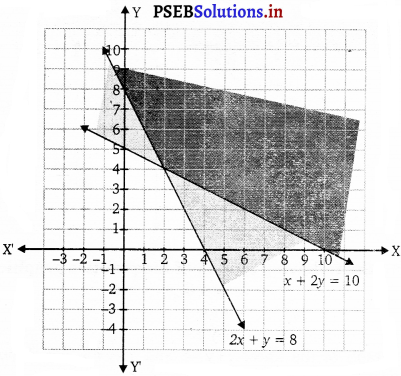
Inequality (i) represents the region above the line, 2x + y = 8, and inequality (ii) represents the region above the line, x + 2y = 10.
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the respective lines as given in figure.
![]()
Question 8.
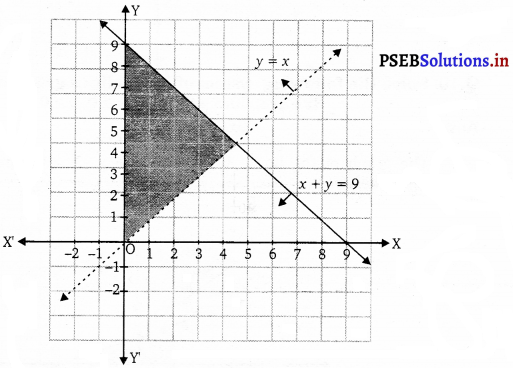
Solve the following system of inequalities graphically: x + y ≤ 9, y > x, x ≥ 0.
Answer.
x+y ≤ 9 ………..(i)
y > x …………..(ii)
x ≥ 0 ……………(iii)
The graph of the lines, x + y = 9 and y = x, are drawn in the figure below.
Inequality (i) represents the region below the line, x + y = 9 (including the line x + y = 9).
It is observed that (0, 1) satisfies the inequality, y > x. [1 > 0].
Therefore, inequality (ii) represents the half plane corresponding to the line, y = x, containing the point (0, 1) [excluding the line y = x].
Inequality (iii) represents the region on the right hand side of the line, x = 0 or y – axis (including y – axis).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the lines, x + y = 9 and x = 0, and excluding the points on line y = x as follows.
Question 9.
Solve the following system of inequalities graphically:
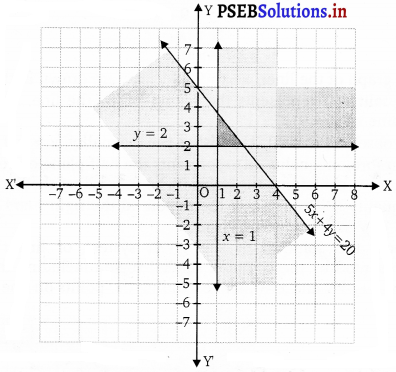
5x + 4y ≤ 20, x ≥ 1, y ≥ 2
Answer.
5x + 4y ≤ 20 …………..(i)
x ≥ 1 ………………(ii)
y ≥ 2 ……………(iii)
The graph of the lines, 5x + 4y = 20, x = 1, and y = 2, are drawn in the figure below.
Inequality (i) represents the region below the line, 5x + 4y = 20 (including the line 5x + 4y = 20).
Inequality (ii) represents the region on the right hand side of the line, x = 1 (including the line x = 1).
Inequality (iii) represents the region above the line, y = 2 (including the line y = 2).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the respective lines as follows.
![]()
Question 10.
Solve the following system of inequalities graphically:
3x + 4y ≤ 60, x + 3y ≤ 30, x ≥ 0, y ≥ 0
Answer.
3x + 4y ≤ 60 …………. (i)
x + 3y ≤ 30 ………….(ii)
The graph of the lines, 3x + 4y = 60 and x + 3y = 30, are drawn in the figure below.
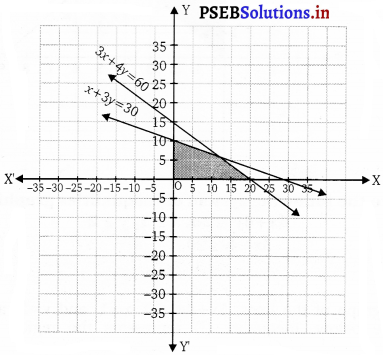
Inequality (i) represents the region below the line, 3x + 4y = 60
(including the line 3x + 4y = 60), and inequality (ii) represents the region below the line, x + 3y = 30 (including the line x + 3y = 30).
Since x > 0 and y > 0, every point in the common shaded region in the first quadrant including the points on the respective line and the axes represents the solution of the given system of linear inequalities.
![]()
Question 11.
Solve the following system of inequalities graphically :
2x + y ≥ 4, x + y ≤ 3, 2x – 3y ≤ 6
Answer.
2x + y ≥ 4 ………….(i)
x + y ≤ 3 …………..(ii)
2x – 3y ≤ 6 …………….(iii)
The graph of the lines, 2x + y = 4, x + y = 3, and 2x – 3y = 6, are drawn in the figure below.
Inequality (i) represents the region above the line, 2x + y = 4 (including the line 2x + y = 4).
Inequality (ii) represents the region below the line, x + y = 3 (including the line x + y = 3).
Inequality (iii) represents the region above the line, 2x – 3y = 6 (including the line 2x – 3y = 6).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the respective lines as follows.

Question 12.
Solve the following system of inequalities graphically:
x – 2y ≤ 3, 3x + 4y ≥ 12, x ≥ 0, y ≥ 1
Answer.
We have
x – 2y ≤ 3 …………(i)
3x + 4y ≥ 12 …………….(ii)
x ≥ 0 …………….(iii)
and y ≥ 1 ……………….(iv)
Take inequality (i), x – 2y ≤ 3
Corresponding equation of the line is,
x – 2y = 3
Table of values satisfying the equation
x – 2y = 3

Join the points (0, – 1.5) and (3, 0) by a dark line.
On putting (0, 0) in the given inequality (i),we get 0 ≤ 3, which is true.
so, half plane of x – 2y ≤ 3 contains the origin.
Take inequality (ii), 3x + 4y ≥ 12
Corresponding equation of the line is 3x + 4y =12.
Table of values satisfying the equation 3x + 4y = 12.

Join the points (0, 3) and (4, 0) by a dark line.
On putting (0, 0) in the inequality (ii), we get 0 ≥ 12, wrhich is false.
So, half plane 3x + 4y > 12 does not contain the origin.
Take inequality (iii) x ≥ 0, this implies, area shaded in I quadrant.
Take inequality (iv), y ≥ 1 Corresponding equation of the line is y =1.
On putting (0,0) in the given inequality (iv), we get 0 ≥ 1, which is false.
So, half plane of y ≥ 1 is does not contain the origin.
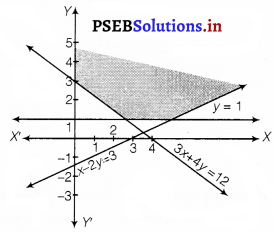
Hence, shade the common region which gives required solution set.
![]()
Question 13.
Solve the following system of inequalities graphically:
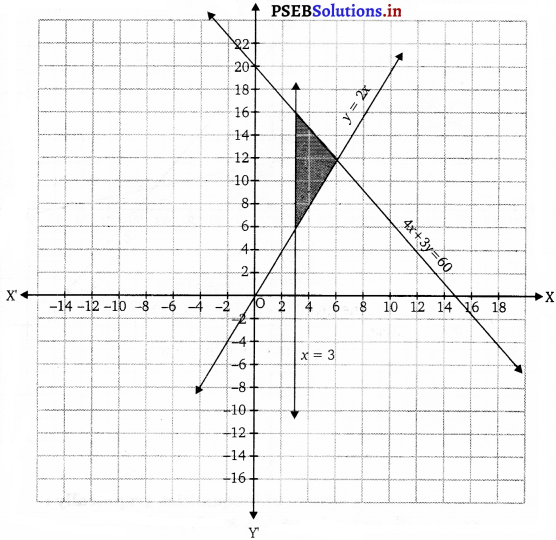
4x + 3y ≤ 60, y ≥ 2x, x ≥ 3, x, y ≥ 0
Answer.
4x + 3y ≤ 60 ………….(i)
y ≥ 2x ………….(ii)
x ≥ 3 …………(iii)
The graph of the lines, 4x + 3y = 60, y = 2x, and x = 3, are drawn in the figure below.
Inequality (i) represents the region below the line, 4x + 3y = 60 (including the line 4x + 3y = 60).
Inequality (ii) represents the region above the line, y = 2x (including the line y = 2x).
Inequality (iii) represents the region on the right hand side of the line, x = 3 (including the line x = 3).
Hence, the solution of the given system of linear inequalities is represented by the common shaded region including the points on the respective lines as follows.
![]()
Question 14.
Solve the following system of inequalities graphically:
3x + 2y ≤ 150, x + 4y ≤ 80, x ≤ 15, y ≥ 0, x ≥ 0
Answer.
3x + 2y ≤ 150 ……………….(i)
x + 4y ≤ 80 ………………(ii)
x ≤ 15 ……………….(iii)
The graph of the lines, 3x + 2y = 150, x + 4y = 80, and x = 15, are drawn in the figure below.
Inequality (i) represents the region below the line, 3x + 2y = 150 (including the line 3x + 2y = 150).
Inequality (ii) represents the region below the line, x+4y=80 (including the line x + 4y = 80).
Inequality (iii) represents the region on the left hand side of the line, x = 15 (including the line x = 15).
Since x ≥ 0 and y ≥ 0, every point in the common shaded region in the first quadrant including the points on the respective lines and the axes represents the solution of the given system of linear inequalities.
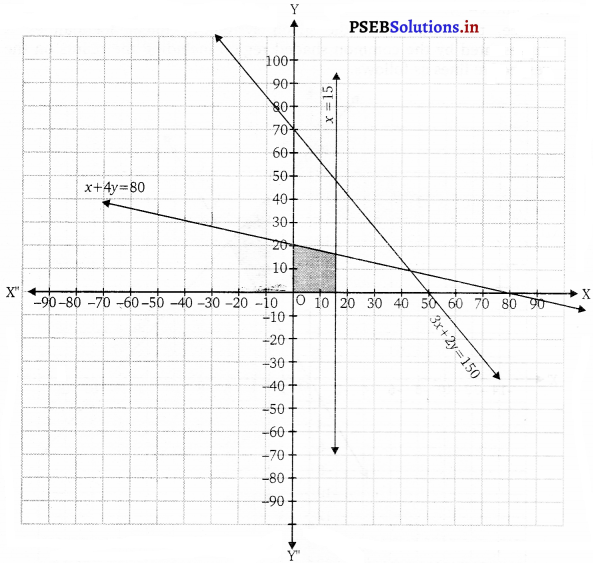
![]()
Question 15.
Solve the following system of inequalities graphically :
x + 2y ≤ 10, x + y > 1, x – y < 0, x > 0, y > 0
Answer.
x + 2y ≤ 10 …………(i)
x + y ≥ 1 ………….(ii)
x – y ≤ 0 ……………(iii)
The graph of the lines, x + 2y = 10, x + y = 1, and x – y = 0, are drawn in the figure below.
Inequality (i) represents the region below the line, x + 2y = 10 (including the line x + 2y = 10).
Inequality (ii) represents the region above the line, x + y = 1 (including the line x + y = 1).
Inequality (iii) represents the region above the line, x – y = 0 (including the line x – y = 0).
Since x > 0 and y > 0, every point in the common shaded region in the first quadrant including the points on the respective lines and the axes represents the solution of the given system of linear inequalities.

