Punjab State Board PSEB 10th Class Maths Book Solutions Chapter 14 Statistics Ex 14.4 Textbook Exercise Questions and Answers.
PSEB Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.4
Question 1.
The following distribution gives the daily income of 50 workers of a factory.
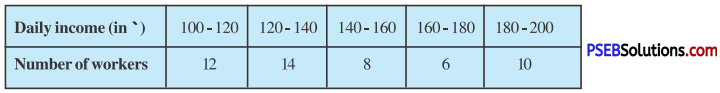
Convert the distribution above to a less than type cumulative frequency distribution and draw its ogive.
Solution:
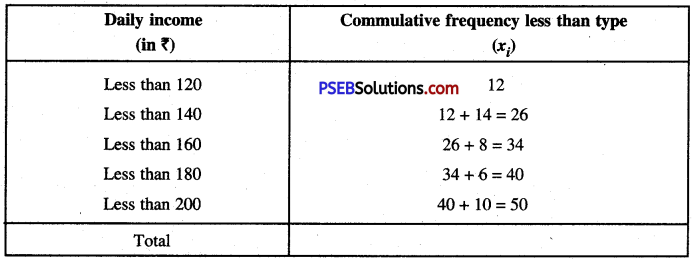
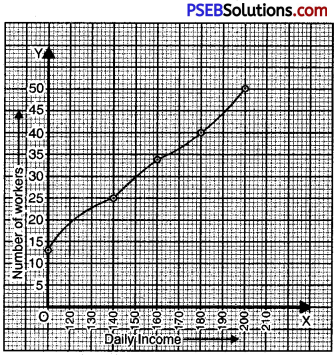
Now, by drawing the points on the graph
i.e. (120, 12); (140, 26); (160, 34); (180, 40); (200, 50).
We get graph of less than type cumulative frequency.
Scale chosen:
On x-axis 10 units = Rs. 10
On y-axis 10 units = 5 workers.
![]()
Question 2.
During the medial check up of 35 students of a class, their weights were recorded as follows:

Draw a less than type ogive for the given data. Hence obtain the median weight from the graph and verWy the result by using the formula.
Solution:

Now, By drawing the points on the graph i.e., (38, 0); (40, 3); (42, 5); (44, 9); (46, 14); (48, 28) ; (50, 32) ; (52, 35) we get graph of less than type cumulative frequency.
![]()
Scale Chosen:
On x-axis, 10 units = 2 kg
On y-axis units = 5 students
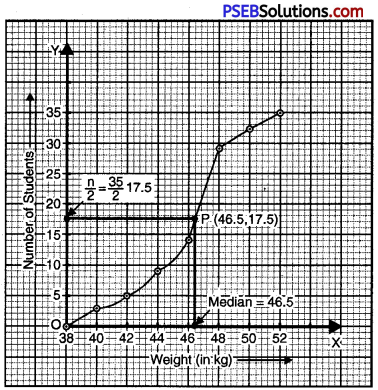
From above graph, it is clear that
Median = 46.5 kg ; which lies in class interval 46 – 48.
Now, in the given table
\(\Sigma f_{i}\) = n = 35
∴ \(\frac{n}{2}=\frac{35}{2}\) = 17.5 ; which lies in the interval 46 – 48.
∴ Median class = 46 – 48
So, l = 46; n = 35; f = 14; cf = 14 and h = 2
Using formula, Median = l + \(\left\{\frac{\frac{n}{2}-c f}{f}\right\}\) × h
Median = 46 + \(\left\{\frac{\frac{35}{2}-14}{14}\right\}\) × 2
= 46 + \(\left\{\frac{\frac{35-28}{2}}{14}\right\}\)
= 46 + \(\frac{7}{2} \times \frac{1}{14}\) = 46 + \(\frac{1}{2}\)
= 46 + 0.5 = 46.5
From above discussion and graph; it is clear that median is same in both cases. Hence, Median weight of students is 46.5 kg.
![]()
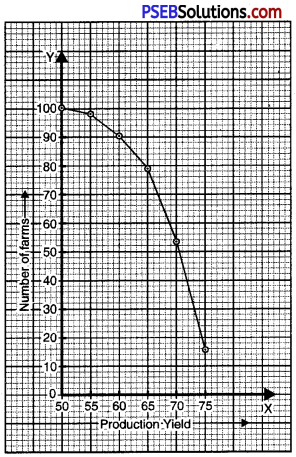
Question 3.
The following table gives production yield per hectare of wheat of 1(X) farms of a village.
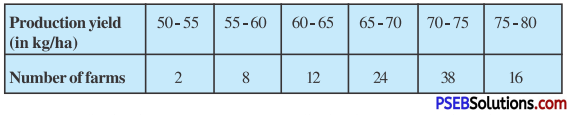
Change the distribution to a more than type distribution, and draw its ogive.
Solution:
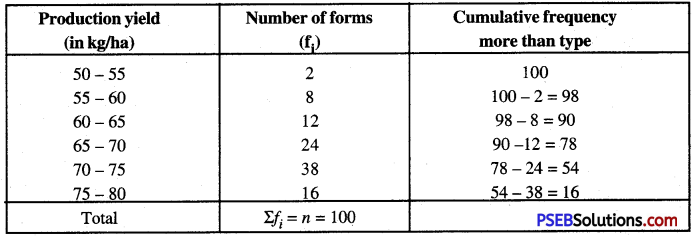
Now, by drawing the points on the graph i.e. (50, 100); (55, 98); (60, 90); (65, 78); (70, 54); (75, 16)
we get graph of more than type cumulative frequency.
Scale chosen:
On x-axis 10 units = 5 kg/ha
On y-axis 10 units = 10 forms