Punjab State Board PSEB 11th Class Maths Book Solutions Chapter 11 Conic Sections Ex 11.1 Textbook Exercise Questions and Answers.
PSEB Solutions for Class 11 Maths Chapter 11 Conic Sections Ex 11.1
Question 1.
Find the equation of the circle with centre (0, 2) and radius 2.
Answer.
The equation of a circle with centre (h, k) and radius r is given as
(x – h)2 + (y – k)2 = r2
It is given that centre (h, k) = (0, 2) and radius (r) = 2
Therefore, the equation of the circle is
(x – 0)2 + (y – 2)2 = 22
x2 + y2 + 4 – 4y = 4
x2 + y2 – 4y = 0.
![]()
Question 2.
Find the equation of the circle with centre (- 2, 3) and radius 4.
Answer.
The equation of a circle with centre (h, k) and radius r is given as
(x – h)2 + (y – k)2 = r2
It is given that centre (h, k) = (- 2, 3) and radius (r) = 4.
Therefore, the equation of circle is
(x + 2)2 + (y – 3)2 = (4)2
x2 + 4x + 4 + y2 – 6y + 9 = 16
x2 + y2 + 4x – 6y – 3 = 0.
Question 3.
Find the equation of the circle with centre (\(\frac{1}{2}\), \(\frac{1}{4}\)) and radius \(\frac{1}{12}\).
Answer.
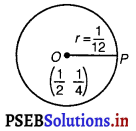

![]()
Question 4.
Find the equation of the circle with cehtre (1,1) and radius √2.
Answer.

Here, centre is at (1, 1).
∴ h = 1, k = 1
Also, radius, r = √2 units
[∵ equation of circle having centre (h, k) and radius r is (x – h)2 + (y – k)2 = r2].
Equation of circle is (x – 1)2 + (y – 1)2 = (V2)2
⇒ x2 – 2x + 1 + y2 – 2y + 1 = 2
⇒ x2 + y2 – 2x – 2y = 0
Question 5.
Find the equation of the circle with centre (- a, – b) and radius \(\sqrt{a^{2}-b^{2}}\).
Answer.
The equation of a circle with centre (h, k) and radius r is given as (x – h)2 + (y – k)2 = r2
It is given that centre (h, k) = (- a, – b) and radius (r) = \(\sqrt{a^{2}-b^{2}}\).
Therefore, the equation of the circle is (x + a)2 + (y + b)2 = (\(\sqrt{a^{2}-b^{2}}\))2
x2 + 2ax + a2 + y2 + 2by + b2 = a2 – b2
x2 + y2 + 2ax + 2by + 2b2 = 0.
Question 6.
Find the centre and radius of the circle (x + 5)2 + (y – 3)2 = 36.
Answer.
The equation of given circle is (x + 5)2 + (y – 3)2 = 36
(x + 5)2 + (y – 3)2 = 36
⇒ {x – (- 5)}2 + (y – 3)2 = (6)2,
which is of the form (x – h)2 + (y – k)2 = r2,
where h = – 5, k = 3, and r = 6.
Thus, the centre of the given circle is (- 5, 3), while its radius is 6.
![]()
Question 7.
Find the equation of the circle x2 + y2 – 4x – 8y – 45 = 0.
Answer.
The equation of given circle is x2 + y2 – 4x – 8y – 45 = 0.
x2 + y2 – 4x – 8y – 45 = 0
⇒ (x2 – 4x) + (y2 – 8y) = 45
⇒ {x2 – 2 (x) (2) + 22} + {y2 – 2(y) (4) + 42} – 4 – 16 = 45
⇒ (x – 2)2 + (y – 4)2 = 65 ,
⇒ (x – 2)2 + (y – 4)2 = (√65)2, which is of the form
(x – h)2 + (y – k)2 = r2 , where h = 2, k = 4 and r = √65
Thus, the centre of the given circle is (2, 4), while its radius is √65.
Question 8.
Find the centre and radius of the circle x2 + y2 – 8x + 10y – 12 = 0.
Answer.
The equation of the given circle is x2 + y2 + 8x + 10y – 12 = 0.
x2 + y2 – 8x + 10y – 12 = 0
(x2 – 8x) + (y2 + 10y) = 12
⇒ (x2 – 2 (x) (4) + 42) + (y2 + 2(y) (5) + 52) – 16 – 25 = 12
(x – 4)2 + (y + 5)2 = 53
(x – 4)2 + {y – (- 5)}2 = (√53)2, which is of the form
(x – h)2 + (y – k)2 = r2, where h = 4, k = – 5 and r = √53
Thus, the centre of the given circle is (2, 4), while its radius is √53.
![]()
Question 9.
Find the centre and radius of the circle 2x2 + 2y2 – x = 0.
Answer.
The given equation of circle is
2x2 + 2y2 – x = 0
x2 + y2 – \(\frac{x}{2}\) = 0
(x2 – \(\frac{x}{2}\)) + y2 = 0
On adding \(\frac{1}{16}\) to make perfect squares, we get
(x2 – \(\frac{x}{2}\) + \(\frac{1}{16}\)) + y2 = \(\frac{1}{16}\)
(x – \(\frac{1}{4}\))2 + (y – 0)2 = (\(\frac{1}{4}\))2
On comparing with (x – h)2 + (y – k)2 = r2 , we get
h = \(\frac{1}{4}\), k = 0 and r = \(\frac{1}{2}\)
Centre = (h, k) = (\(\frac{1}{4}\), 0)
Radius = \(\frac{1}{4}\)
Question 10.
Find the equation of the circle passing through the points (4, 1) and (6, 5) and whose centre is on the line 4x + y = 16.
Answer.
Let the equation of the required circle be (x – h)2 + (y – k)2 = r2.
Since the circle passes through points (4, 1) and (6, 5).
(4 – h)2 + (1 – k)2 = r2 ……………(i)
(6 – h)2 + (5 – k)2 = r2 ……………(ii)
Since the centre (h, k) of the circle lies on line 4x + y = 16,
4h + k = 16
From equations (i) and (ii), we obtain
(4 – h)2 + (1 – k)2 = (6 – h)2 + (5 – k)2
⇒ 16 – 8h + h2 + 1 – 2k + k2 = 36 – 12h + h2 + 25 – 10k + k2
⇒ 16 – 8h + 1 – 2k = 36 – 12h + 25 – 10k
⇒ 4h + 8k = 44
⇒ h + 2k = 11 …………………(iv)
On solving equations (iii) and (iv), we obtain h = 3 and k = 4.
On substituting the values of h and k in equation (i), we obtain
(4 – 3)2 + (1 – 4)2 = r2
⇒ (1)2 + (- 3)2 = r2
⇒ 1 + 9 = r2
⇒ r2 = 10
⇒ r = √10
Thus, the equation of the required circle is (x – 3)2 + (y – 4)2= (√10)2
x2 – 6x + 9 + y2 – 8y + 16 = 10
x2 + y2 – 6x – 8y + 15 = 0.
![]()
Question 11.
Find the equation of the circle passing through the points (2, 3) and (- 1, 1) and whose centre is on the line x – 3y – 11 = 0.
Answer.
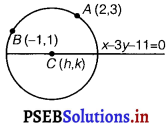
Let the equation of the circle be
(x -h)2 + (y – k)2 =r2 ……………(i)
Since, the circle (i) passes through (2, 3) and (- 1, 1).
We have, (2 – h)2 + (3 – k)2 = r2
⇒ h2 – 4h + 4 + 9 – 6k + k2 = r2 and
(- 1 – h)2 + (1 – k)2 = r2
h2 + 2h + 1 + 1 – 2k + k2 = r2
Centre (h, k) of circle is on the line
x – 3y – 11 = 0
∴ h – 3k – 11 = 0 …………..(iv)
On subtracting eQuestion (iii) from eq.(ii), we get
– 6h – 4k + 11 = 0
⇒ 6h + 4k = 11 ……………….(v)
On solving eqs. (iv) and (v), we get
h = \(\frac{7}{2}\), k = \(\frac{-5}{2}\)
On putting the values of h and k in eq. (ii), we get
(2 – \(\frac{7}{2}\))2 + (3 + \(\frac{5}{2}\))2 = r2
(- \(\frac{7}{2}\))2 + (\(\frac{11}{2}\))2 = r2
Therefore, equation of circle having centre (\(\frac{7}{2}\), – \(\frac{5}{2}\)) and radius \(\frac{65}{2}\) is
(x – \(\frac{7}{2}\))2 + (y + \(\frac{5}{2}\))2 = \(\frac{65}{2}\)
x2 + \(\frac{49}{4}\) – 7x + y2 + \(\frac{25}{4}\) + 5y = \(\frac{65}{2}\)
x2 + y2 – 7x + 5y – \(\frac{56}{4}\) = 0
x2 + y2 – 7x + 5y – 14 = 0
Alternative Method:

Let the equation of circle be
x2 + y2 + 2gx + 2fy + c = 0 ……………..(i)
Since, it passes through (2, 3).
∴ (2)2 + (3)2 + 2g(2) + 2f(3) + c = 0
4 + 9 + 4g + 6f + c = 0
4g + 6f + c = – 13 ……………….(ii)
Circle also passes through (- 1, 1).
∴ (- 1)2 + (1)2 + 2g(- 1) + 2f(1) + c = 0
⇒ 1 + 1 – 2g + 2f + c = 0
⇒ – 2g + 2f + c = – 2 …………..(iii)
Since, centre of circle lies on line x – 3y – 11 = 0
i.e.,C(- g, – f) lies on line.
∴ – g – 3 (- f) – 11 = 0
– g + 3f = 11 …………..(iv)
On subtracting eq. (iii) from eq. (ii), we get
6g + 4f = – 11 …………….(v)
On solving eqs. (iv) and (y) for g and f, we get
g = – \(\frac{7}{2}\), f = \(\frac{5}{2}\)
On putting the values of g and f in eq. (ii), we get
⇒ – 14 + 15 + c = – 13
⇒ c = – 14
Now, putting the values of g, f and c in eq. (i), we get
x2 + y2 + 2(- \(\frac{7}{2}\)) + 2 (\(\frac{7}{2}\)) – 14 = 0
x2 + y2 – 7x + 5y – 14 = 0.
![]()
Question 12.
Find the equation of the circle with radius 5 whose centre lies on x-axis and passes through the point (2, 3).
Answer.
Let the equation of the required circle be (x – h)2 + (y – k)2 = r2.
Since the radius of the circle is 5 and its centre lies on the x-axis, k = 0 and r = 5.
Now, the equation of the circle becomes (x – h)2 + y2 = 25.
It is given that the circle passes through point (2, 3).
(2 – h)2 + 32 = 25
⇒ (2 – h)2 = 25 – 9
⇒ (2 – h)2 = 16
⇒ 2 – h = ± √l6 = ± 4
If 2 – h = 4, then h = – 2
If 2 – h = – 4, then h = 6
when h = – 2, the equation of the circle becomes
(x + 2)2 + y2 = 25
x2 + 4x + 4 + y2 = 25
x2 + y2 + 4x – 21 = 0.
When h = 6, the equation of the circle becomes
(x – 6)2 + y2 = 25
x2 – 12x + 36 + y2 = 25
x2 + y2 – 12x + 11 = 0.
Question 13.
Find the equation of the circle passing through (0, 0) and making intercepts a and b on the coordinate axes.
Answer.
Let the equation of the required circle be (x- h)2 + (y – k)2 = r2.
Since the circle passes through (0, 0).
(0 – h)2 + (0 – k)2 = r2.
h2 + k2 = r2
The equation of the circle now becomes (x – h)2 + (y – k)2 = h2 + k2.
It is given that the circle makes intercepts a and b on the coordinate axes.
This means that the circle passes thróugh points (a, 0) and (0, b).
(a – h)2 + (0 – k)2 = h2 + k2
(0 – h)2 + (b – k)2 = h2 + k2
From equation (i), we obtain
a2 – 2ah + h2 + k2 = h2 + k2
⇒ a2 – 2ah = 0
⇒ a(a – 2h) = 0
⇒ a = 0 or (a – 2h) = 0
However, a ≠ 0; hence, (a – 2 h) = 0
⇒ h = \(\frac{a}{2}\).
From equation (ii), we obtain
h2 + b2 – 2bk + k2 = h2 + k2
⇒ b2 – 2bk = 0
⇒ b(b – 2k) = 0
⇒ b = 0 or (b – 2k) = 0
However, b ≠ 0; hence, (b – 2k) = 0
⇒ k = \(\frac{b}{2}\).
Thus, the equation of the required circle is .
\(\left(x-\frac{a}{2}\right)^{2}+\left(y-\frac{b}{2}\right)^{2}=\left(\frac{a}{2}\right)^{2}+\left(\frac{b}{2}\right)^{2}\)
⇒ \(\left(\frac{2 x-a}{2}\right)^{2}+\left(\frac{2 y-b}{2}\right)^{2}=\frac{a^{2}+b^{2}}{4}\)
⇒ 4x2 – 4ax + a2 + 4y2 – 4by + b2 = a2 + b2
⇒ 4x2 + 4y2 – 4ax – 4by = 0
⇒ x2 + y2 – ax – by = 0.
![]()
Question 14.
Find the equation of a circle with centre (2, 2) and passes through the point (4, 5).
Answer.
The equation of circle is (x – h)2 + (y – k)2 = r2 ……………..(i)
Since the circle passes through point (4, 5) and co-ordinates of centre are (2, 2)
∴ radius of circle = \(\sqrt{(4-2)^{2}+(5-2)^{2}}=\sqrt{4+9}=\sqrt{13}\)
Now the equation of required circle is
(x – 2)2 + (y – 2)2 = (√13)2
4x2 + 4y2 – 4ax – 4ay = 13
x2 + y2 – 4x – 4y – 5 = 0
Question 15.
Does the point (- 2.5, 3.5) lie inside, outside or on the circle x2 + y2 = 25?
Answer.
The equation of the given circle is x2 + y2 = 25
x2 + y2 = 25
⇒ (x – 0)2 + (y – 0)2 = 52, which is of the form(x – h)2 + (y – k)2 = r2,
where h = 0, k = 0 and r = 5.
centre = (0, 0) and radius = 5.
Distance between point (- 2.5, 3.5) and centre (0, 0)
= \(\sqrt{(-2.5-0)^{2}+(3.5-0)^{2}}=\sqrt{6.25+12.25}=\sqrt{18.5}\)
= 4.3 (approx.) < 5.
Since the distance between point (- 2.5, 3.5) and centre (0, 0) of the circle is less than the radius of the circle, point (- 2.5, 3.5) lies inside the circle.
